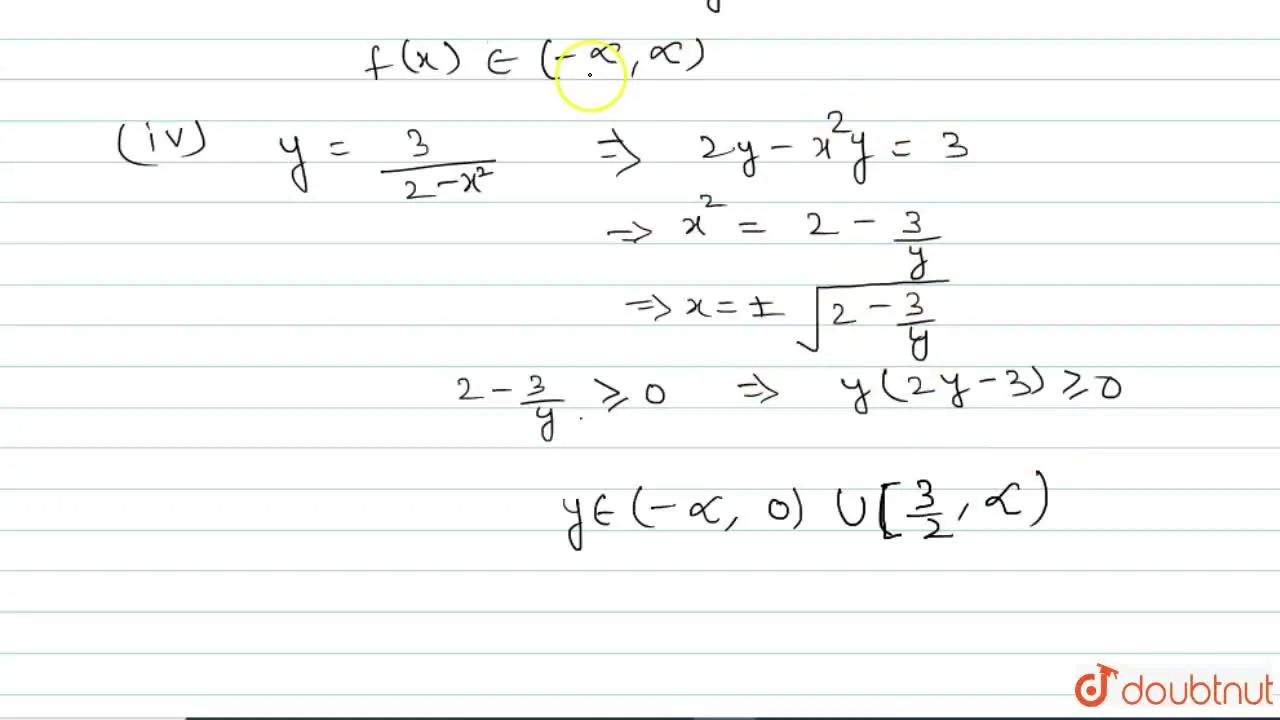Find the range of each of the following functions: f(x)=1√x−5 (ii) f(x)=√6−x2 (iii) f(x)=x1−x2 (iv) f(x)=32−x2
Step by step video & image solution for Find the range of each of the following functions: f(x)=1/(sqrt(x-5)) (ii) f(x)=sqrt(6-x^2) (iii) f(x)=x/(1-x^2) (iv) f(x)=3/(2-x^2) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
Find the range of the following functions.
f(x)=√3x2−4x+5View SolutionFind the domain of each of the following functions: f(x)=√cosx−12√6+35x−6x2
View SolutionFind the domain of each of the following functions: f(x)=1logx(1−x)+√x+2
View SolutionFind the domain of each of the following function: f(x)=2sin−1x+1√x−2
View SolutionFind the range of each of the following function:
f(x)=cos−1((x−1)(x+5)x(x−2)(x−3))View SolutionFind the domain of each of the following functions given by f(x)=1√x−|x| (ii) f(x)=1√x+|x| (iii) f(x)=1√x−[x] (iv) f(x)=1√x+[x]
View SolutionFind the range of each of the following functions: f(x)=|x−3| f(x)=1−|x−2| f(x)=|x−4|x−4
View SolutionFind the range of each of the following function:
f(x)=sin−1(√3x2+15x2+1)View SolutionFind the domain of each of the following functions: f(x)=sin−1√x2−1 (ii) f(x)=sin−1x+sin−12x
View SolutionFind the domain of the following functions: f(x)=√2−x−1√9−x2
View Solution