Two men PandQ start with velocity u at the same time from the junction of two roads inclined at 450 to each other. If they travel by different roads, find the rate at which they are being separated.
To solve the problem step by step, we will follow the reasoning provided in the video transcript and derive the solution systematically.
Step 1: Understand the Setup
Two men, P and Q, start from the same point (junction O) and travel along two roads that are inclined at an angle of 45 degrees to each other. They both have the same initial velocity u.
Step 2: Define the Distances
Let the distance traveled by both men after time t be represented as x. Since they both travel with the same speed, we have:
x=u⋅t
Step 3: Use the Cosine Rule
To find the distance y between the two men after time t, we can apply the cosine rule in triangle OPQ, where O is the junction, P is the position of man P, and Q is the position of man Q.
According to the cosine rule:
y2=x2+x2−2xxcos(45∘)
Since cos(45∘)=1√2, we can substitute this in:
y2=x2+x2−2x2⋅1√2
This simplifies to:
y2=2x2−2x2√2=2x2(1−1√2)
Step 4: Simplify the Expression
Now, we can factor out 2x2:
y2=2x2(1−1√2)
Taking the square root gives:
y=x
⎷2(1−1√2)
Step 5: Substitute for x
Since x=ut, we substitute this into our equation for y:
y=ut
⎷2(1−1√2)
Step 6: Differentiate to Find Rate of Separation
To find the rate at which they are being separated, we differentiate y with respect to t:
dydt=u
⎷2(1−1√2)
Step 7: Final Expression
Now, we simplify the expression under the square root:
1−1√2=√2−1√2
Thus,
⎷2(1−1√2)=√2⋅√2−1√2=√2(√2−1)=√2√2−2
Finally, we have:
dydt=u√2√2−2
Conclusion
The rate at which the two men are being separated is:
dydt=u√2√2−2
Two men, P and Q, start from the same point (junction O) and travel along two roads that are inclined at an angle of 45 degrees to each other. They both have the same initial velocity u.
Let the distance traveled by both men after time t be represented as x. Since they both travel with the same speed, we have:
x=u⋅t
To find the distance y between the two men after time t, we can apply the cosine rule in triangle OPQ, where O is the junction, P is the position of man P, and Q is the position of man Q.
y2=x2+x2−2xxcos(45∘)
Since cos(45∘)=1√2, we can substitute this in:
y2=x2+x2−2x2⋅1√2
This simplifies to:
y2=2x2−2x2√2=2x2(1−1√2)
Now, we can factor out 2x2:
y2=2x2(1−1√2)
Taking the square root gives:
y=x
⎷2(1−1√2)
Since x=ut, we substitute this into our equation for y:
y=ut
⎷2(1−1√2)
To find the rate at which they are being separated, we differentiate y with respect to t:
dydt=u
⎷2(1−1√2)
Now, we simplify the expression under the square root:
1−1√2=√2−1√2
Thus,
⎷2(1−1√2)=√2⋅√2−1√2=√2(√2−1)=√2√2−2
Finally, we have:
dydt=u√2√2−2
The rate at which the two men are being separated is:
dydt=u√2√2−2
Topper's Solved these Questions
Similar Questions
Two men A and B start with velocities v at the same time from the junction of two roads inclined at 45∘ to each other.If they travel by different roads,find the rate at which they are being separated.
View SolutionTwo cyclists start from the junction of two perpendicular roads, there velocities being 3um/m∈ and 4um/m∈ , respectively. Find the rate at which the two cyclists separate.
View SolutionTwo cyclists start from the junction of two perpendicular roads, there velocities being 3um/m∈ and 4um/m∈ , respectively. Find the rate at which the two cyclists separate.
View SolutionThe distance between two particles is decreasing at the rate of 6 m/sec,when they travel in opposite direction to each other. If these particles travel with same speeds and in the same direction, then the separation increase at the rate of 4 m/sec. The particles have speed as
View SolutionPlaces A and B are 100 km apart on a highway. One car starts from A and another from b at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars
View SolutionPlaces A and B are 100 km apart on a highway. One car starts from A and another from b at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars
View SolutionTwo ships leave port at the same time. One goes 24 km per hour in the direction N 450E and other travels 32 km per hour in the direction S750E. Find the distance between the ships at the end of 3 hours.
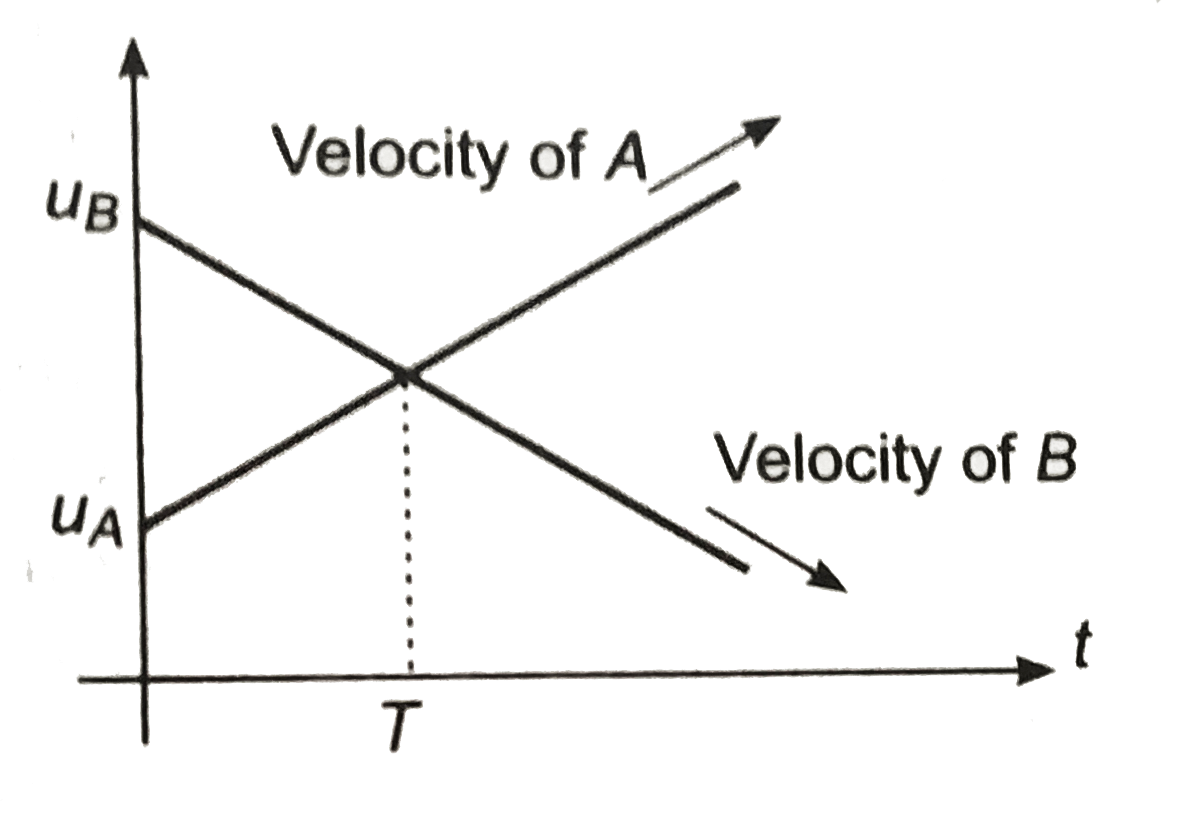
View SolutionA situation is shown in which two objects A and B start their motion from same point in same direction. The graph of their velocities against time is drawn. uAanduB are the initial velocities of A and B respectively. T is the time at which their velocities become equal after start of motion. You cannot use the data of one question while solving another question of the same set. So all the questions are independent of each other.
5. If the value of T is 4 s, then the time after which A will meet B is
View SolutionTwo particles P1 and P2 are performing SHM along the same line about the same mean position , initial they are at their extreme position. If the time period of each particle is 12 sec and the difference of their amplitude is 12cm then find the minimum time after which the separation between the particle becomes 6cm.
View SolutionThrough a rectangular field of sides 90 m × 60 m , two roads are constructed which are parallel to the sides and cut each other at right angles through the centre of the field. If the width of the roads is 3 m, find the total area covered by the two roads.
View Solution
