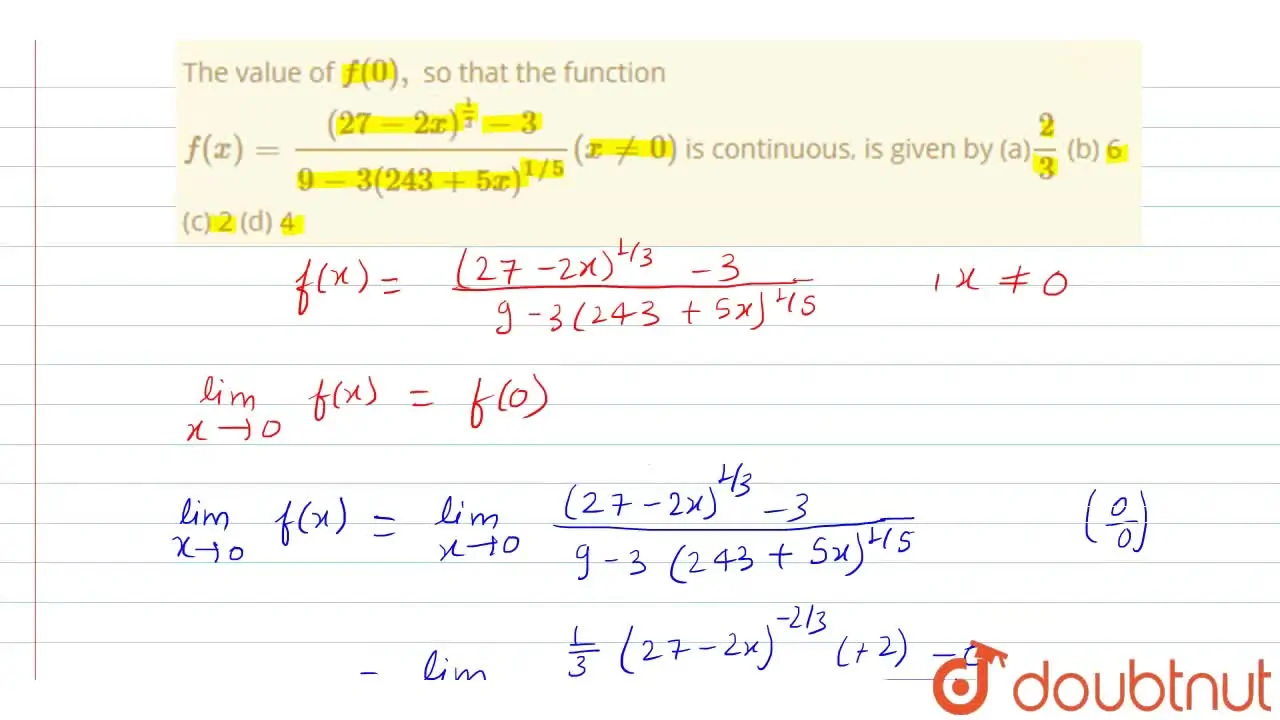
The value of so that the function is continuous, is given by (a) (b) 6 (c) 2 (d) 4
option c is correct
Related Playlists
Similar Questions
The value of f(0) so that the function f(x)=2−(256−7x)18(5x+32)1/5−2,x≠0 is continuous everywhere, is given by
View SolutionThe value of f(0) so that the function f(x)=2−(256−7x)18(5x+32)1/5−2,x≠0 is continuous everywhere, is given by −1 (b) 1 (c) 26 (d) none of these
View SolutionFind the value of f(1) that the function f(x)=9(x23−2x13+1)(x−1)2,x≠1 is continuous at x =1
View SolutionThe value of f(0) such that the function f(x)=3√1+2x−4√1+xx is continuous at x = 0, is
View SolutionThe value of f(0), so that the function f(x)=√a2−ax+x2−√a2+ax+x2√a+x−√a−x becomes continuous for all x, given by a32 (b) a12 (c) −a12 (d) −a32
View SolutionThe value of f(0), so that the function f(x)=√a2−ax+x2−√a2+ax+x2√a+x−√a−x becomes continuous for all x, given by a32 (b) a12 (c) −a12 (d) −a32
View SolutionThe value of a for which the function f(x)=f(x)={(4x−1)ˆ3sin(xa)log{(1+x23)},x≠012(log4)3,x=0 may be continuous at x=0 is 1 (b) 2 (c) 3 (d) none of these
View SolutionIf the function f(x)=2x−sin−1x2x+tan−1x is continuous at each point of its domain, then the value of f(0) (a) 2 (b) 13 (c) −13 (d) 23
View SolutionFind the value of f(0) so that the function given below is continuous at x=0 f(x)=1−cos2x2x2
View SolutionIf the function f(x)=2x−sin−1x2x+tan−1x is continuous at each point of its domain, then the value of f(0) (A) 43 (B) 13 (C) −13 (D) 23
View Solution