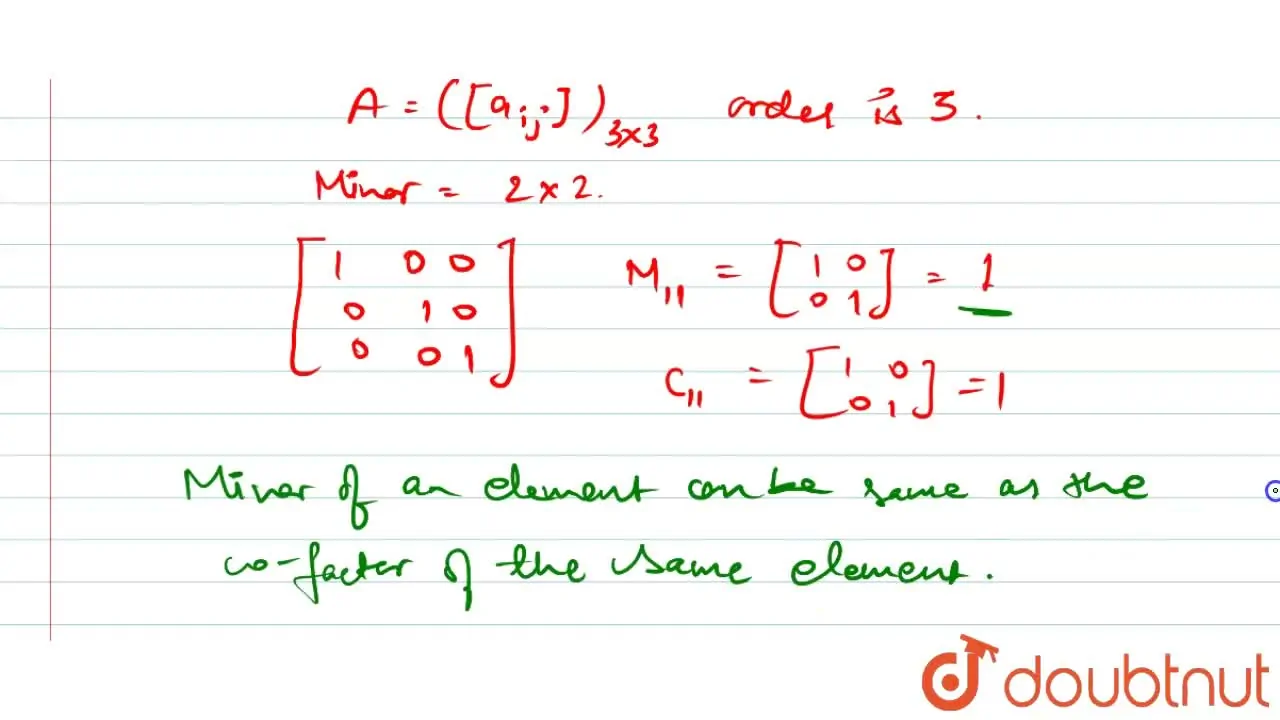
Which of the following is not correct in a given determinant of A,
where A=([aij])3x3
A. Order of minor is less than order of the det(A)
B.
Minor of an element can never be equal to cofactor of the same element
C. Value of a determinant is obtained by multiplying elements of a row or
column by corresponding cofactors
D. Order of minors and cofactors of elements of A is same
A=[aij]3×3
minor=2×2
⎡⎢⎣100010001⎤⎥⎦
c11=1
minor can be equal to cofactor
Related Playlists
Similar Questions
Find minors and cofactors of all the elements of the determinant ∣∣∣1−243∣∣∣
View SolutionIf A is square matrix of order 3, then which of the following is not true ? a.| A'|=|A|b.|kA∣∣=k3∣∣A∣∣ c. minor of an element of |A| can never be equal cofactor of the same element d. order of minors and of cofactors of elements of |A| is same
View SolutionThe sum of the products of the elements of any row of a matrix A with the corresponding cofactors of the elements of the same row is always equal to
View SolutionWhich is correct increasing order of their tendency of the given elements to from M3− ion?
View SolutionWrite the minor and cofactor of each element of the following determinants and also evaluate the determinant in each case: ∣∣∣5−1003∣∣∣
View SolutionElementary Transformation of a matrix:
The following operation on a matrix are called elementary operations (transformations)
1. The interchange of any two rows (or columns)
2. The multiplication of the elements of any row (or column) by any nonzero number
3. The addition to the elements of any row (or column) the corresponding elements of any other row (or column) multiplied by any number
Echelon Form of matrix : A matrix A is said to be in echelon form if
(i) every row of A which has all its elements 0, occurs below row, which has a non-zero elements
(ii) the first non-zero element in each non –zero row is 1.
(iii) The number of zeros before the first non zero elements in a row is less than the number of such zeros in the next now.
[ A row of a matrix is said to be a zero row if all its elements are zero]
Note: Rank of a matrix does not change by application of any elementary operations
For example ⎡⎢⎣113012000⎤⎥⎦,⎡⎢⎣113601220000⎤⎥⎦ are echelon forms
The number of non-zero rows in the echelon form of a matrix is defined as its RANK. For example we can reduce the matrix A=⎡⎢⎣1232473610⎤⎥⎦ into echelon form using following elementary row transformation.
(i)R2→R2−2R1 and R3→R3−3R1⎡⎢⎣123001001⎤⎥⎦
(ii)R2→R2−2R1⎡⎢⎣123001000⎤⎥⎦
This is the echelon form of matrix A Number of nonzero rows in the echelon form =2 ⇒ Rank of the matrix A is 2
The echelon form of the matrix ⎡⎢⎣1343391291341⎤⎥⎦ is :View SolutionElementary Transformation of a matrix:
The following operation on a matrix are called elementary operations (transformations)
1. The interchange of any two rows (or columns)
2. The multiplication of the elements of any row (or column) by any nonzero number
3. The addition to the elements of any row (or column) the corresponding elements of any other row (or column) multiplied by any number
Echelon Form of matrix : A matrix A is said to be in echelon form if
(i) every row of A which has all its elements 0, occurs below row, which has a non-zero elements
(ii) the first non-zero element in each non –zero row is 1.
(iii) The number of zeros before the first non zero elements in a row is less than the number of such zeros in the next now.
[ A row of a matrix is said to be a zero row if all its elements are zero]
Note: Rank of a matrix does not change by application of any elementary operations
For example ⎡⎢⎣113012000⎤⎥⎦,⎡⎢⎣113601220000⎤⎥⎦ are echelon forms
The number of non-zero rows in the echelon form of a matrix is defined as its RANK. For example we can reduce the matrix A=⎡⎢⎣1232473610⎤⎥⎦ into echelon form using following elementary row transformation.
(i)R2→R2−2R1 and R3→R3−3R1⎡⎢⎣123001001⎤⎥⎦
(ii)R2→R2−2R1⎡⎢⎣123001000⎤⎥⎦
This is the echelon form of matrix A Number of nonzero rows in the echelon form =2 ⇒ Rank of the matrix A is 2
Rank of the matrix ⎡⎢⎣1111−1−1311⎤⎥⎦ is :View SolutionElementary Transformation of a matrix:
The following operation on a matrix are called elementary operations (transformations)
1. The interchange of any two rows (or columns)
2. The multiplication of the elements of any row (or column) by any nonzero number
3. The addition to the elements of any row (or column) the corresponding elements of any other row (or column) multiplied by any number
Echelon Form of matrix : A matrix A is said to be in echelon form if
(i) every row of A which has all its elements 0, occurs below row, which has a non-zero elements
(ii) the first non-zero element in each non –zero row is 1.
(iii) The number of zeros before the first non zero elements in a row is less than the number of such zeros in the next now.
[ A row of a matrix is said to be a zero row if all its elements are zero]
Note: Rank of a matrix does not change by application of any elementary operations
For example ⎡⎢⎣113012000⎤⎥⎦,⎡⎢⎣113601220000⎤⎥⎦ are echelon forms
The number of non-zero rows in the echelon form of a matrix is defined as its RANK. For example we can reduce the matrix A=⎡⎢⎣1232473610⎤⎥⎦ into echelon form using following elementary row transformation.
(i)R2→R2−2R1 and R3→R3−3R1⎡⎢⎣123001001⎤⎥⎦
(ii)R2→R2−2R1⎡⎢⎣123001000⎤⎥⎦
This is the echelon form of matrix A Number of nonzero rows in the echelon form =2 ⇒ Rank of the matrix A is 2
Rank of the matrix ⎡⎢⎣111−112443452⎤⎥⎦ is :View SolutionWrite Minors and Cofactors of the elements of following determinants:
(i) ∣∣∣2−403∣∣∣ (ii) ∣∣∣acbd∣∣∣View SolutionFind the minors and cofactors of elements of the matrix A=[aij]=⎡⎢⎣13−24−56352⎤⎥⎦
View Solution