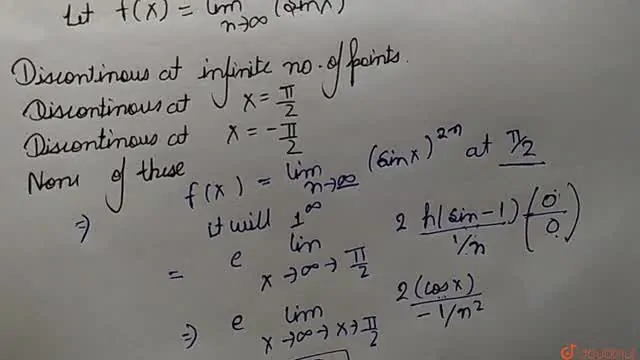Let f(x)=limn→∞(sinx)2n
A
Discontinuous at infinite number of points
B
discontinuous at x=π2
C
Discontinuous at x=−π2
D
none of these
Video Solution
Text Solution
The correct Answer is:D
|
Answer
Step by step video & image solution for Let f(x)= lim_(n->oo)(sinx)^(2n) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023
Similar Questions
Let f(x)=limn→∞log(2+x)−x2nsinx1+x2n. then
View Solutionlimn→∞sin(x2n)x2n
View Solutionlimn→∞nsin(1n)
View Solutionlimn→∞2n−1sin(a2n)
View SolutionLet f(x)=limn→∞(2sinx)2n3n−(2cosx)2n,n∈Z. Then
View SolutionLet f(x)=limn→∞xx2n+1. Then f has
View Solutionlimx→∞[sinxx]
View Solutionlimx→∞sinxx=
View SolutionLet f:(−π2,π2)→R, f(x)={limn→∞(tanx)2n+x2sin2x+(tanx)2nx∈0,n∈N; 1 if x=0 which of the following holds good? (a) f(−π−4)=f(π+4) (b) f(−π−4)=f(−π+4) (c) f(π−4)=f(π+4) (d) f(0+)=f(0)=f(0−)
View SolutionDiscuss the continuity of f(x)∈[0,2],wheref(x)=(lim)n→∞(sin(πx2))2n
View Solution