Real part of (ee)ιθ is
To find the real part of (ee)iθ, we can follow these steps:
Step 1: Define the expression
Let Z=(ee)iθ.
Step 2: Rewrite using exponential properties
Using the property of exponents, we can rewrite Z:
Z=ee⋅iθ
Step 3: Apply Euler's formula
According to Euler's formula, eiθ=cos(θ)+isin(θ). Therefore, we can express Z as:
Z=ee⋅iθ=e0⋅eiθ=e0⋅(cos(θ)+isin(θ))=cos(θ)+isin(θ)
Step 4: Identify the real part
Now, we need to find the real part of Z. The real part of Z is simply the coefficient of the real component:
Real part of Z=Re(Z)=eRe(e⋅iθ)⋅cos(Im(e⋅iθ))
Here, Re(e⋅iθ)=0 and Im(e⋅iθ)=e⋅θ.
Thus, we can simplify:
Real part of Z=e0⋅cos(e⋅θ)=1⋅cos(e⋅θ)=cos(e⋅θ)
Final Answer
The real part of (ee)iθ is:
Real part=cos(e⋅θ)
---
Let Z=(ee)iθ.
Using the property of exponents, we can rewrite Z:
Z=ee⋅iθ
According to Euler's formula, eiθ=cos(θ)+isin(θ). Therefore, we can express Z as:
Z=ee⋅iθ=e0⋅eiθ=e0⋅(cos(θ)+isin(θ))=cos(θ)+isin(θ)
Now, we need to find the real part of Z. The real part of Z is simply the coefficient of the real component:
Real part of Z=Re(Z)=eRe(e⋅iθ)⋅cos(Im(e⋅iθ))
Here, Re(e⋅iθ)=0 and Im(e⋅iθ)=e⋅θ.
Real part of Z=e0⋅cos(e⋅θ)=1⋅cos(e⋅θ)=cos(e⋅θ)
The real part of (ee)iθ is:
Real part=cos(e⋅θ)
Topper's Solved these Questions
- COMPLEX NUMBERSBOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE3.6
10 Videos
- COMPLEX NUMBERSBOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE3.7
6 Videos
- COMPLEX NUMBERSBOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERSEXERCISE - EXERCISE3.4
7 Videos
- CIRCLESBOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
- CONIC SECTIONSBOOK - CENGAGE ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - All Questions
101 Videos
Similar Questions
Find the real part of ee^(iθ)
View SolutionIf the imaginery part of z−3eiθ+eiθz−3 is zero, then z can lie on
View SolutionBetween any two real roots of the equation exsinx−1=0 the equation excosx+1=0 has
View Solutionlimx→0{(1+x)2x} (where {.} denotes the fractional part of x (a) e2−7 (b) e2−8 (c) e2−6 (d) none of these
View SolutionFind number of real roots of equation e4x+e3x−4e2x+ex+1=0 is
View SolutionStatement-1: The equation πex−e+eπx−π+ππ+eex−π−e=0 has real roots.
Statement-2: If f(x) is a polynomial and a, b are two real numbers such that f(a)f(b)<0, then f(x) = 0 has an odd number of real roots between a and b.
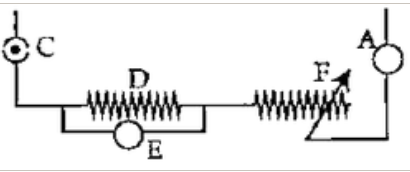
View SolutionIn the electric circuit shown in Fig., label the parts A, B, C, D, E, and E
State the function of each part. Show in the diagram the direction of flow of current.
View SolutionThe number of real root of the equation ex−1+x−2=0, is
View SolutionNumber of real roots of the equation ex−1−x=0 is
View SolutionThe number of real solutions of the equation sin(ex)=2x+2−x is
View Solution
