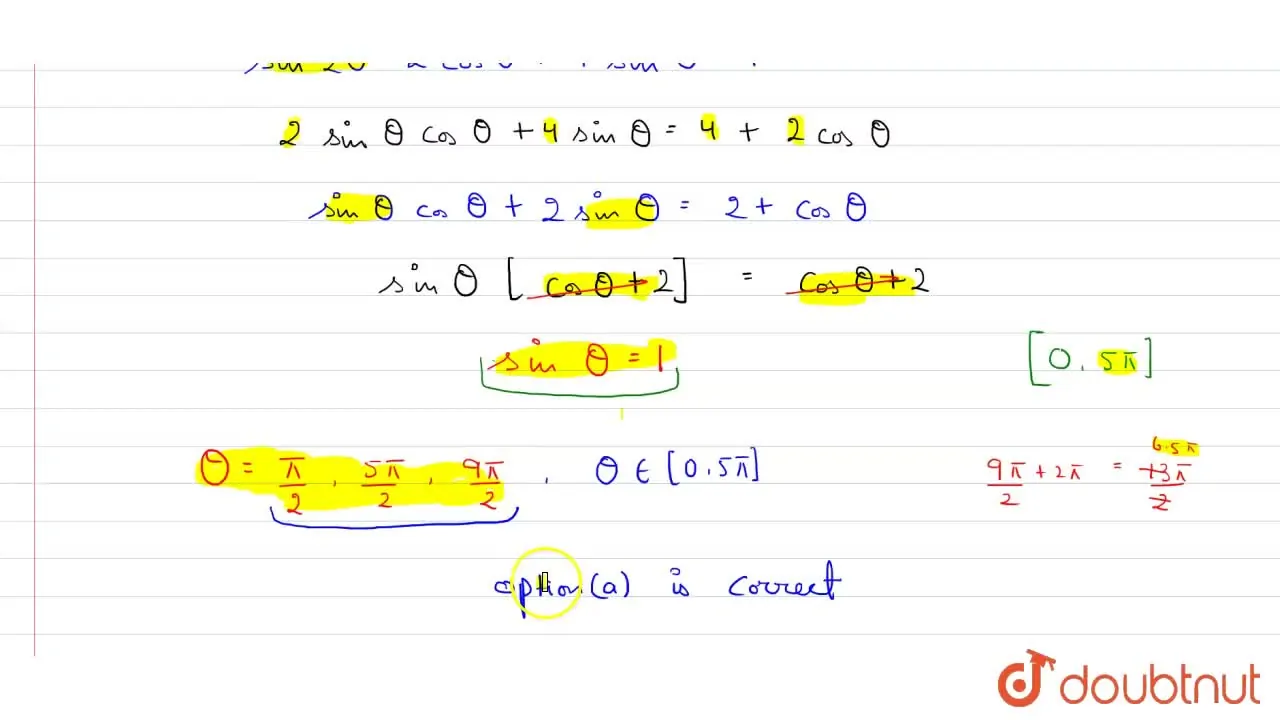
The number of solutions of the equation sin2θ−2cosθ+4sinθ=4 in [0,5π] is equal to
3
4
5
6
The correct Answer is:A
sin2θ−2cosθ+4sinθ=4
⇒(2sinθcosθ+4sinθ)=(2cosθ+4)
⇒sinθ(cosθ+2)=(cosθ+2)
∴sinθ=1
⇒θ=π2,5π2,9π2
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Exercises (Multiple correct type)31videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Exercises (Linked comprehension type)20videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Concept Application Exercise 4.96videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - ARCHIVES INTEGER TYPE1videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - SINGLE CORRECT ANSWER TYPE38videos
TRIGONOMETRIC FUNCTIONS
Similar Questions
The number of solution of the equation sinθcosθcos2θ.cos4θ=12 in the interval [0,π]
View SolutionThe number of solution satisfying the equations tan4θ=cot5θ and sin2θ=cosθ in [0,2π] is
View SolutionNumber of solutions of the equation sin2θ+2=4sinθ+cosθ lying in the interval [π,5π] is
View SolutionThe solution of the equation cos2θ−2cosθ=4sinθ−sin2θ where θ∈[0,π] is
View SolutionThe number of solutions of the pair of equations 2sin2θ−cos(2θ)=0,2cos2θ−3sinθ=0 in the interval [0,2π] is
View SolutionThe number of solutions of cot(5πsinθ)=tan(5πcosθ),∀θ∈(0,2π) is
View SolutionThe number of solutions of the equation log5tanθ=log54.log4(3sinθ)in[0,8π] is
View SolutionThe number of solutions of the pair of equations 2sin2θ−cos2θ=0 2cos2θ−3sinθ=0 in the interval [0,2π] is
View SolutionNumber of solution (s) of equation sinθ=sec24θ in [0,π] is/are :
View SolutionThe number of solutions of the equation cos7θ+sin4θ=1 in the interval (−π,π), is
View Solution