If cospθ+cosqθ=0, then prove that the different values of θ are in A.P. with common difference 2π/(p±q).
Video Solution
Text Solution
cospθ=−cosqθ=cos(π−qθ)
⇒pθ=2nπ−+(π−qθ)
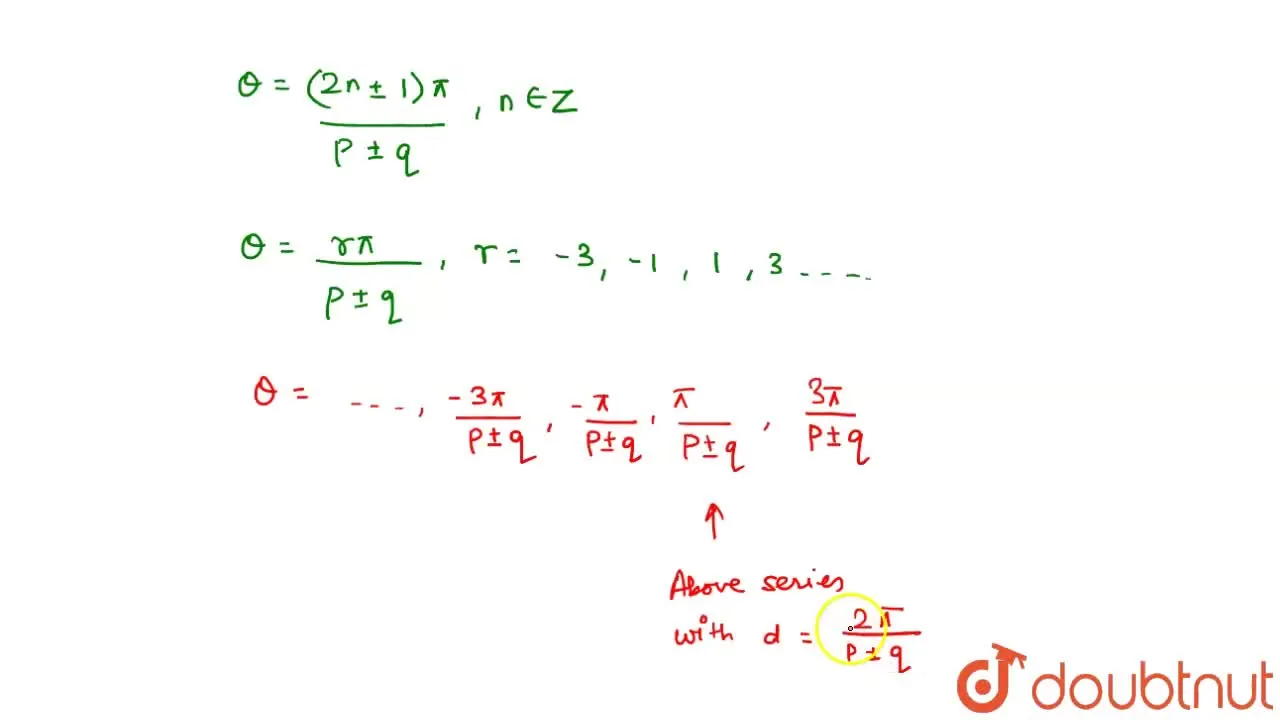
⇒(p−+q)θ=(2n−+1)π
⇒θ=(2n−+1)π(p±q),n∈Z
⇒θ=rπp−+q, where r=−3,−1,1,3....
⇒θ=....,−3πp−+q,−πp−+q,πp−+q,3πp−+q,....
The above series is an A.P., of common difference =2πp−+q
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Concept Application Exercise 4.49videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Concept Application Exercise 4.55videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Concept Application Exercise 4.26videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - ARCHIVES INTEGER TYPE1videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - SINGLE CORRECT ANSWER TYPE38videos
TRIGONOMETRIC FUNCTIONS
Similar Questions
If tanaθ−tanbθ=0, then prove that the values of θ forms an A.P.
View SolutionIf tan mθ=tannθ and general value of θ are in AP, then common difference is
View SolutionSolve : cospθ=sinqθ.
View SolutionIf cosecθ+cotθ=p, then prove that the cosθ=p2−1p2+1
View SolutionThe common difference of the A.P. is :
View SolutionIf cosec theta + cot theta = p prove that cos theta = p2-1 / p2 + 1
View SolutionIf sin(πcosθ)=cos(πsinθ), then the value of cos(θ±π4) is
View SolutionIf sinθ,1,cos2θ are in G.P., then find the general values of θ
View SolutionIf ∣∣ ∣∣cosθ−11cos2θ43277∣∣ ∣∣=0, then the number of values of θ in [0,1π] is
View SolutionThe values of x between 0 and 2π which satisfy the equation sinx√8cos2x=1 are in A.P. with common difference is
View Solution