Prove that (1+sec2θ)(1+sec4θ)(1+sec8θ)=Tan8θtanθ
Video Solution
Text Solution
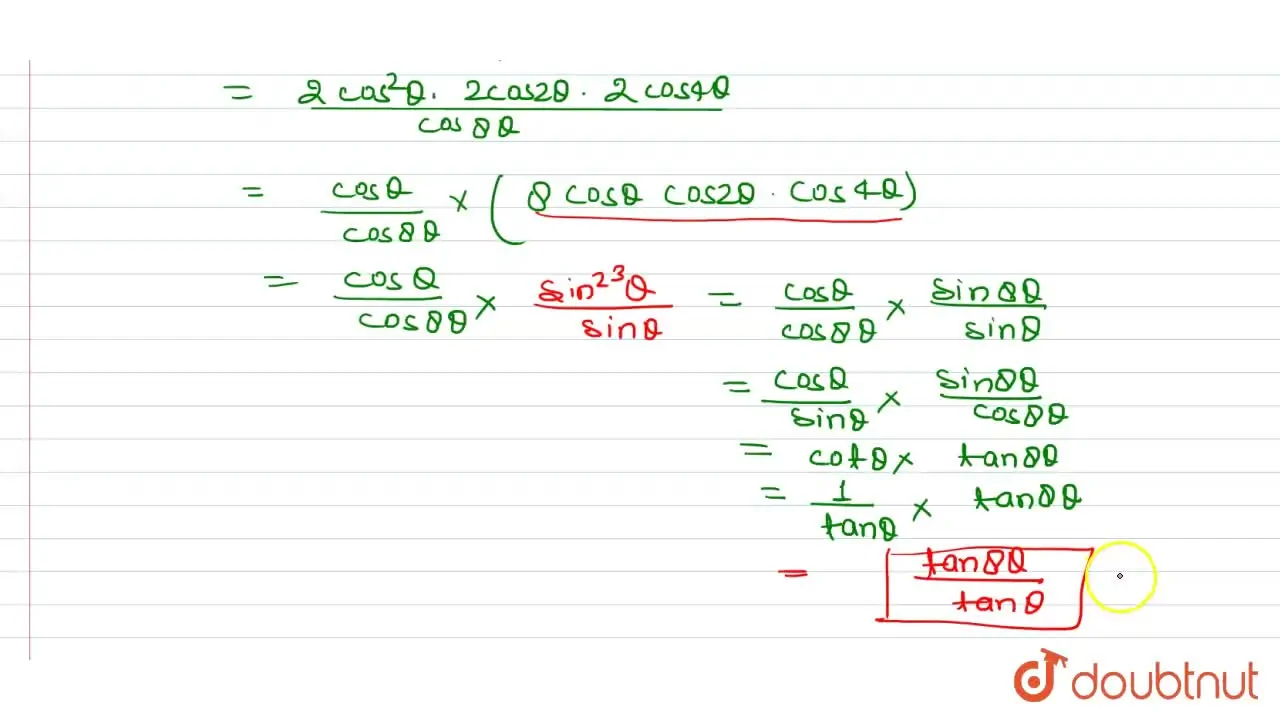
RHS=1+cos2θcos2θ×1+cos4θcos4θ×1+cos8θcos8θ
=2cos2θ×2cos22θ×2cos24θcos2θcos4θcos8θ
=[8cosθcos2θcos4θ]cosθcos8θ=[sin8θsinθ]cosθcos8θ=tan8θtanθ
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Concept App. 3.56videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Concept App. 3.65videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Concept App. 3.313videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - SINGLE CORRECT ANSWER TYPE38videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC RATIOS FOR COMPOUND, MULTIPLE, SUB-MULTIPLE ANGLES, AND TRANSFORMATION FORMULAS EXERCISE - Multiple Correct Answers Type6videos
TRIGONOMETRIC RATIOS FOR COMPOUND, MULTIPLE, SUB-MULTIPLE ANGLES, AND TRANSFORMATION FORMULAS
Similar Questions
Prove that: (1+sec2θ)(1+sec4θ)(1+sec8θ)....(1+sec2nθ)=tan2nθcotθ,n∈N.
View SolutionProve that: (1+sec2θ)(1+sec4θ)(1+sec8θ)...(1+sec2nθ)=tan2nθcotθ,n∈N.
View SolutionProve that:sec8θ−1sec4θ−1=tan8θtan2θ
View SolutionProve that:sec8θ−1sec4θ−1=tan8θtan2θ
View SolutionProve that : sec8θ−1sec4θ−1=tan8θtan2θ
View SolutionProve that secθ+tanθ−1tanθ−secθ+1=cosθ1−sinθ
View SolutionProve that
sec2θ−1tan2θ=1View SolutionProve that
(sinθ−cosecθ)(cosθ−secθ)=1tanθ+cotθ.View SolutionSolve 4cosθ−3 sec θ=tanθ.
View Solutionprove that : tan2nθtanθ=(1+sec2θ)(1+sec22θ)(1+sec23θ)…(1+sec2nθ)
View Solution