Question

let $A(x_1,0)$ and $B(x_2,0)$ be the foci of the hyperbola $\frac{x^2}{9}-\frac{y^2}{16}=1$ suppose parabola having vertex at origin and focus at $B$ intersect the hyperbola at $P$ in first quadrant and at point Q in fourth quadrant.

Options

A. `(-(9)/(10),0)`
B. `((2)/(3),0)`
C. `((9)/(10),0)`
D. `((2)/(3),sqrt(6))`

Explanation

`a=3,e=(1)/(3)`
`:. F_(1)-=(-1,0) and F_(2)-=(1,0)`
SO, equation of parbola is `y^(2)=4x`

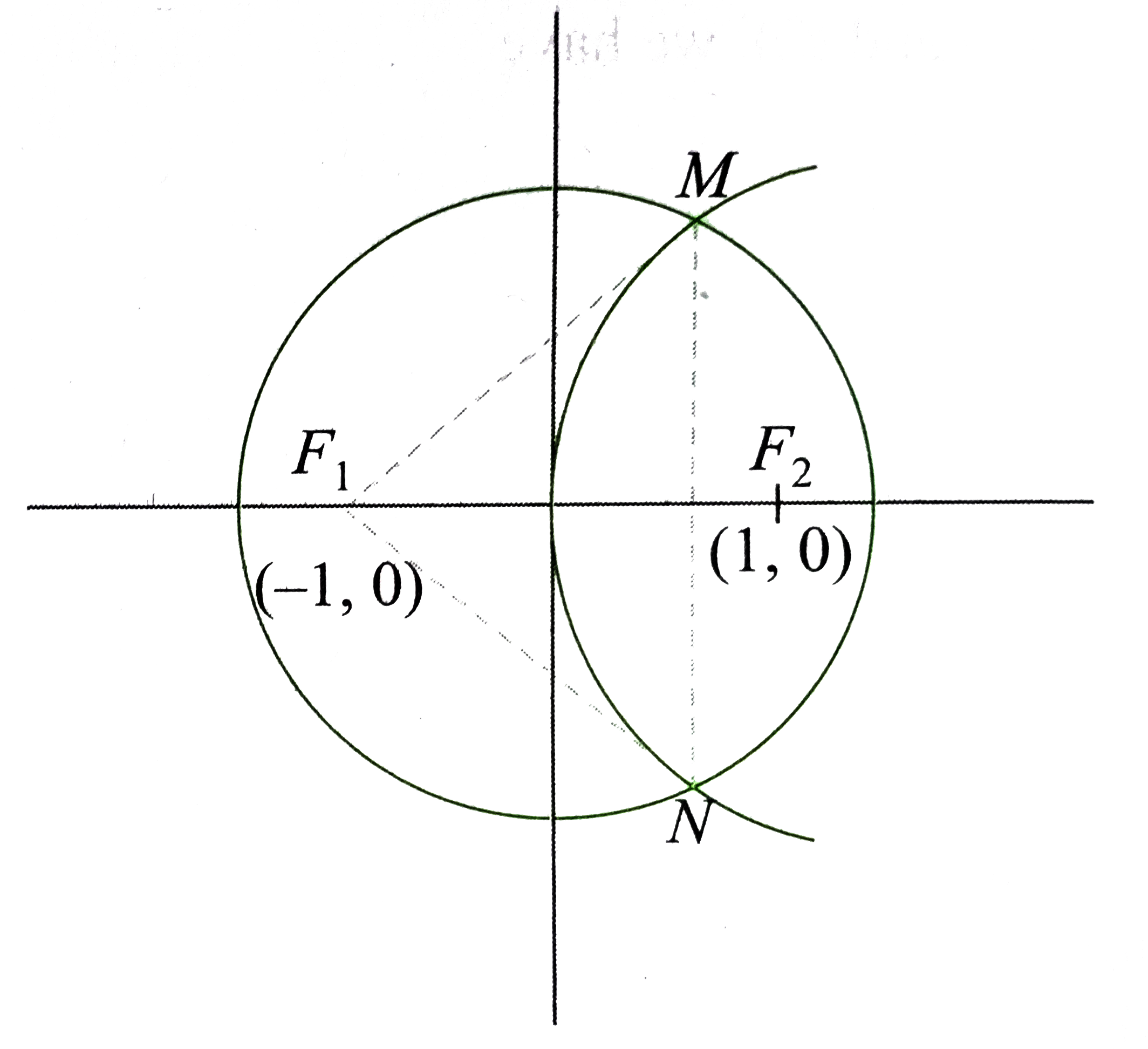
Solving ellipse and parabola, we get points of intersection as
`M((3)/(2),sqrt(6)) 1 and M((3)/(2),sqrt(6))`
Orthocentre of `DeltaF_(1)`MN lioes onb x-axis.
Slope of
`F_(1)N=(-sqrt(6)-0)/((3)/(2)+1)=(-2sqrt(6))/(5)`
Putting y=0 or orhtocentre, we get `y=-(9)/(10)`
So, orthocenter is `(-(9)/(10),0)`