Question

If the normal at $P\left(2\frac{3\sqrt{3}}{2}\right)$
meets the
major axis of ellipse $\frac{x^2}{16}+\frac{y^2}{9}=1$
at $Q$
, and $S$
and $S\text{'}$
are the
foci of the given ellipse, then find the ratio $SQ:S^{\prime }Q\stackrel{.}{}$

Explanation

Equatio of ellipse `P(2,(3sqrt(3))/(2))`
`:. e^(2)=1-(9)/(16)+(7)/(16)`
`rArre=(sqrt(7))/(4)`
Hence , foci are `S(sqrt7,0) and S(-sqrt(7))`

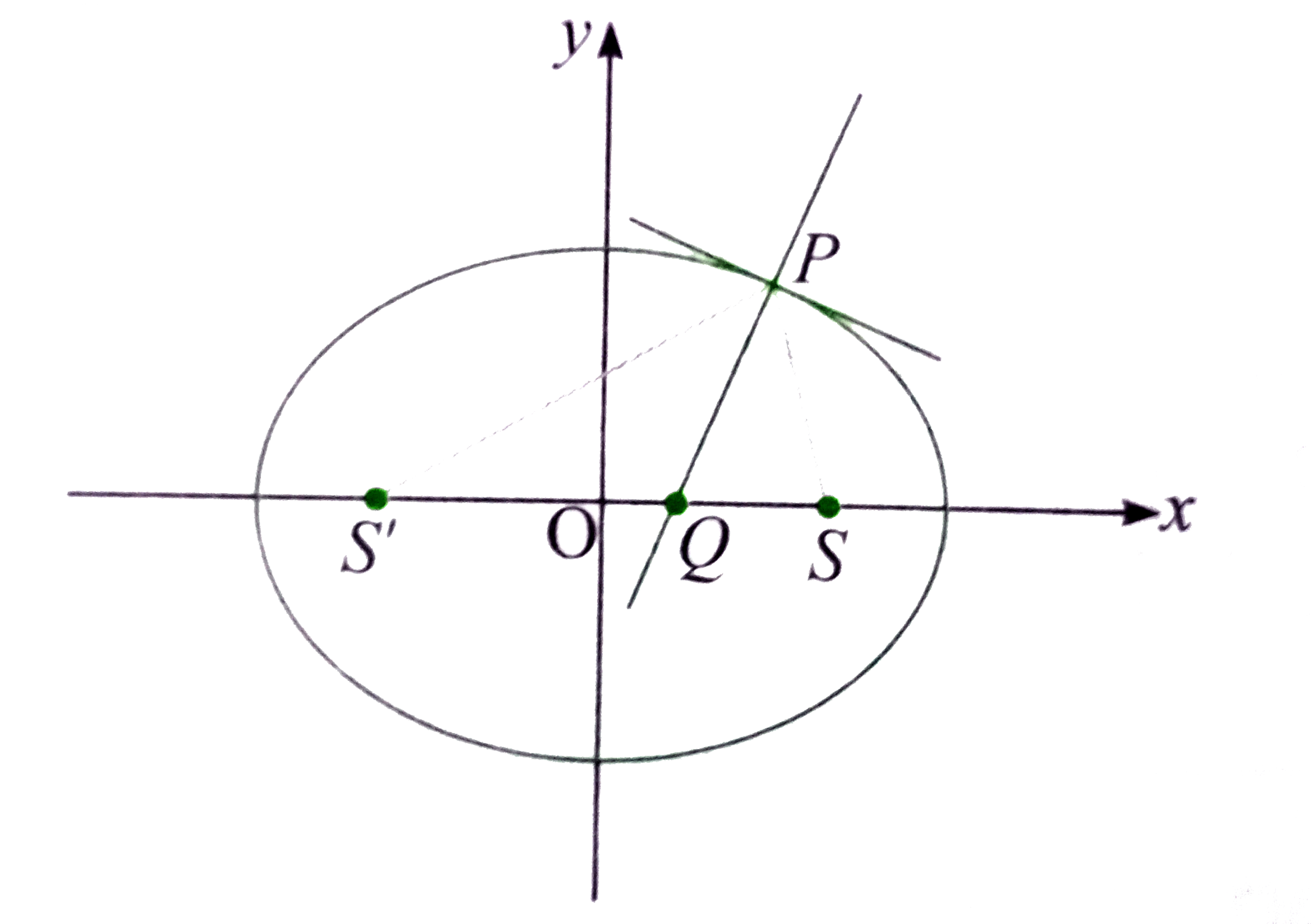
Normal at P bisects the angle `angleSPS''`
Therefore, in triangle SPS' using bisector theorem, we get `(SQ)/(S'Q)=(SP)/(S'P)=(a-ex)/(a+ex)=(4-(sqrt(7))/(4)xx2)/(4+(sqrt(7))/(4)xx2)=(8-sqrt(7))/(8-sqrt(7))`