Find the locus of the midpoint of the chord of the circle x2+y2−2x−2y=0 , which makes an angle of 1200 at the center.
The correct Answer is:(x−1)2+(y−1)2=1
Given circles is (x−1)2+(y−1)2=4.
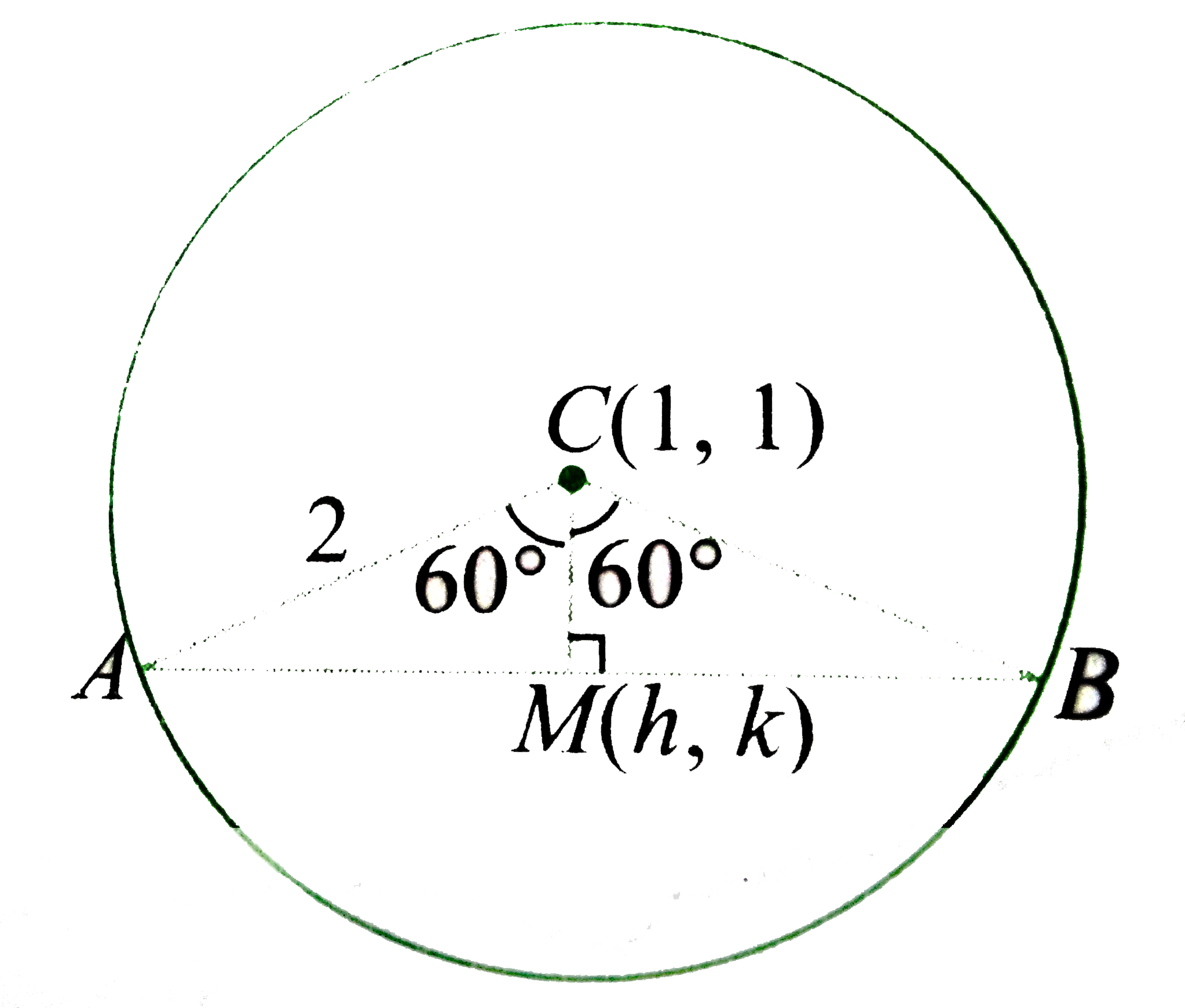
In the figure, chord AB subtends an angle of 120∘ at the centre . M (h,k) is the midpoint of chord AB.
In right angled triangle AMC,
CM=ACcos60∘
√(h−1)2+(k−1)2=2×12
Therefore, required locus is (x−1)2+(y−1)2=1
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.20
1 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - Excercises (Single Correct Answer Type)
109 Videos
- CIRCLE BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.18
1 Videos
- BINOMIAL THEOREMBOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix
4 Videos
- CIRCLESBOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type
8 Videos
Similar Questions
The locus of the midpoint of the chord of the circle x2+y2−2x−2y−2=0 which makes an angle of 120∘ at the centre is
View SolutionFind the locus of the mid-point of the chords of the circle x2+y2+2gx+2fy+c=0 which subtend an angle of 1200 at the centre of the circle.
View SolutionThe locus of the mid-points of the chords of the circle x2+y2−2x−4y−11=0 which subtends an angle of 60∘ at center is
View SolutionFind the locus of the midpoint of the chords of the circle x2+y2−ax−by=0 which subtend a right angle at the point (a2,b2). is
View SolutionFind the locus of the midpoint of normal chord of parabola y2=4ax
View SolutionFind the locus of the midpoint of the chords of the circle x2+y2=a2 which subtend a right angle at the point (0,0).
View SolutionFind the locus of the midpoint of the chords of the circle x2+y2=a2 which subtend a right angle at the point (c,0).
View SolutionFind the locus of the midpoint of the chords of circle x2+y2=a2 having fixed length l.
View SolutionFind the equation of the locus of the middle points of the chords of the hyperbola 2x2−3y2=1, each of which makes an angle of 450 with the x-axis.
View SolutionFind the locus of the midpoint of normal chord of parabola y2=4ax.
View Solution
