Find the number of common tangents of the circles x2+y2−2x−6y+9=0 and x2+y2+6x−2y+1=0
Here, for the first circle, the center is C1(−3,1) and radius r1 is 1.
For the second circle, the center is C2(−3,1) and radius r2 is 3.
Thus, C1C2=2√5 and r1+r2=4 or C_(1)C_(2) gt r_(1)+r_(2),r_(1) cancel(=)r_(2). Thus,we have four common tangents.
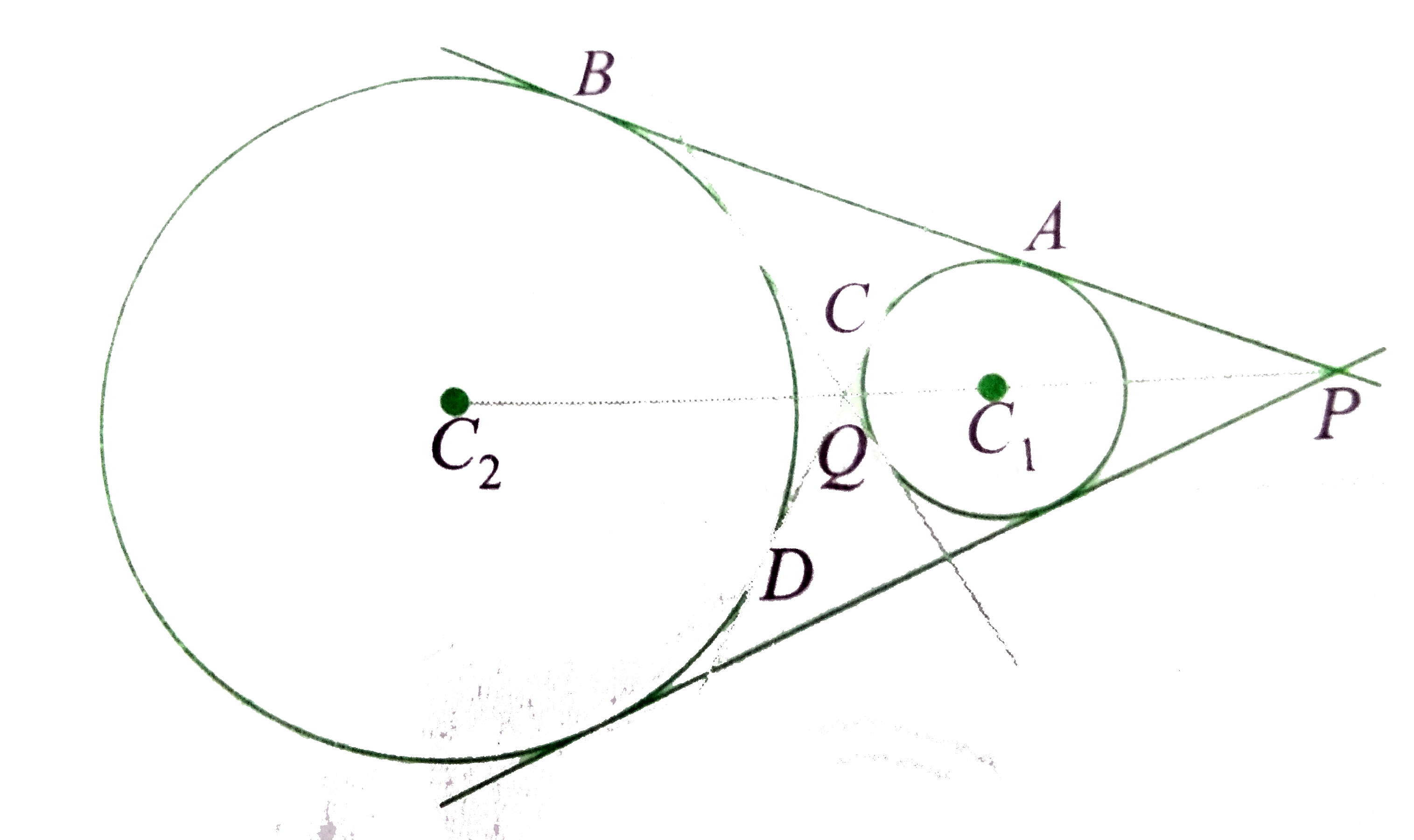
To find direct common tangents :
The coordinates of the point P dividing line C1C2 in the ratio r1:r2,i.e.,1:3, externally, are
(1×(−3)−3×11−3,1×1−3×31−3) or (3,4)
Therefore, the equation of any line through point P(3,4) is
y−4=m1(x−3)
or m1x−y+4−3m1=0 (1)
If (1) is tangent to the first circle, then the length of perpendicular from the center C1(1,3) of (1) is equal to r1 (radius) . Therefore, |m1−3+4−3m1|√(m21+1)=1 or (1−2m1)2=m21+1
or3m21−4m1=0
or m1=0,43
Substituting m1=0 and 4/3 in (1), the equations of direct common tangents are y=4 and 4x−3y=0.
To find transverse common tangents :
The coordinates of the point Q dividing the line C1C2 in the ratio r1:r2,i.e.,1:3, internally , are (0,5/2).
Therefore, the equation of any line through Q(0,5/2) is
y−52=m2(x−0) or m2x−y+52=0 (2)
If (2) is tanent to the first circle, then the length of perpendicular from the center C1(1,3) on (2) is equal to r1 (radius) . Therefore, |m2−3+5/2|√(m211+1)=1 ltbr> or (2m2−1)2=4(m22+1)
or −4m2−3=0
∴m2=−34 and ∞ (A coefficient of m22 is zero )
Substituting m2=−3/4 and ∞ in (2), the equatinos of transverse comon tangents are 3x+4y−10=0 and x=0
AB2=C1C22−(r1−r2)2
=20−(3−1)2=16
∴AB=4
CD2=C1C22−(r1+r2)2
=20−(3+1)2=4
∴CD=2
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - Examples13videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - CONCEPT APPLICATION EXERCISE 4.11videos
CIRCLE
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Matrix4videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLESEXERCISE - Comprehension Type8videos
CIRCLES
Similar Questions
The number of common tangents of the circles x2+y2−2x−1=0 and x2+y2−2y−7=0
View SolutionFind the number of common tangents to the circle x2+y2=4 and x2+y2−6x−8y−24=0
View SolutionThe number of common tangents to the circles x2+y2−x=0andx2+y2+x=0 are
View SolutionFind the equations to the common tangents of the circles x2+y2−2x−6y+9=0 and x2+y2+6x−2y+1=0
View SolutionFind the number of common tangent to the circles x2+y2+2x+8y−23=0 and x2+y2−4x−10y+9=0
View SolutionThe number of common tangents of the circles x2+y2=16 and x2+y2−2y=0 is :
View SolutionThe number of common tangents to the circles x2+y2−4x+6y+8=0andx2+y2−10x−6y+14=0 is : (A) 2 (B) 3 (C) 4 (D) none of these
View SolutionFind the number of common tangents to the circles x2+y2−8x+2y+8=0andx2+y2−2x−6y−15=0.
View SolutionThe number of common tangents of the circles x2+y2+4x+1=0 and x2+y2−2y−7=0, is
View SolutionThe number of common tangents to the circles x2+y2−4x−6y−12=0 and x2+y2+6x+18y+26=0, is
View Solution


