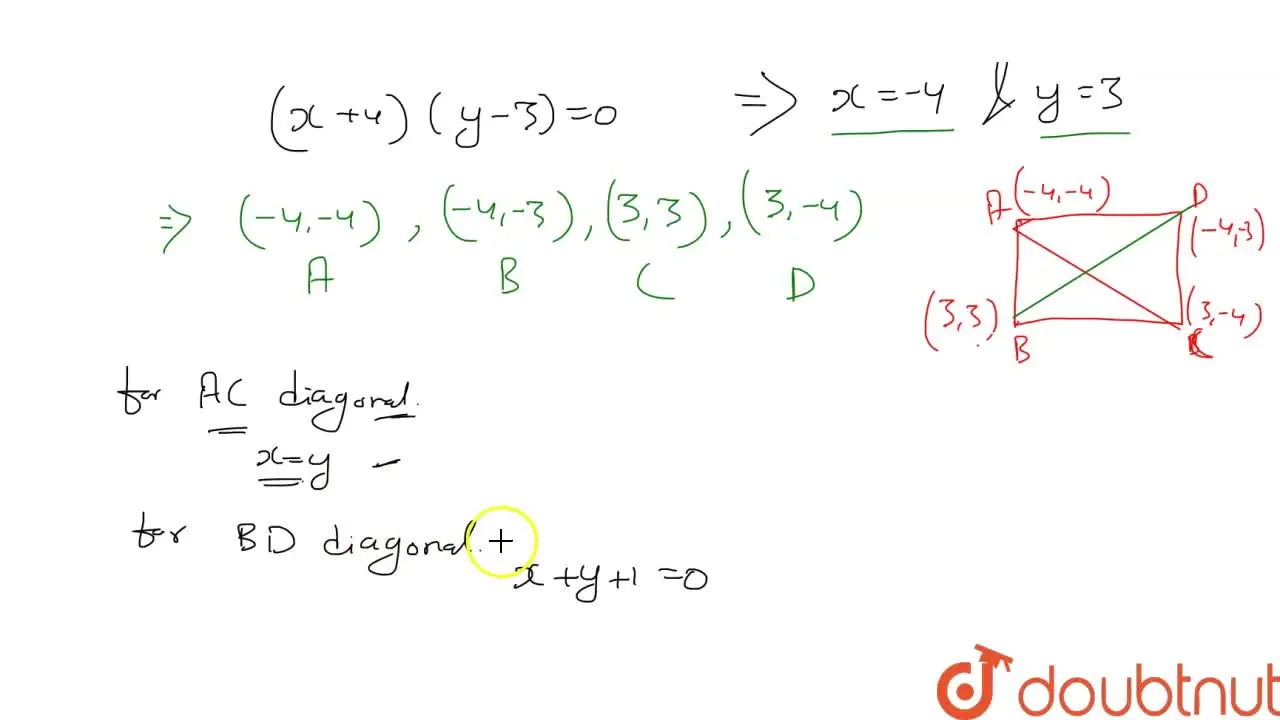
The equation of the diagonal of the square formed by the pairs of lines and is
The correct Answer is:A, B
The vertices are
Diagonal AC is and diagonal BD is .
Similar Questions
Find the equations of the diagonals of the square formed by the lines x=0,y=0,x=1 and y=1.
View SolutionEquations of diagonals of the square formed by the lines x=0,y=0,x=1 and y=1 are
View SolutionThe equation of the diagonal through origin of the quadrilateral formed by the lines x=0,y=0,x+y−1=0 and 6x+y−3=0, is
View SolutionThe circumcentre of the triangle formed by the lines, xy+2x+2y+4=0andx+y+2=0 is-
View SolutionThe circumcentre of the triangle formed by the lines, xy+2x+2y+4=0andx+y+2=0 is-
View SolutionThe in-radius of the triangle formed by the axes and the line 4x+3y–12=0 is :
View SolutionIf the area of the triangle formed by the pair of lines 8x2−6xy+y2=0 and the line 2x+3y=a is 7 then a=
View SolutionArea of the triangle formed by the lines x2+4xy+y2=0andx−y=4 is____
View SolutionThe bisector of the acute angle formed between the lines 4x - 3y + 7 = 0 and 3x - 4y + 14 = 0 has the equation
View SolutionThe bisector of the acute angle formed between the lines 4x - 3y + 7 = 0 and 3x - 4y + 14 = 0 has the equation
View Solution