Sixteen players S1, S2, S3,…,S16 play in a tournament. Number of ways in which they can be grouped into eight pairs so that S1 and S2 are in different groups, is equal to
(14)!26⋅6!
(15)!27⋅7!
(14)!27⋅6!
(14)!26⋅7!
The correct Answer is:A
To solve the problem of grouping 16 players into 8 pairs such that players S1 and S2 are in different groups, we can follow these steps:
Step 1: Calculate the total number of ways to pair 16 players
The total number of ways to pair 16 players into 8 pairs can be calculated using the formula:
Total ways=16!28×8!
Where:
- 16! is the total arrangements of 16 players.
- 28 accounts for the fact that within each pair, the order does not matter (i.e., (A,B) is the same as (B,A)).
- 8! accounts for the arrangement of the 8 pairs.
Step 2: Calculate the number of ways when S1 and S2 are in the same group
If S1 and S2 are in the same group, we can treat them as a single unit or pair. This leaves us with 14 players (the pair (S1,S2) plus the remaining 14 players).
The number of ways to pair these 15 units (the pair (S1,S2) and the 14 other players) is given by:
Ways with S1 and S2 together=14!27×7!
Where:
- 14! is the total arrangements of the 14 remaining players plus the pair.
- 27 accounts for the order within the 7 pairs.
- 7! accounts for the arrangement of the 7 pairs.
Step 3: Subtract the two results
To find the number of ways in which S1 and S2 are in different groups, we subtract the number of ways they are together from the total number of ways:
Required ways=16!28×8!−14!27×7!
Step 4: Simplify the expression
Now we can simplify the expression:
1. Factor out 14!27×7!:
Required ways=14!27×7!(16!14!×2×8−1)
2. Simplifying 16!14!=16×15:
=14!27×7!(16×152×8−1)
3. Calculate 16×1516=15:
=14!27×7!(15−1)=14!27×7!×14
4. Finally, we can express it as:
=14!26×6!
Final Answer
Thus, the number of ways in which the players can be grouped into pairs such that S1 and S2 are in different groups is:
14!26×6!
The total number of ways to pair 16 players into 8 pairs can be calculated using the formula:
- 16! is the total arrangements of 16 players.
- 28 accounts for the fact that within each pair, the order does not matter (i.e., (A,B) is the same as (B,A)).
- 8! accounts for the arrangement of the 8 pairs.
If S1 and S2 are in the same group, we can treat them as a single unit or pair. This leaves us with 14 players (the pair (S1,S2) plus the remaining 14 players).
- 14! is the total arrangements of the 14 remaining players plus the pair.
- 27 accounts for the order within the 7 pairs.
- 7! accounts for the arrangement of the 7 pairs.
To find the number of ways in which S1 and S2 are in different groups, we subtract the number of ways they are together from the total number of ways:
Now we can simplify the expression:
Thus, the number of ways in which the players can be grouped into pairs such that S1 and S2 are in different groups is:
Topper's Solved these Questions
- PERMUTATION AND COMBINATIONBOOK - CENGAGE ENGLISHCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Multiple Correct Answer
2 Videos
- PERMUTATION AND COMBINATIONBOOK - CENGAGE ENGLISHCHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Comprehension
8 Videos
- PARABOLABOOK - CENGAGE ENGLISHCHAPTER - PARABOLAEXERCISE - Matching Column Type
1 Video
- PRINCIPLE OF MATHEMATICAL INDUCTIONBOOK - CENGAGE ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTIONEXERCISE - Sovled Examples
22 Videos
Similar Questions
Knowledge Check
SN1 and SN2 reactions are
ABoth stereospecificBBoth stereoselectiveCStereoselective and stereospecific respectivelyDStereospecific and stereoselective respectively
If S0,S1,S2,… are areas bounded by the x-axis and half-wave of the curve y=sinπ√x, then prove that S0,S1,S2,… are in A.P…
View SolutionIdentify number of substrate those can give SN1 and SN2 reaction both.
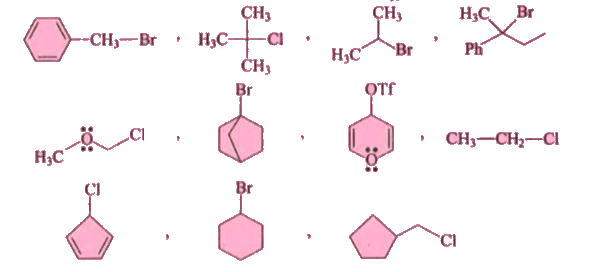
View SolutionThe number of hydroxyl group(s) in N is
_S01_496_Q01.png)
View SolutionIn a knockout tournament 2n equally skilled players, S1,S2,….S2n, are participatingl. In each round, players are divided in pair at random and winner from each pair moves in the next round. If S2 reaches the semi-final, then the probability that S1 wins the tournament is 1/84. The value of n equals _______.
View SolutionIf S1,S2,S3are the sum of first n natural numbers, their squares and their cubes, respectively, show that 9S22=S3(1+8S1).
View SolutionIf S1,S2,S3 are the sums of first n natural numbers, their squares and cubes respectively, show that 9S22=S3(1+8S1).
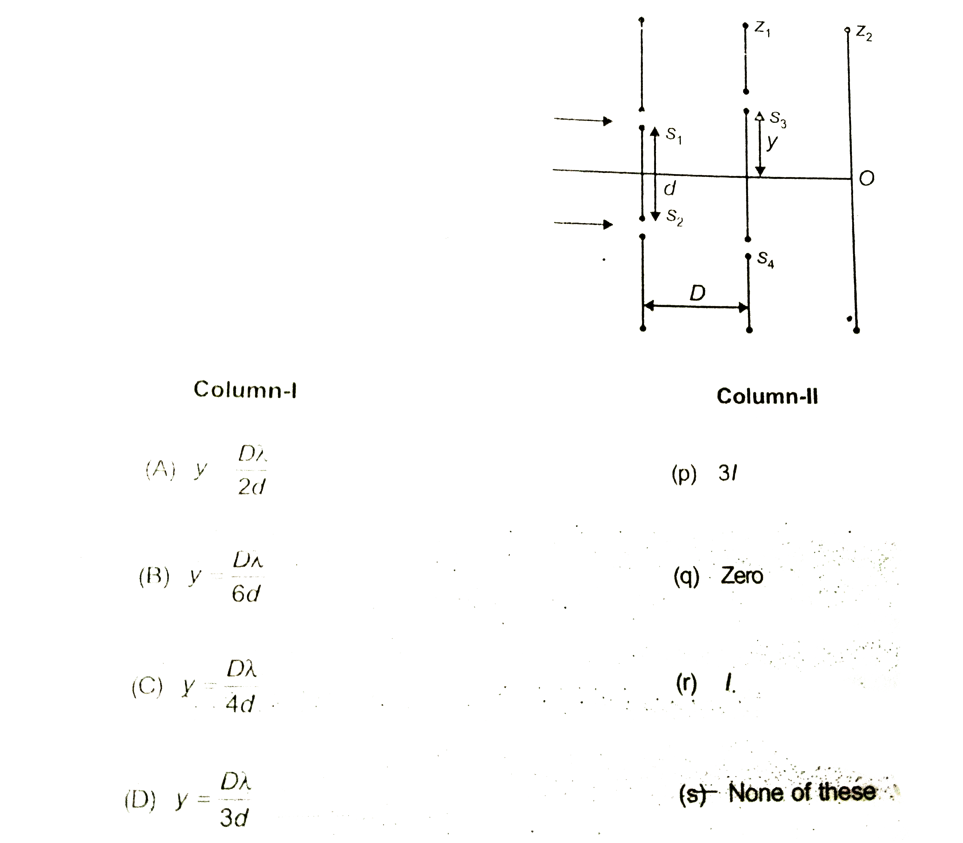
View SolutionIn the arrangement shown in figure, z1 and z2 are two screens. Line PO is the bisector line of s1s2 and s3s4,s1 is removed, resultant intensity at O due to slits s1 and s2 is l. now z1 is placed. For different values of y given in column-I match the resultant intensity at O given in column -II.
View Solution
