The first three terms of a geometric sequence are x, y,z and these have the sum equal to 42. If the middle term y is multiplied by 5/4, the numbers x, 5y4, z now form an arithmetic sequence. The largest possible value of x is
A
6
B
12
C
24
D
20
Video Solution
Text Solution
The correct Answer is:C
(c) The three terms of the geometric sequence with the common ratio r are x, xr, xr2. ∴x+xr+xr2=42
After multiplying the middle term by 5/4, we will get an arithmetic sequence. ∴54xr−x=xr2−54xr
∴2r2−5r+2=0∴r=12 and r=2
On substituting these in x+xr+xr2=42, we get
x=6 or 24
|
Topper's Solved these Questions
- PROGRESSION AND SERIESBOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - EXERCIESE ( MULTIPLE CORRECT ANSWER TYPE )
66 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - EXERCIESE ( MATRIX MATCH TYPE )
3 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE )
8 Videos
- PROBABILITY IIBOOK - CENGAGE ENGLISHCHAPTER - PROBABILITY IIEXERCISE - MULTIPLE CORRECT ANSWER TYPE
6 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Numerical Value Type)
3 Videos
Similar Questions
Knowledge Check
Two positive numbers, a and b, are in the sequence 4. a,b,12. The first three numbers form a geometric sequence, and the last three numbers form an arithmetic sequence. The difference b-a equals
A1B112C2D3Three consecutive terms, in order, of an arithmetic sequence are x+√2,2x+√3,and5x−√5. Then x equals
A2.14B2.45C2.46D3.24
Identify X, Y and Z in the given sequence of reactions.
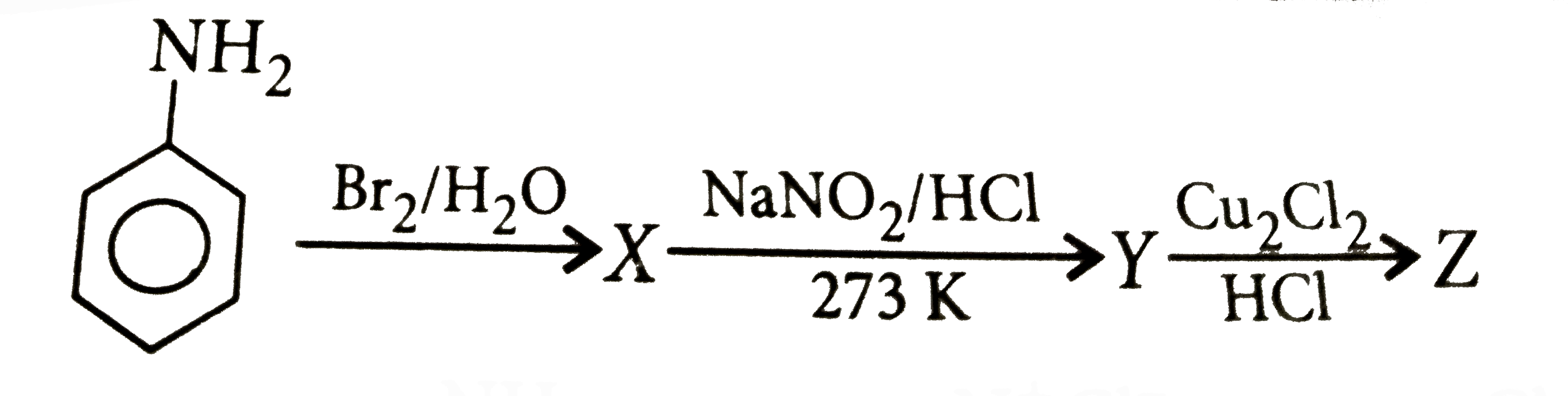
View SolutionIdentify X,Y and Z in the given sequence of reactions
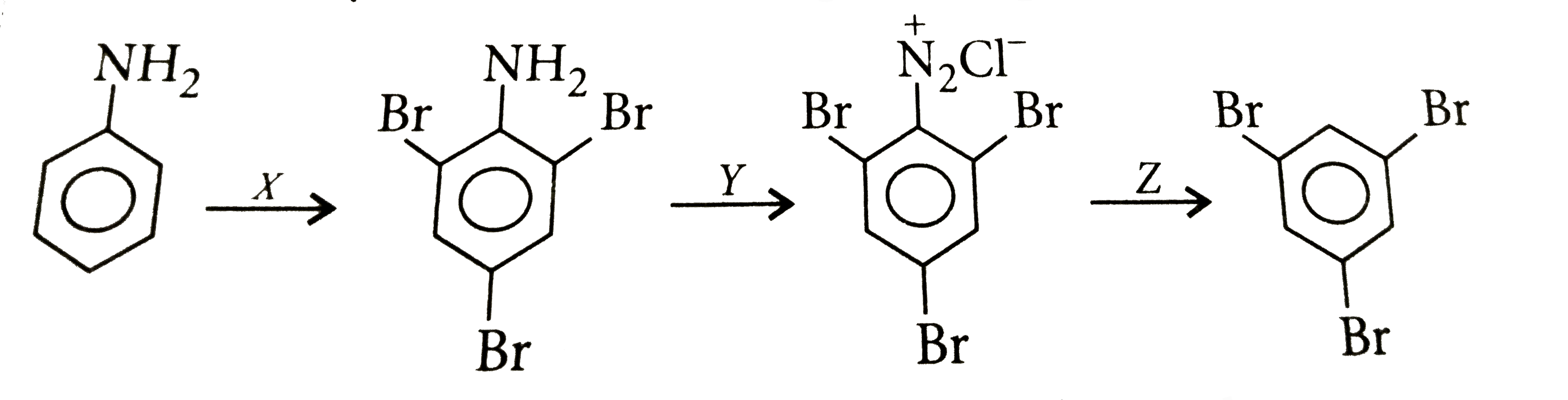
View SolutionIf 5, x, y, z, 405 are the first five terms of a geometric progression, find the values of x, y, and z.
View SolutionIf the 4th, 10th and 16th terms of a G.P. are x,y and z respectively, then x,y,z are in
View SolutionIf x, y, z are positive real numbers such that x3y2z4=7, then the least value of 2x+5y+3z, is
View SolutionThree distinct numbers x,y,z form a GP in that order and the numbers 7x+5y,7y+5z,7z+5x form an AP in that order. The common ratio of GP is
View Solution