Evaluate: ∫cos2xsin4xdxcos4x(1+cos22x)
Video Solution
Text Solution
The correct Answer is:−12log|cos2x|−12(1+cos2x)+14log(1+cos22x)+C
I=∫cos2xsin4xdxcos4x(1+cos22x)
=∫2cos22xsin2xdx(1+cos2x2)2(1+cos22x)
Let cos2x=t. then dt=−2sin2xdx.
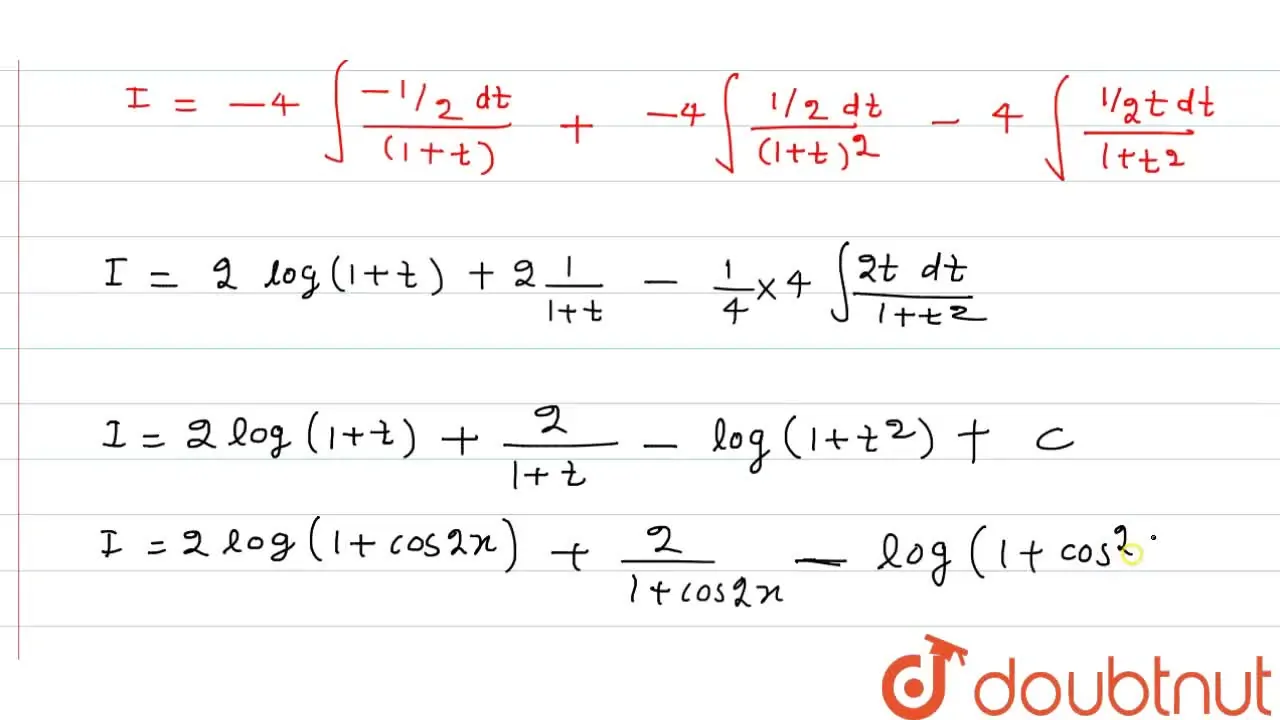
∴I=−∫t2dt(1+t2)2(1+t2)=−4∫t2dt(1+t)2(1+t2)
Now ,t2(1+t)2(1+t2)=A1+t+B(1+t)2+Ct+D1+t2
or t2=A(1+t)(1+t2)+B(1+t2)+(Ct+D)(1+t)2
Put t=−1. Then B=1/2
Put t=0. Then 0=A+1/2+D (1)
Put t=1. Then 1=4A+1+4C+4D or A+C+D=0 (2)
From equations(1)and(2), C=1/2
Comparing coefficients of t3,A+C=0 (3)
or A=−1/2
From equations(2)and(3),D=0
Hence, I=∫(−1/21+t+1/2(1+t)2+(1/2)t1+t2)dt
=−12log|t|−12(1+t)+14log(1+t2)+C
=−12log|t|−12(1+t)+14log(1+t2)+C, where t=cos2x
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - INDEFINITE INTEGRATIONEXERCISE - CONCEPT APPLICATION EXERCISE 7.915videos
INDEFINITE INTEGRATION
- BOOK - CENGAGE ENGLISHCHAPTER - INDEFINITE INTEGRATIONEXERCISE - EXERCISES (Single Correct Answer Type)77videos
INDEFINITE INTEGRATION
- BOOK - CENGAGE ENGLISHCHAPTER - INDEFINITE INTEGRATIONEXERCISE - CONCEPT APPLICATION EXERCISE 7.710videos
INDEFINITE INTEGRATION
- BOOK - CENGAGE ENGLISHCHAPTER - HYPERBOLAEXERCISE - COMPREHENSION TYPE2videos
HYPERBOLA
- BOOK - CENGAGE ENGLISHCHAPTER - INEQUALITIES AND MODULUSEXERCISE - Single correct Answer21videos
INEQUALITIES AND MODULUS
Similar Questions
Evaluate: ∫sin3xdx(cos4x+3cos2x+1)tan−1(secx+cosx)
View SolutionEvaluate: ∫(1+x2)cos2x dx
View SolutionEvaluate: ∫cos2x+2 sin2xcos2x dx
View SolutionEvaluate: ∫14cos2x+9sin2xdx
View SolutionEvaluate: ∫cos2xs∈2xcos2x dx
View SolutionEvaluate: ∫cos2x−sin2x√1+cos4x dx
View SolutionEvaluate: ∫cos5x+cos4x1−2cos3xdx
View SolutionEvaluate: ∫14cos2x+9sin2x dx
View SolutionEvaluate: ∫cos5x+cos4x1−2cos3x dx
View SolutionEvaluate: ∫cos5x+cos4x1−2cos3x dx
View Solution