G is the centroid of triangle ABC and A1 and B1 are the midpoints of sides AB and AC, respectively. If Δ1 is the area of quadrilateral GA1AB1 and Δ is the area of triangle ABC, then ΔΔ1 is equal to
32
3
13
none of these
The correct Answer is:b
To solve the problem, we need to find the ratio of the area of triangle ABC (denoted as Δ) to the area of quadrilateral GA₁AB₁ (denoted as Δ₁).
1. Identify the Points and Vectors:
- Let the position vectors of points A, B, and C be represented as A, B, and C.
- The midpoints A₁ and B₁ are given by:
- A₁ = (A + B) / 2
- B₁ = (A + C) / 2
- The centroid G of triangle ABC is given by:
- G = (A + B + C) / 3
2. Calculate the Area of Triangle ABC (Δ):
- The area of triangle ABC can be calculated using the formula:
Δ=12|AB×AC|
- Here, AB = B - A and AC = C - A.
- Thus, we can express the area as:
Δ=12|(B−A)×(C−A)|
3. Calculate the Diagonal Vectors for Quadrilateral GA₁AB₁:
- The diagonal GA₁ can be calculated as:
GA₁=A₁−G=(A+B)2−(A+B+C)3=3(A+B)−2(A+B+C)6=A+B−2C6
- The diagonal A₁B₁ can be calculated as:
A₁B₁=B₁−A₁=(A+C)2−(A+B)2=C−B2
4. Calculate the Area of Quadrilateral GA₁AB₁ (Δ₁):
- The area of the quadrilateral can be calculated using the cross product of the diagonals:
Δ₁=12|GA₁×A₁B₁|
- Substitute the expressions for GA₁ and A₁B₁:
Δ₁=12∣∣∣A+B−2C6×C−B2∣∣∣=124|(A+B−2C)×(C−B)|
5. Finding the Ratio Δ/Δ₁:
- Now we can find the ratio:
ΔΔ₁=12|(B−A)×(C−A)|124|(A+B−2C)×(C−B)|
- Simplifying this gives:
ΔΔ₁=12|(B−A)×(C−A)||(A+B−2C)×(C−B)|
6. Final Calculation:
- After simplifying the areas and using properties of cross products, we find that:
ΔΔ₁=2
Conclusion:
Thus, the ratio of the area of triangle ABC to the area of quadrilateral GA₁AB₁ is:
ΔΔ₁=2
- Let the position vectors of points A, B, and C be represented as A, B, and C.
- The midpoints A₁ and B₁ are given by:
- A₁ = (A + B) / 2
- B₁ = (A + C) / 2
- The centroid G of triangle ABC is given by:
- G = (A + B + C) / 3
- The area of triangle ABC can be calculated using the formula:
Δ=12|AB×AC|
- Here, AB = B - A and AC = C - A.
- Thus, we can express the area as:
Δ=12|(B−A)×(C−A)|
- The diagonal GA₁ can be calculated as:
GA₁=A₁−G=(A+B)2−(A+B+C)3=3(A+B)−2(A+B+C)6=A+B−2C6
- The diagonal A₁B₁ can be calculated as:
A₁B₁=B₁−A₁=(A+C)2−(A+B)2=C−B2
- The area of the quadrilateral can be calculated using the cross product of the diagonals:
Δ₁=12|GA₁×A₁B₁|
- Substitute the expressions for GA₁ and A₁B₁:
Δ₁=12∣∣∣A+B−2C6×C−B2∣∣∣=124|(A+B−2C)×(C−B)|
- Now we can find the ratio:
ΔΔ₁=12|(B−A)×(C−A)|124|(A+B−2C)×(C−B)|
- Simplifying this gives:
ΔΔ₁=12|(B−A)×(C−A)||(A+B−2C)×(C−B)|
- After simplifying the areas and using properties of cross products, we find that:
ΔΔ₁=2
Thus, the ratio of the area of triangle ABC to the area of quadrilateral GA₁AB₁ is:
ΔΔ₁=2
Topper's Solved these Questions
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS Book:CENGAGE ENGLISHChapter:DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS Exercise:Reasoning type
Explore 8 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS Book:CENGAGE ENGLISHChapter:DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS Exercise:Comprehension type
Explore 27 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS Book:CENGAGE ENGLISHChapter:DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS Exercise:Multiple correct answers type
Explore 11 Videos
- CONIC SECTIONSBook:CENGAGE ENGLISHChapter:CONIC SECTIONSExercise:All Questions
Explore 1344 Videos
- LIMITS AND DERIVATIVESBook:CENGAGE ENGLISHChapter:LIMITS AND DERIVATIVESExercise:All Questions
Explore 691 Videos
Similar Questions
G is the centroid of triangle ABCandA1andB1 are rthe midpoints of sides ABandAC, respectively. If Delta1 is the area of quadrilateral GA1AB1andDelta is the area of triangle ABC, then Delta/Delta1 is equal to a.32 b. 3 c. 13 d. none of these
View SolutionArea of quadrilateral ABCD =Area of ΔABC+ area of _______
View SolutionIn the given triangle P, Q and R are the mid points of sides AB, BC and AC respectively. Prove that triangle PQR is similar to triangle ABC.

View SolutionIn a ΔABC,D,EandF are the mid-point of sides BC, CA and AB respectively. If area of ΔABC=16cm2, find the area of trapezium FBCE
View SolutionD, E and F are the mid-points of the sides BC, CA and AB respectively of triangle ABC. Prove that:
area of BDEF is half the area of ΔABC.
View SolutionIf the area of triangle ABC is 15, what is the length of AC ?
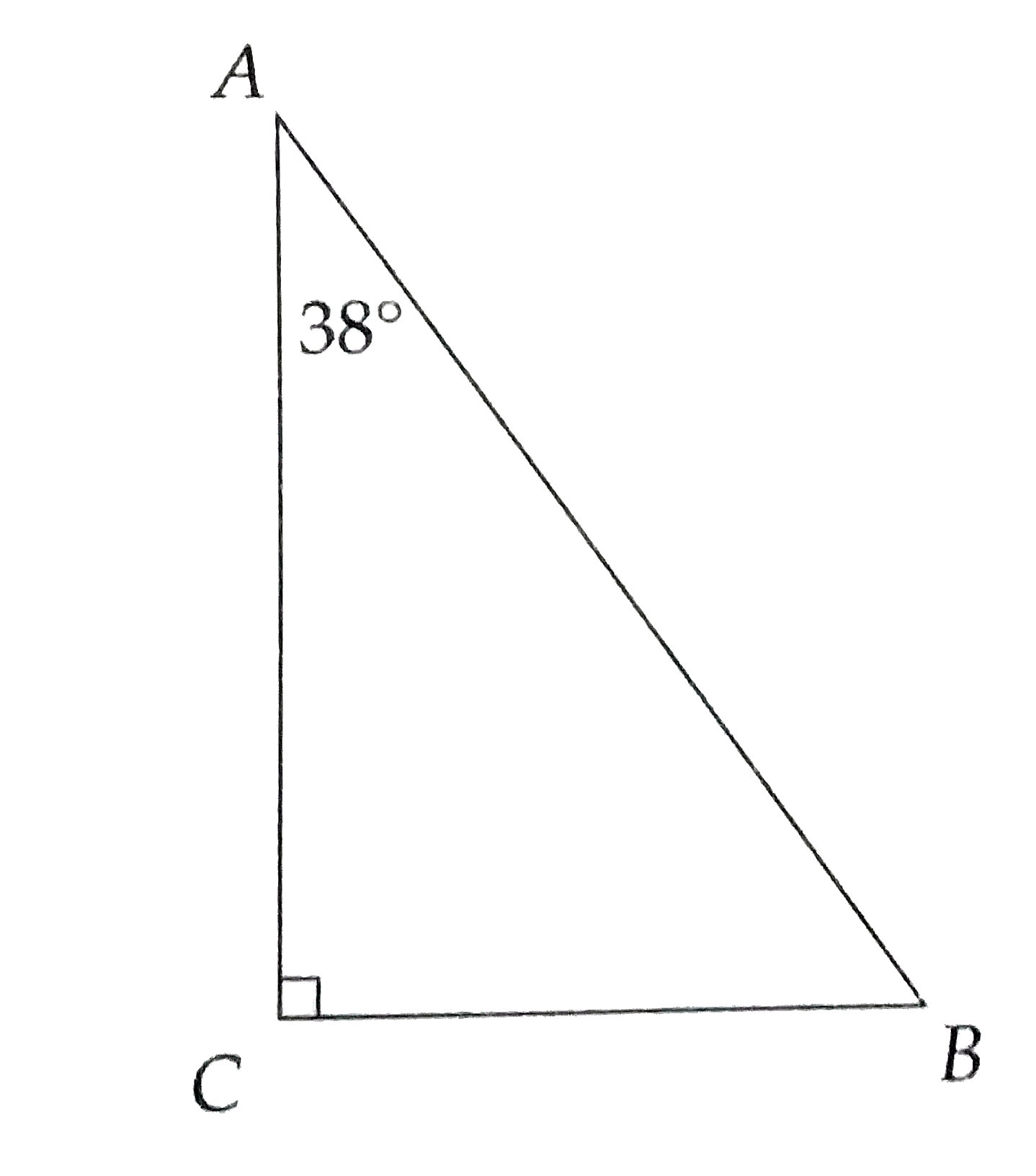
View SolutionLet the area of a given triangle ABC be Δ. Points A1,B1,andC1 , are the mid points of the sides BC,CA and AB respectively. Point A2 is the mid point of CA1. Lines C1A1andAA2 meet the median BB1 points E and D respectively. If Δ1 be the area of the quadrilateral A1A2DE, using vectors or otherwise find the value of Δ1Δ
View SolutionIn the adjoning figure, D and E are the points on the sides AB and AC respectively of ΔABC and area of ΔBCE= area of ΔBCD.
Prove that DE∣∣BC

View SolutionIn a ΔABC,PandQ are the mid-points of ABandAC respectively. If O is the circumcentre of the ΔABC, then the value of (Area of ΔABCArea of ΔOPQ)cotBcotC equal to
View SolutionAL, BM and CN are perpendicular from angular points of a triangle ABC on the opposite sides BC, CA and AB respectively. Δ is the area of triangle ABC, (r ) and R are the inradius and circumradius.
If area of ΔLMN is Δ', then the value of Δ'Δ is
View Solution