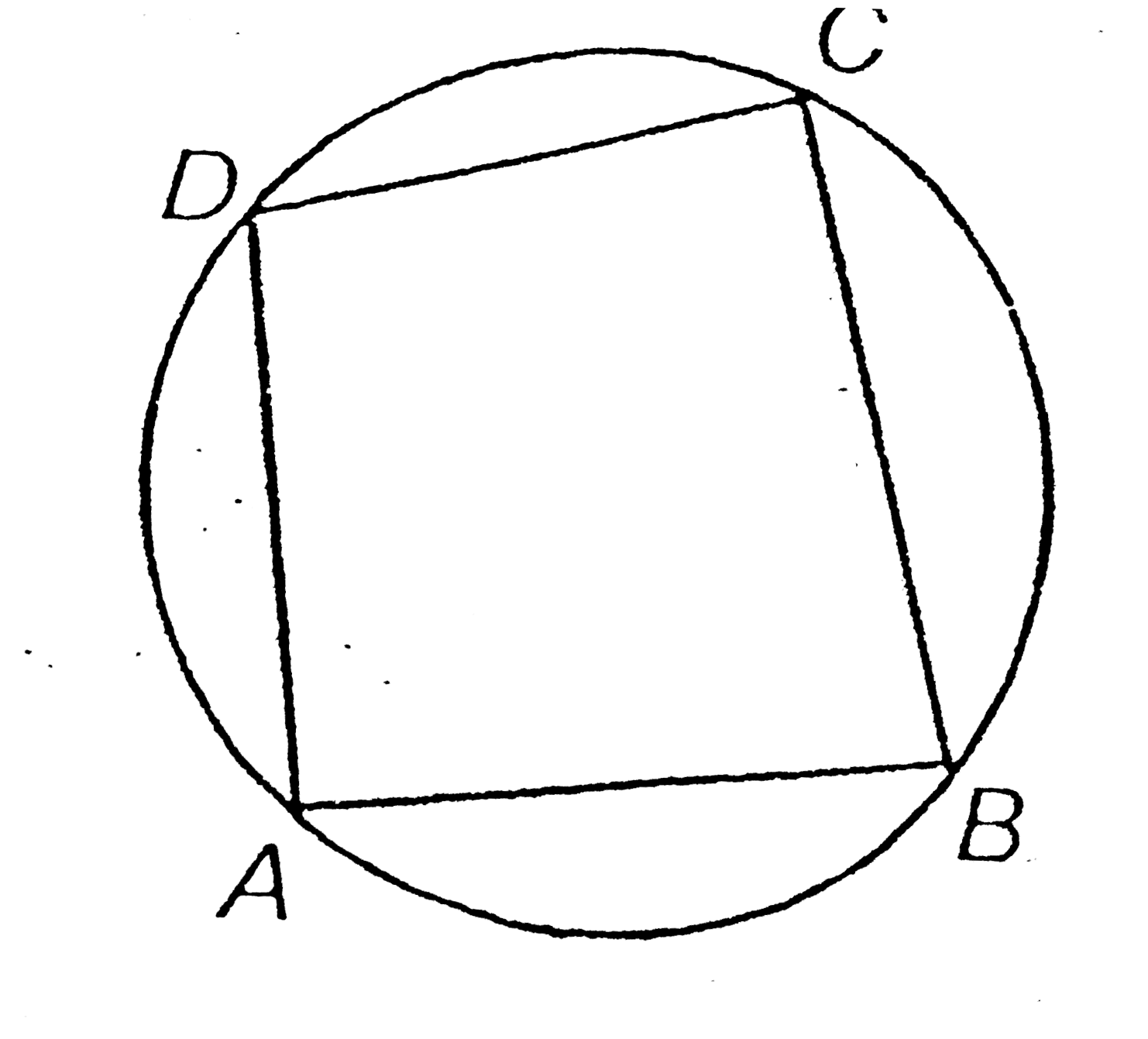
If →a,→b,→cand→d are the position vectors of the vertices of a cycle quadrilateral ABCD, prove that ∣∣→a×→b+→b×→d+→d×→a∣∣(→b−→a).(→d−→a)+∣∣→b×→c+→c×→d+→d+→d×→b∣∣(→b−→c).(→d−→c)
∣∣→a×→b+→b×→d+→d×→a∣∣(→b−→a).(→d−→a)=∣∣(→a−→d)×(→b−→a)∣∣(→b−→a).(→d−→a)
=∣∣→a−→d∣∣∣∣→b−→a∣∣sinA∣∣→b−→a∣∣∣∣→d−→a∣∣cosA
tanA
Also∣∣→b×→c+→c×→d+→d×→b∣∣(→b−→c).(→d−→c)=∣∣(→b−→c)×(→c−→d)∣∣(→b−→a).(→d−→c)
=∣∣→b−→c∣∣∣∣→c−→d∣∣sinC∣∣→b−→c∣∣.∣∣→d−→c∣∣cosA
tanC
As it is a cycle quadrilateral, we have
A=180∘−C
tanA=tan(180∘−C)
tanA+tanC=0
∣∣→a×→b+→b×→d+→d×→a∣∣(→b−→a).(→d−→a)+∣∣→b×→c+→c×→d+→d×→b∣∣(→b−→c).(→d−→c)

Topper's Solved these Questions
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Exercise 2.1
18 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Exercise 2.2
15 Videos
- DETERMINANTSBOOK - CENGAGE ENGLISHCHAPTER - DETERMINANTSEXERCISE - All Questions
264 Videos
- DIFFERENTIAL EQUATIONSBOOK - CENGAGE ENGLISHCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Matrix Match Type
5 Videos
Similar Questions
If →a,→b,→c,→d are the position vectors of the verticles of a cyclic quadrilateral ABCd prove that
∣∣→a×→b+→b×→d+→d×→a∣∣(→b−→a).(→d−→a)+∣∣→b×→c+→c×→d+→d×→b∣∣(→b−→c).(→d−→c)=0
View SolutionProve that: [(→a×→b)×(→a×→c)].→d=[→a→b→c](→a.→d)
View SolutionProve that: (→a×→b)×(→c×→d)+(→a×→c)×(→d×→b)+(→a×→d)×(→b×→c) = −2[→b→c→d]→a
View SolutionProve that →a×{→b×(→c×→d)}=(→b.→d)(→a×→c)−(→b.→c)(→a×→d)
View SolutionIf →a,→b,→cand→d ar distinct vectors such that →a×→c=→b×→dand→a×→b=→c×→d. Prove that (→a−→d).(→c−→b)≠0,i.e.,→a.→b+→d.→c≠→d.→b+→a.→c.
View SolutionShow that: (→a−→d)×(→b−→c)+(→b−→d)×(→c−→a)+(→c−→d)×(→a−→b) is independent of →d.
View SolutionIf [→a→b→c]=1 then value of →a.→b×→c→c×→a.→b+→b.→c×→a→a×→b.→c+→c.→a×→b→b×→c.→a is
View SolutionIf vectors, →b,→cand→d are not coplanar, the prove that vector (→a×→b)×(→c×→d)+(→a×→c)×(→d×→b)+(→a×→d)×(→b×→c) is parallel to →a.
View SolutionIf →a,→b,→cand→a',→b',→c' are reciprocal system of vectors, then prove that →a'×→b'+→b'×→c'+→c'×→a'=→a+→b+→c[→a→b→c]
View SolutionIf →a,→b,→c,→d are four distinct vectors satisfying the conditions →a×→b=→c×→dand→a×→c=→b×d then prove that →a.→b+→c.→d≠→a.→c+→b.→d
View Solution
