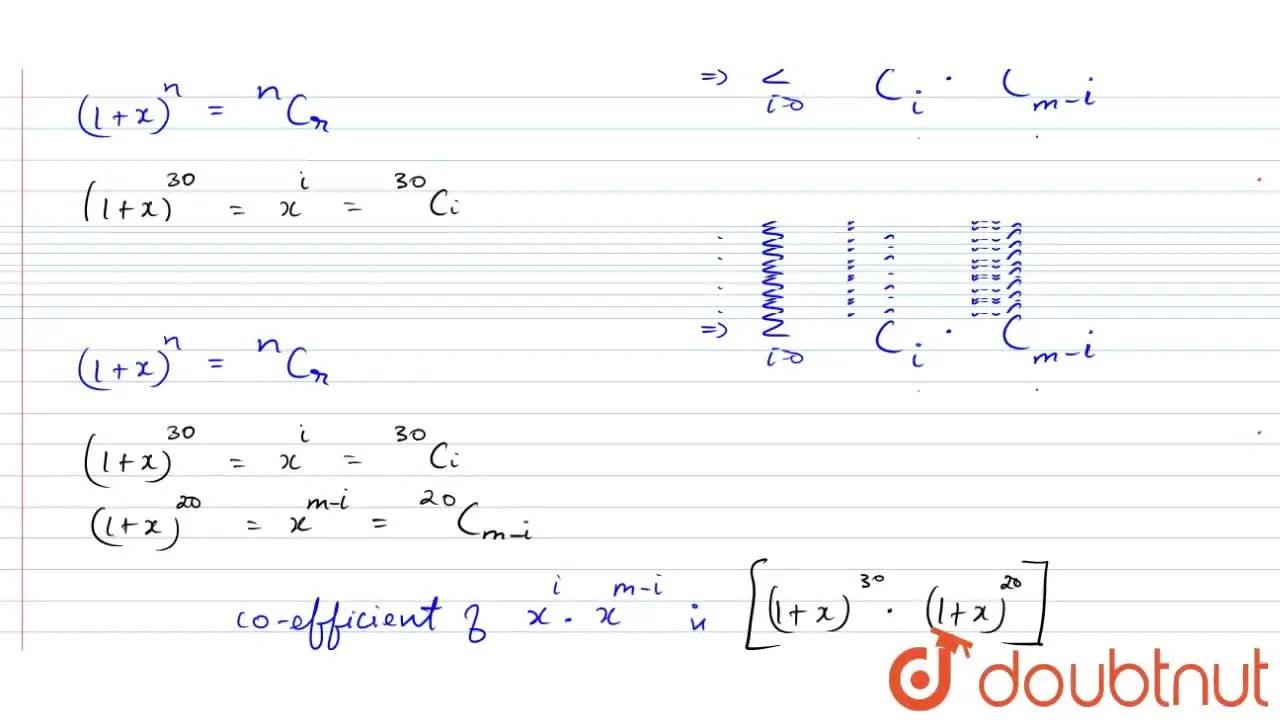
If where , then
maximum value of is
is always divisible by
The value of
The correct Answer is:A, B, D
is greatest when . Also,
Also, is not divisible by 50 for all m as 50 is not a prime number
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Linked Comphrension20videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Numerical25videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Single Correct Answer152videos
BINOMIAL THEOREM
- BOOK - CENGAGE ENGLISHCHAPTER - AREAEXERCISE - Comprehension Type2videos
AREA
- BOOK - CENGAGE ENGLISHCHAPTER - CIRCLE EXERCISE - MATRIX MATCH TYPE7videos
CIRCLE
Similar Questions
The sum i=0∑m(10i)(20m−i), (where (pq)=0, if p<q) is maximum when 'm' is
View SolutionThe sum i=0∑m(10i)(20m−i), (where (pq)=0, if p<q) is maximum when 'm' is
View SolutionIf f(m)=m∑i=0(30(^)30−i)(20(^)m−i)where(pq)=pCq,then (a) maximum value of f(m)is50C25 (b)f(0)+f(1)+...f(50)=250 (c)f(m) is always divisible by 50(1≤m≤49) (d)The value of 50∑m=0(f(m))2=100C50
View SolutionA=⎡⎢⎣l1m1n1l2m2n2l3m3n3⎤⎥⎦ and B=⎡⎢⎣p1q1r1p2q2r2p3q3r3⎤⎥⎦
Where pi,qi,ri are the co-factors of the elements li,mi,ni for i=1,2,3. If (l1,m1,n1),(l2,m2,n2) and (l3,m3,n3) are the direction cosines of three mutually perpendicular lines then (p1,q1,r1),(p2,q2,r2) and (p3,q,r3) areView SolutionThree particles of masses m,m and 4kg are kept at a verticals of triangle ABC. Coordinates of A, B and C are (1,2) , (3,2) and (−2,−2) respectively such that the centre of mass lies at origin. Find the value of mass m.
Hint. xcm=∑3i−1mixi∑3i=1mi, ycm=∑3i=1miyi∑3i=1miView SolutionThree particles of masses m,m and 4kg are kept at a verticals of triangle ABC. Coordinates of A, B and C are (1,2) , (3,2) and (−2,−2) respectively such that the centre of mass lies at origin. Find the value of mass m.
Hint. xcm=∑3i−1mixi∑3i=1mi, ycm=∑3i=1miyi∑3i=1miView SolutionIf angles of a linear pair are equal, then the measure of each angle is (a) (b)(c)(d)(e)30(f)0(g)(h)(i) (j) (b) (k)(l)(m)(n)45(o)0(p)(q)(r) (s) (c) (d)(e)(f)(g)60(h)0(i)(j)(k) (l) (d) (m)(n)(o)(p)90(q)0(r)(s)(t) (u)
View SolutionThe measure of an angle which is its own complement is (a) (b)(c)(d)(e)30(f)0(g)(h)(i) (j) (b) (k)(l)(m)(n)60(o)0(p)(q)(r) (s) (c) (d)(e)(f)(g)90(h)0(i)(j)(k) (l) (d) (m)(n)(o)(p)45(q)0(r)(s)(t) (u)
View SolutionIn Fig.88 PR is a straight line and ∠PQS:∠SQR=7:5. The measure of ∠SQR is (a) (b)(c)(d)(e)60(f)0(g)(h)(i) (j) (b) (k)(l)62(m)(n)(o)1p2(q)(r)(s)0(t)(u)(v) (w) (c) (d)(e)67(f)(g)(h)1i2(j)(k)(l)0(m)(n)(o) (p) (d) (q)(r)(s)(t)75(u)0(v)(w)(x) (y)
View SolutionThree particles having their masses in the ratio 1:3:5 are kept at the vertices of a triangle ABC. Coordinate of A, B and C are (9,−3), (3,4) and (0,0). Find the coordinates of the centre of mass.
Hint. xcm=∑3i−1mixi∑3i=1mi, ycm=∑3i=1miyi∑3i=1miView Solution