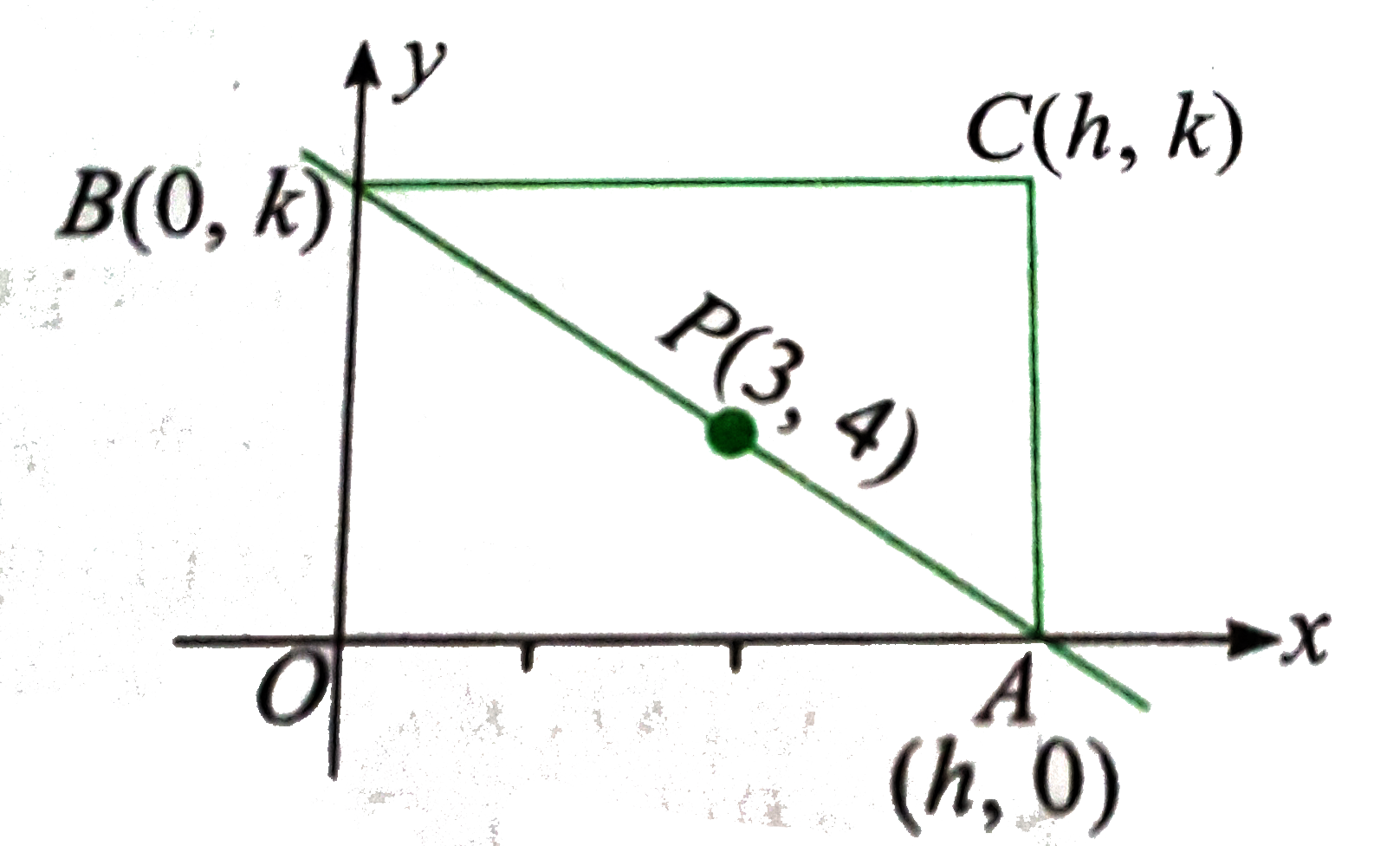
A straight line is drawn through P(3,4) to meet the axis of x and y at AandB , respectively. If the rectangle OACB is completed, then find the locus of C.
The correct Answer is:3x+4y=1
Let the point C be (h,k).
Then the coordinates of A and B are (h,0)and90,k), respectively, Since points A,P, and B are collinear, we have
∣∣
∣
∣
∣∣h0340kh0∣∣
∣
∣
∣∣=0
or 4h+3k−hk=0
or 4x+3y−xy=0
Therefore, the required locus is 3x+4y=1
Topper's Solved these Questions
- COORDINATE SYSYEM BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Exercises
59 Videos
- COORDINATE SYSYEM BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Multiple correct
13 Videos
- COORDINATE SYSYEM BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.5
5 Videos
- COORDINATE SYSTEMBOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type
2 Videos
- CROSS PRODUCTS BOOK - CENGAGE ENGLISHCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.2
13 Videos
Similar Questions
Tangent and normal drawn to a parabola at A(at2,2at),t≠0 meet the x-axis at point B and D , respectively. If the rectangle ABCD is completed, then the locus of C is
(a)y=2a (b) y+2a=c (c)x=2a (d) none of these
View SolutionA tangent is drawn to the parabola y2=4ax at P such that it cuts the y-axis at Q. A line perpendicular to this tangents is drawn through Q which cuts the axis of the parabola at R . If the rectangle PQRS is completed, then find the locus of S..
View SolutionThe ends A and B of a straight line segment of constant length c slide upon the fixed rectangular axes OX and OY, respectively. If the rectangle OAPB be completed, then the locus of the foot of the perpendicular drawn from P to AB is
View SolutionFind the equation of the line which passes through P(1,−7) and meets the axes at AandB respectively so that 4AP−3BP=0.
View SolutionIf a tangent to the parabola y2=4ax meets the x-axis at T and intersects the tangents at vertex A at P, and rectangle TAPQ is completed, then find the locus of point Q.
View SolutionIf a tangent to the parabola y2=4ax meets the x-axis at T and intersects the tangents at vertex A at P, and rectangle TAPQ is completed, then find the locus of point Q.
View SolutionThe straight line through a fixed point (2,3) intersects the coordinate axes at distinct point P and Q. If O is the origin and the rectangle OPRQ is completed then the locus of R is
View SolutionFind the equation of the line which passes through P(1,−7) and meets the axes at A and B respectively so that 4AP−3BP=0.
View SolutionConsider two lines L1andL2 given by a1x+b1y+c1=0anda2x+b2y+c2=0respectivelywherec1andc2≠0, intersecting at point P.A line L3 is drawn through the origin meeting the lines L1andL2 at AandB, respectively, such that PA=PB . Similarly, one more line L4 is drawn through the origin meeting the lines L1andL2 at A1andB2, respectively, such that PA1=PB1. Obtain the combined equation of lines L3andL4.
View SolutionIf a tangent to the parabola y2=4ax meets the x-axis in T and the tangent at the Vertex A in P and the rectangle TAPQ is completed then locus of Q is
View Solution
