Question

Find the area bounded by $y={\sin }^{-1}\left(\sin x\right)$ and x-axis for $x\in [0,100\pi ]$

Explanation

`y sin^(-1) (sin x)` is periodic with period `2 pi`
`:.` Required area `= 50 xx` (area bounded by `y = sin^(-1) (sin x)` and x a-axis for `x in [0, 2pi])`
Graph of `y = sin^(-1) (sin x) " for " x in [0, 2pi]` is as shown in the following figure.

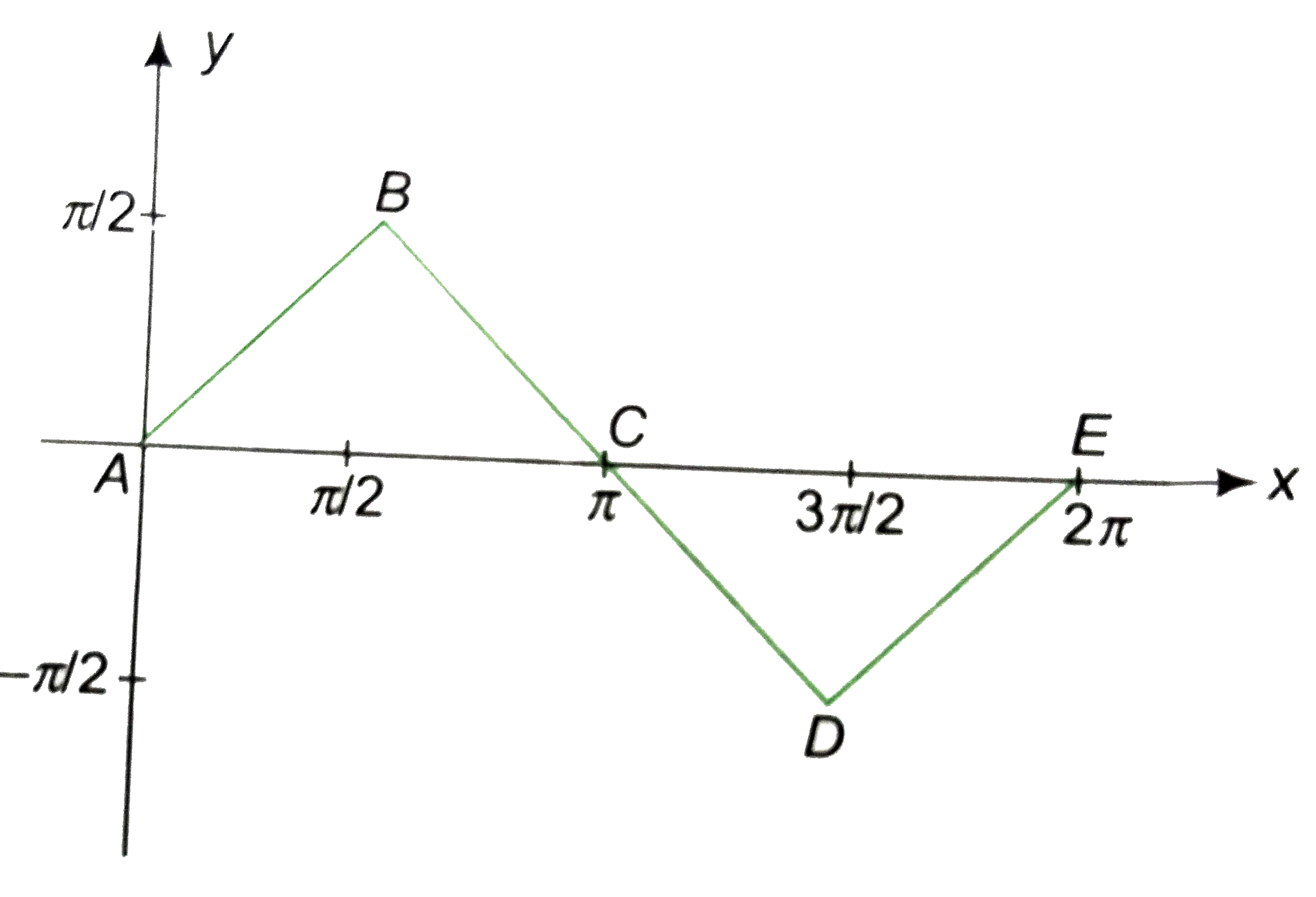
Required area `= 50 xx (" area of " Delta ABC + "area of " Delta CDE)`
`= 100 xx ("area of " Delta ABC)`
`= 100 xx ((1)/(2) xx pi xx (pi)/(2))`
`25 pi^(2)`