Evlute $[lim t_{x \to 0} \frac{{tan}^{- 1} x}{x}]$ , where $[\cdot]$ re[resemts the greatest integer function.

Video Solution

Text Solution

Verified by Experts

See the graphs of y=x and ${tan}^{- 1} x$ in the following figure.

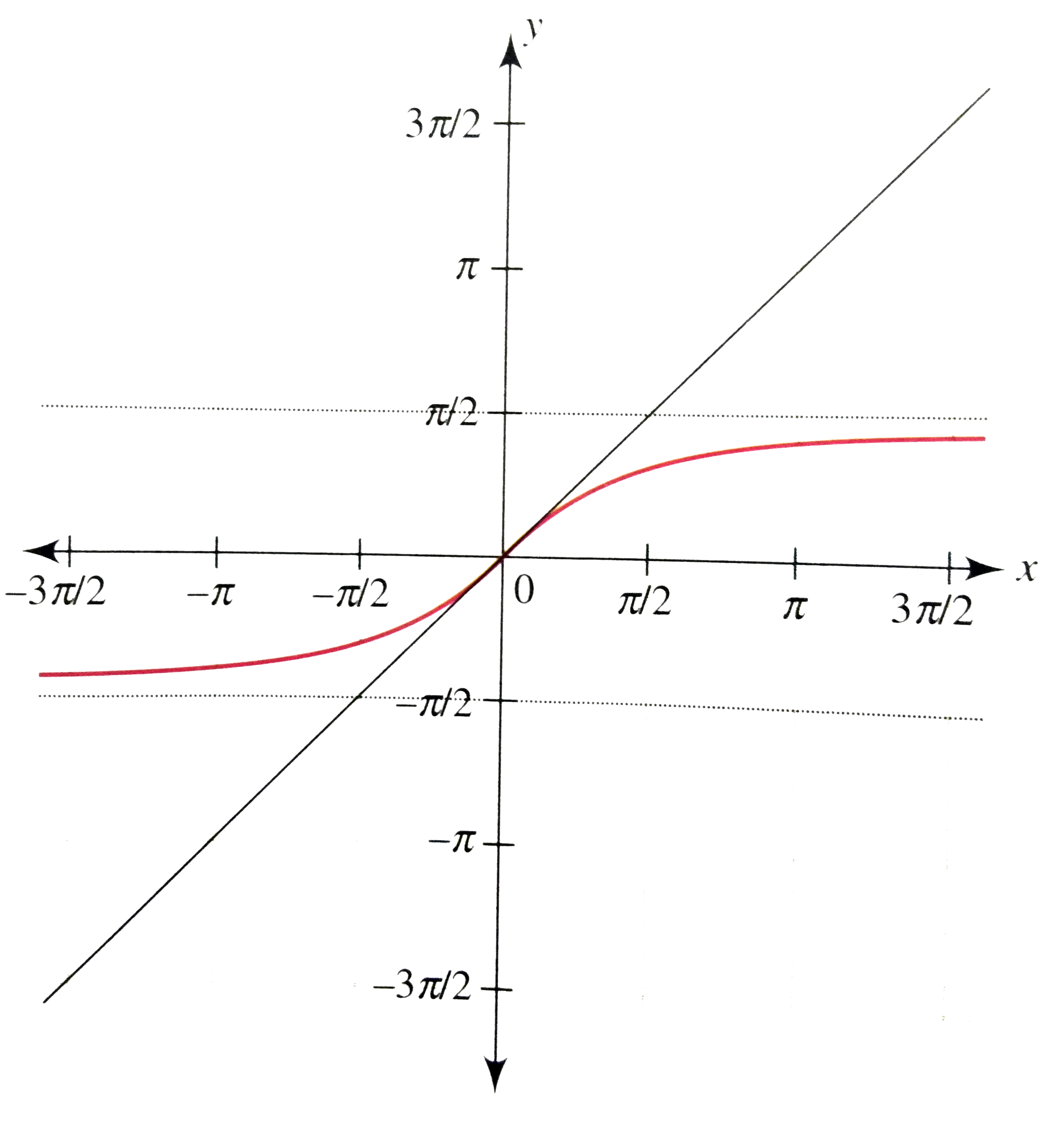
For $f (x) = {tan}^{- 1} (x) = \frac{1}{1 + x^{2}}$
$∴ f' (0) = 1$
So the curve touches the line y = x at x = 0
For $x > 0, f' (x) < 1$
So the graph of $y = {tan}^{- 1} x$ lies below the graph of y = x.
From the graph, when $x \to o^{+}$ , the graph of y = x is above the graph of $y = {tan}^{- 1} x$
$or {tan}^{- 1} x < x \Rightarrow \frac{{tan}^{- 1} x}{x} < 1 \Rightarrow [lim x \to 0^{+} \frac{tan x}{x}] = 0$
$Thus [lim x \to 0^{+} \frac{tan x}{x}] = 0$

|

Updated on:21/07/2023

Class 12 MATHS INVERSE TRIGONOMETRIC FUNCTIONS

Topper's Solved these Questions

Similar Questions

CENGAGE ENGLISH-GRAPH OF INVERSE TRIGONOMETRIC FUNCTIONS-Exercises

Home
Ask Doubt
Profile