Let M
be a 2×2
symmetric matrix with integer entries. Then M
is invertible if
a. The first column of M
is the transpose of the second
row of M
b. The second row of M
is the transpose of the first
column of M
c. M
is a diagonal matrix with
non-zero entries in the main diagonal
d. The product of entries in the main diagonal of M
is not the square of an integer
the first column of M is the transpose of the second row of M
the second row of M is the transpose of the column of M
M is a diagonal matrix with non-zero entries in the main diagonal
the product of entries in the main diagonal of M is not the square of an integer
The correct Answer is:C, D
Let M=[accb] (where a,b,c,∈I)
(1) If the first column of M is the transpose of the second row of M, then
[ac]=[cb]
∴A=b=c
Thus, det. (M)=ab−c2=0
Hence, M is not invertible.
(2) If the second row of M is the transpose of the first column of M, then
[cb]=[ac]
∴a=b=c
thus, det (M)=ab−c2=0
Hence, M is not invertible.
(3) If M=[a00b], with a,b,≠0, then
det. (M)=ab≠0
Hence, M is invertible.
(4) If product of elements in main diagonal which (ab) is not perfect square, then
det. (M)=ab−c2≠0
Hence, M is invertiable.
Topper's Solved these Questions
- MATRICESBOOK - CENGAGE ENGLISHCHAPTER - MATRICESEXERCISE - Linked Comprehension Type
27 Videos
- MATRICESBOOK - CENGAGE ENGLISHCHAPTER - MATRICESEXERCISE - Matrix Type
5 Videos
- MATRICESBOOK - CENGAGE ENGLISHCHAPTER - MATRICESEXERCISE - Exercises
65 Videos
- MATHMETICAL REASONINGBOOK - CENGAGE ENGLISHCHAPTER - MATHMETICAL REASONINGEXERCISE - Archives
10 Videos
- METHODS OF DIFFERENTIATIONBOOK - CENGAGE ENGLISHCHAPTER - METHODS OF DIFFERENTIATIONEXERCISE - Single Correct Answer Type
46 Videos
Similar Questions
Let M be a 2×2 symmetric matrix with integer entries. Then M is invertible if The first column of M is the transpose of the second row of M The second row of M is the transpose of the first column of M M is a diagonal matrix with non-zero entries in the main diagonal The product of entries in the main diagonal of M is not the square of an integer
View SolutionIf the order of matrix A is m×n, then the order of the transpose of matrix A is :
View Solution
Knowledge Check
Let A be a symmetric matrix, then Am,m∈N is a
ASingular matrixBNull matrixCSymmetric matrixDUnit matrixLet P stand for the product of the first 5 positive integer. What is the greatest possible value of m if P10m is an integer?
A1B2C3D5
In a 4×4 matrix the sum of each row, column and both the main diagonals is α. Then the sum of the four corner elements
View SolutionIf the side of a square is 4 m and it is converted into a rhombus whose major diagonal is 6 m, find the other diagonal and the area of the rhombus.
View SolutionThe diagonal of a square is 15 m, find the length of its one side and perimeter.
View SolutionABCD is a rhombus whose diagonals intersect at O. If AB=10cm, diagonals BD=16 cm , find the length of diagonal AC
View SolutionThe number of matrices X with entries {0,2,3} for which the sum of all the principal diagonal elements of X.XT is 28 (where XT is the transpose matrix of X), is
View SolutionMatch the corresponding entries of column 1 with column 2. [where m is the magnification produced by the mirror

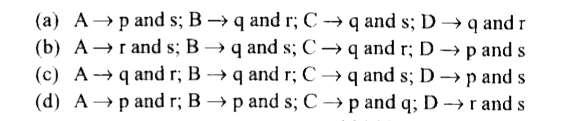
View Solution
