How many different diagonal matrices of order n can be formed which are involuntary ?
The correct Answer is:2n
To find how many different diagonal matrices of order n can be formed which are involutory, we will follow these steps:
Step 1: Understanding Diagonal Matrices
A diagonal matrix is a square matrix where all the elements outside the main diagonal are zero. For a diagonal matrix of order n, it can be represented as:
A=⎛⎜
⎜
⎜
⎜
⎜
⎜
⎜⎝a100⋯00a20⋯000a3⋯0⋮⋮⋮⋱⋮000⋯an⎞⎟
⎟
⎟
⎟
⎟
⎟
⎟⎠
where a1,a2,…,an are the diagonal elements.
Step 2: Involutory Matrices
A matrix A is said to be involutory if A2=I, where I is the identity matrix. For diagonal matrices, squaring the matrix results in squaring each of the diagonal elements:
A2=⎛⎜
⎜
⎜
⎜
⎜
⎜
⎜
⎜⎝a2100⋯00a220⋯000a23⋯0⋮⋮⋮⋱⋮000⋯a2n⎞⎟
⎟
⎟
⎟
⎟
⎟
⎟
⎟⎠
Step 3: Setting Up the Equation
Since A2=I, we have:
A2=⎛⎜
⎜
⎜
⎜
⎜
⎜
⎜⎝100⋯0010⋯0001⋯0⋮⋮⋮⋱⋮000⋯1⎞⎟
⎟
⎟
⎟
⎟
⎟
⎟⎠
This implies:
a21=1,a22=1,…,a2n=1
Step 4: Finding Possible Values for Diagonal Elements
The equation a2i=1 has two solutions for each ai:
ai=1orai=−1
for i=1,2,…,n.
Step 5: Counting the Combinations
Since each diagonal element ai can independently take on one of two values (1 or -1), the total number of different diagonal matrices of order n that can be formed is:
2×2×…×2(n times)=2n
Conclusion
Thus, the number of different involutory diagonal matrices of order n is:
2n
A diagonal matrix is a square matrix where all the elements outside the main diagonal are zero. For a diagonal matrix of order n, it can be represented as:
A=⎛⎜
⎜
⎜
⎜
⎜
⎜
⎜⎝a100⋯00a20⋯000a3⋯0⋮⋮⋮⋱⋮000⋯an⎞⎟
⎟
⎟
⎟
⎟
⎟
⎟⎠
where a1,a2,…,an are the diagonal elements.
A matrix A is said to be involutory if A2=I, where I is the identity matrix. For diagonal matrices, squaring the matrix results in squaring each of the diagonal elements:
A2=⎛⎜
⎜
⎜
⎜
⎜
⎜
⎜
⎜⎝a2100⋯00a220⋯000a23⋯0⋮⋮⋮⋱⋮000⋯a2n⎞⎟
⎟
⎟
⎟
⎟
⎟
⎟
⎟⎠
Since A2=I, we have:
A2=⎛⎜
⎜
⎜
⎜
⎜
⎜
⎜⎝100⋯0010⋯0001⋯0⋮⋮⋮⋱⋮000⋯1⎞⎟
⎟
⎟
⎟
⎟
⎟
⎟⎠
This implies:
a21=1,a22=1,…,a2n=1
The equation a2i=1 has two solutions for each ai:
ai=1orai=−1
for i=1,2,…,n.
Since each diagonal element ai can independently take on one of two values (1 or -1), the total number of different diagonal matrices of order n that can be formed is:
2×2×…×2(n times)=2n
Thus, the number of different involutory diagonal matrices of order n is:
2n
Topper's Solved these Questions
- MATRICESBOOK - CENGAGE ENGLISHCHAPTER - MATRICESEXERCISE - CAE 13.5
17 Videos
- MATRICESBOOK - CENGAGE ENGLISHCHAPTER - MATRICESEXERCISE - Exercises
65 Videos
- MATRICESBOOK - CENGAGE ENGLISHCHAPTER - MATRICESEXERCISE - CAE 13.3
10 Videos
- MATHMETICAL REASONINGBOOK - CENGAGE ENGLISHCHAPTER - MATHMETICAL REASONINGEXERCISE - Archives
10 Videos
- METHODS OF DIFFERENTIATIONBOOK - CENGAGE ENGLISHCHAPTER - METHODS OF DIFFERENTIATIONEXERCISE - Single Correct Answer Type
46 Videos
Similar Questions
Matrices of any order can be added.
View SolutionHow many 9- digits numbers of different digits can be formed?
View SolutionHow many different numbers of three digits can be formed with the digits 1,2,3,4,5 no digit is being repeated ?
View SolutionHow many different 4-digit number can be formed with the digits 1,2,3,4 without any repetition ? Also find their sum.
View SolutionFind the number of idempotent diagonal matrices of order n.
View Solution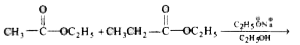
How many different condensation products would be formed by above reaction?
View SolutionHow many different words of 4 letters can be formed with the letters of the word EXAMINATION?
View SolutionHow many different numbers of six digits can be formed with the digits 3,1,7,0,9, 5 ?
View SolutionIf none of the digits 3,5,7,8,9 be repeated, how many different numbers greater than 7000 can be formed with them?
View SolutionHow many different numbers of 4 digits each can be formed with the ten digits 0,1,2,…9 when digits are not repeated?
View Solution
