A straight line L through the point (3,-2) is inclined at an angle $60^{\circ}$ to the line $\sqrt{3} x + y = 1$ If L also intersects the x-axis then the equation of L is

A

$y + \sqrt{3} x + 2 - 3 \sqrt{3} = 0$

B

$y - \sqrt{3} x + 2 + 3 \sqrt{3} = 0$

C

$\sqrt{3} y - x + 3 + 2 \sqrt{3} = 0$

D

$\sqrt{3} y + x - 3 + 2 \sqrt{3} = 0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

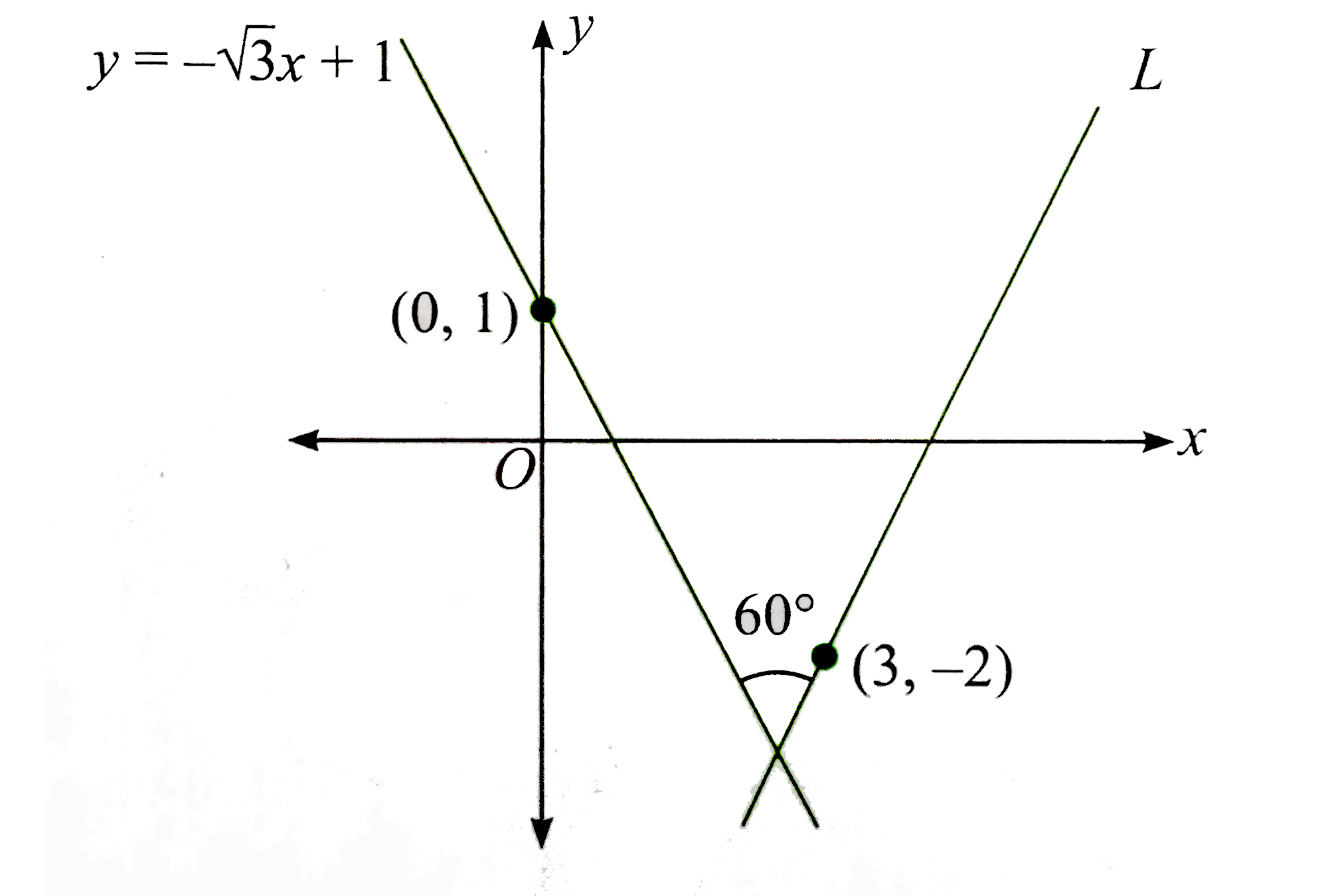
Let the slope of the required line be m. Then
$∣ ∣ ∣ \frac{m + \sqrt{3}}{1 - \sqrt{3} m} ∣ ∣ ∣ = \sqrt{3}$
$∴ m + \sqrt{3} = \pm (\sqrt{3} - 3 m)$
$∴ m = 0 or m = \sqrt{3}$
Therefore, the equation is
$y + 2 = \sqrt{3} (x - 3) (m \neq 0 as given that line cuts the x-axis)$
$or \sqrt{3} x - y - (2 + 3 \sqrt{3}) = 0$

Updated on:21/07/2023

|

Class 12 MATHS STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Find the equation of straight lines through the point A(3,-2) and inclined at $60^{\circ}$ to the line $\sqrt{3} x + y = 1$ .
A $y + \sqrt{3} x + 2 - 3 \sqrt{3} = 0$
B $y - \sqrt{3} x + 2 + 3 \sqrt{3} = 0$
C $\sqrt{3} y - x + 3 + 2 \sqrt{3} = 0$
D $\sqrt{3} y + x - 3 + 2 \sqrt{3} = 0$

CENGAGE ENGLISH-STRAIGHT LINES-ARCHIVES (JEE ADVANCED)

Home
Ask Doubt
Profile