The line 3x+2y=24 meets the y-axis at A and the x-axis at B. The perpendicular bisector of AB meets the line through (0,−1) parallel to the x-axis at C. If the area of triangle ABC is A , then the value of A13 is________
The correct Answer is:91
To solve the problem step by step, we will follow the instructions given in the video transcript and derive the required values systematically.
Step 1: Find the Points A and B
The line given is 3x+2y=24.
Finding Point A (y-intercept):
To find where the line meets the y-axis, set x=0:
3(0)+2y=24⟹2y=24⟹y=12
Thus, point A is (0,12).
Finding Point B (x-intercept):
To find where the line meets the x-axis, set y=0:
3x+2(0)=24⟹3x=24⟹x=8
Thus, point B is (8,0).
Step 2: Find the Midpoint of AB
The midpoint M of segment AB can be calculated using the midpoint formula:
M=(x1+x22,y1+y22)=(0+82,12+02)=(4,6)
Step 3: Find the Slope of AB
The slope m of line segment AB is given by:
m=y2−y1x2−x1=0−128−0=−128=−32
Step 4: Find the Slope of the Perpendicular Bisector
The slope of the perpendicular bisector is the negative reciprocal of the slope of AB:
mperpendicular=−1m=23
Step 5: Write the Equation of the Perpendicular Bisector
Using point-slope form y−y1=m(x−x1) with point M(4,6):
y−6=23(x−4)
Expanding this:
y−6=23x−83⟹y=23x+103
Step 6: Find Point C
Point C lies on the line y=−1 (a horizontal line through (0,−1)). Set y=0 in the equation of the perpendicular bisector:
0=23x+103
Solving for x:
23x=−103⟹x=−5
Thus, point C is (−5,0).
Step 7: Calculate the Area of Triangle ABC
The area A of triangle ABC can be calculated using the formula:
A=12|x1(y2−y3)+x2(y3−y1)+x3(y1−y2)|
Substituting the coordinates A(0,12), B(8,0), and C(−5,0):
A=12|0(0−0)+8(0−12)+(−5)(12−0)|
=12|0−96−60|=12|−156|=1562=78
Step 8: Find the Value of A13
Now, we need to find:
A13=7813=6
Final Answer
Thus, the value of A13 is 6.
---
The line given is 3x+2y=24.
To find where the line meets the y-axis, set x=0:
3(0)+2y=24⟹2y=24⟹y=12
Thus, point A is (0,12).
To find where the line meets the x-axis, set y=0:
3x+2(0)=24⟹3x=24⟹x=8
Thus, point B is (8,0).
The midpoint M of segment AB can be calculated using the midpoint formula:
M=(x1+x22,y1+y22)=(0+82,12+02)=(4,6)
The slope m of line segment AB is given by:
m=y2−y1x2−x1=0−128−0=−128=−32
The slope of the perpendicular bisector is the negative reciprocal of the slope of AB:
mperpendicular=−1m=23
Using point-slope form y−y1=m(x−x1) with point M(4,6):
y−6=23(x−4)
Expanding this:
y−6=23x−83⟹y=23x+103
Point C lies on the line y=−1 (a horizontal line through (0,−1)). Set y=0 in the equation of the perpendicular bisector:
0=23x+103
Solving for x:
23x=−103⟹x=−5
Thus, point C is (−5,0).
The area A of triangle ABC can be calculated using the formula:
A=12|x1(y2−y3)+x2(y3−y1)+x3(y1−y2)|
Substituting the coordinates A(0,12), B(8,0), and C(−5,0):
A=12|0(0−0)+8(0−12)+(−5)(12−0)|
=12|0−96−60|=12|−156|=1562=78
Now, we need to find:
A13=7813=6
Thus, the value of A13 is 6.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (JEE MAIN)
9 Videos
- STRAIGHT LINESBOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (JEE ADVANCED)
3 Videos
- STRAIGHT LINESBOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MATRIX MATCH TYPE)
8 Videos
- STRAIGHT LINEBOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGE ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )
1 Video
Similar Questions
The straight line 2x+3y=24 meets the x-axis at P and the y-axis at Q. The perpendicular bisector of PQ meets the line through (-2,0) parallel to the y-axis at R. Find the area of the ΔPQR.
View SolutionStatement -1 : The line 3x + 2y = 24 meets the coordinates axes at A and B , and the perpendicular bisector of AB meets the line through (0,-1) parallel to the x-axis at C . The area of Δ ABC is 91 square units .
Statement-2 : Area of the triangle with vertices at (a,0) , (0,b) and (a , b) is ab/2 sq. units .
View Solution
Knowledge Check
The line xa−yb=1 cuts the x-axis at P. The equation of the line through P and perpendicular to the given line is
A(a) x+y=abB(b) x+y=a+bC(c) ax+by=a2D(d) bx+ay=b2
The line 3x - 4y + 12 = 0 meets x-axis at point A and y-axis at point B. Find :
equation of perpendicular bisector of line segment AB
View SolutionThe line 3x - 4y + 12 = 0 meets x-axis at point A and y-axis at point B. Find :
the co-ordinates of A and B.
View SolutionThe line 2x+3y=12 meets the x-axis at A and y-axis at B. The line through (5,5) perpendicular to AB meets the x-axis and the line AB at C and E respectively. If O is the origin of coordinates, find the area of figure OCEB.
View SolutionThe line xa+yb=1 meets the axis of and y at A and B respectively and C is middle point of AB then
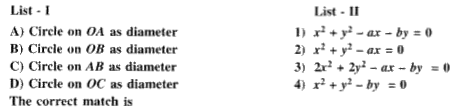
View SolutionThe line 4x + 5y + 20 = 0 meets x-axis at point A and y-axis at point B. Find :
the co-ordinates of points A and B.
View SolutionFind the equation of a line drawn perpendicular to the line x4+y6=1 through the point where it meets the y axis.
View SolutionFind the equation of a line drawn perpendicular to the line x4+y6=1 through the point where it meets the y axis.
View Solution
