Question

The equation of the bisector of the acute angle between the lines $2x-y+4=0$
and $x-2y=1$
is
$x-y+5=0$

$x-y+1=0$

$x-y=5$
(d) none of
these

Options

A. x+y+5=0
B. x-y+1=0
C. x-y=5
D. none of these

Answer

B

Explanation

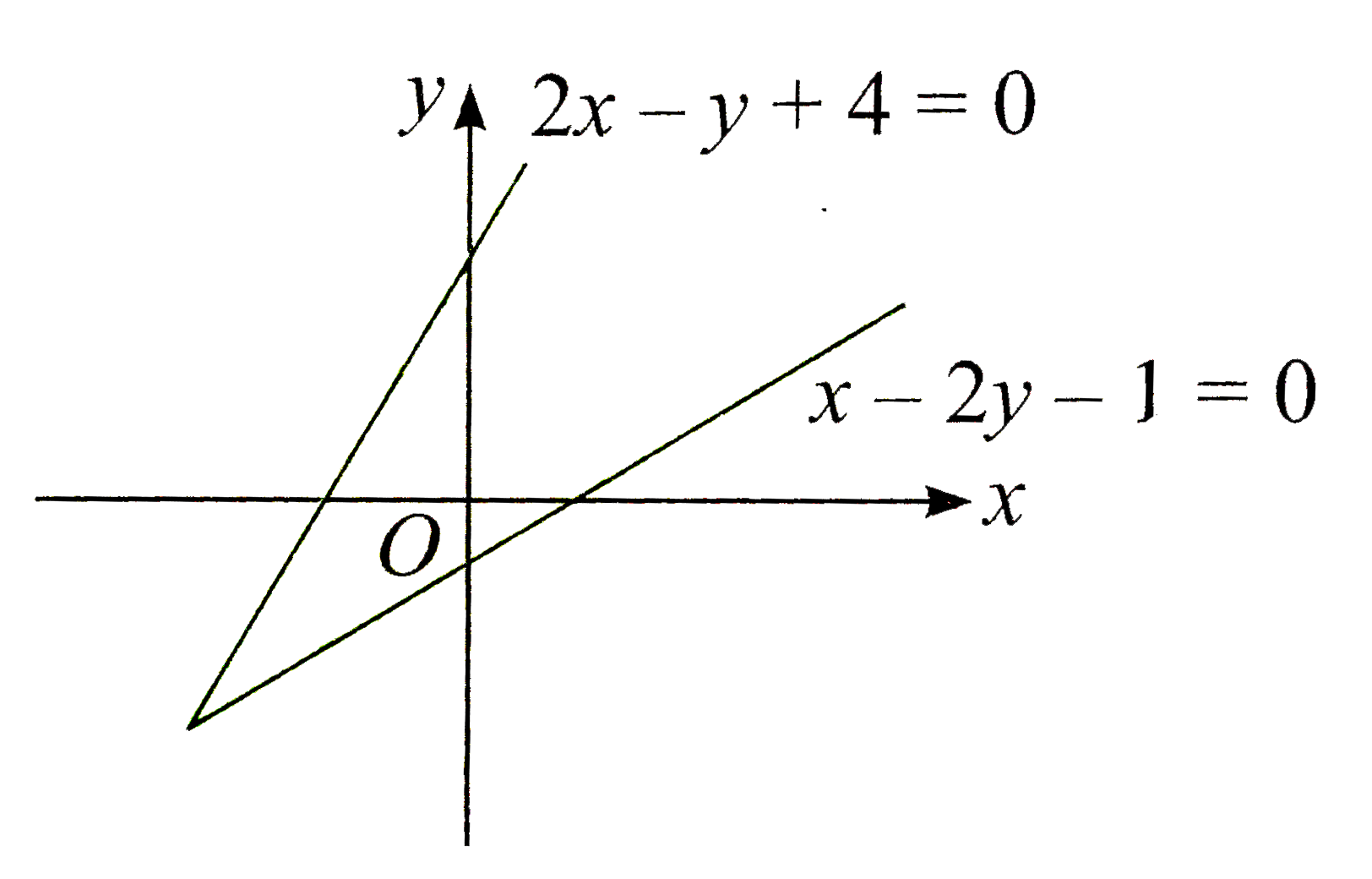
Clearly, from the figure, the origin is contained in the acute angle. Writing the equations of thel lines as 2x-y+4=0 and -x+2y+1=0, the required bisector is
`(2x-y+4)/(sqrt(5)) = (-x+2y+1)/(sqrt(5))`