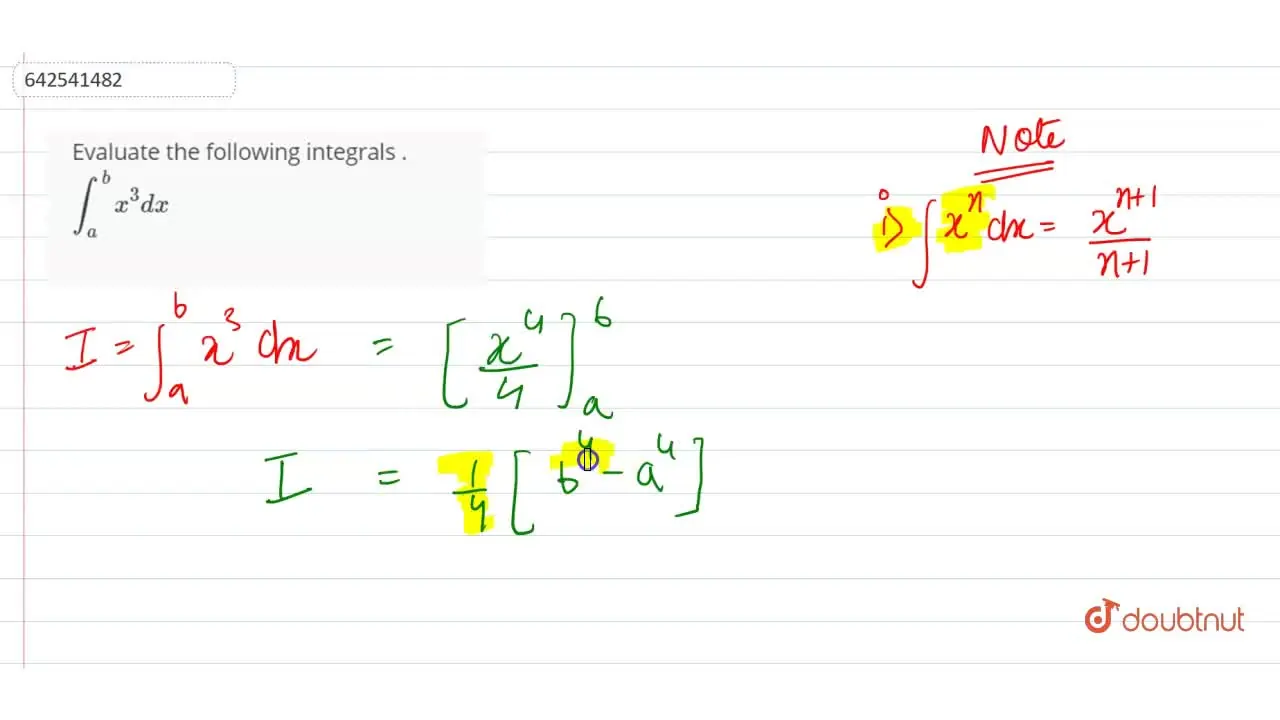
Evaluate the following integrals .
∫bax3dx
Video Solution
Text Solution
The correct Answer is:14(b4−a4)
Here f(x)=x3
∫bax3dx=limn→∞hn−1∑r=0(a+rh)3, where nh=b−a
=limn→∞[nha3+3a2h2n−1∑r=1r+3ah3n−1∑r=1r2+h4n−1∑r=1r3]
=limn→∞[nha3+3a2h2{(n−1)n2}+3ah3{n(n−1)(2n−1)6}+h4{(n−1)2n24}]
=limh→∞[(nh)a3+3a2{(nh−h)(nh)2}
+3a{(nh)(nh−h)(2nh−h)6}
+{(nh−h)2(nh)24}]
=[(b−a)3+3a2{(b−a−0)(b−a)2}
+3a{(b−a)(b−a−0)(2(b−a)−0)6}
+{(b−a−0)2(b−a)24}]
[∵asn→∞,h→0,nh→b−a]
=14(b−a)[4a3+6a3(b−a)+4a(b−a)2+(b−a)3]
=14(b−a)(a3+a2b+ab2+b3)
=14(b−a)(b+a)(b2+a2)=14(b4−a4)
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - DEFINITE INTEGRATION EXERCISE - SCQ_TYPE113videos
DEFINITE INTEGRATION
- BOOK - CENGAGE ENGLISHCHAPTER - DEFINITE INTEGRATION EXERCISE - MCQ_TYPE27videos
DEFINITE INTEGRATION
- BOOK - CENGAGE ENGLISHCHAPTER - DEFINITE INTEGRATION EXERCISE - SOLVED EXAMPLE_TYPE20videos
DEFINITE INTEGRATION
- BOOK - CENGAGE ENGLISHCHAPTER - CURVE TRACINGEXERCISE - EXERCISES24videos
CURVE TRACING
- BOOK - CENGAGE ENGLISHCHAPTER - DETERMINANTEXERCISE - Multiple Correct Answer5videos
DETERMINANT
Similar Questions
Evaluate the following integrals : ∫20(x+3)dx
View SolutionEvaluate the following integrals : ∫1−1(x+3)dx
View SolutionEvaluate the following integrals ∫31(2x+3)dx
View SolutionEvaluate the following integrals : ∫(3x+4)2dx
View SolutionEvaluate the following Integrals :
∫dx1+x3View SolutionEvaluate the following integrals : ∫20(x2+x)dx
View SolutionEvaluate the following integral: ∫150[x2]dx
View SolutionEvaluate the following Integrals.
∫x2axdxView SolutionEvaluate the following integral: ∫3−3|x+1|dx
View SolutionEvaluate the following integrals
∫√a−xx−bdxView Solution