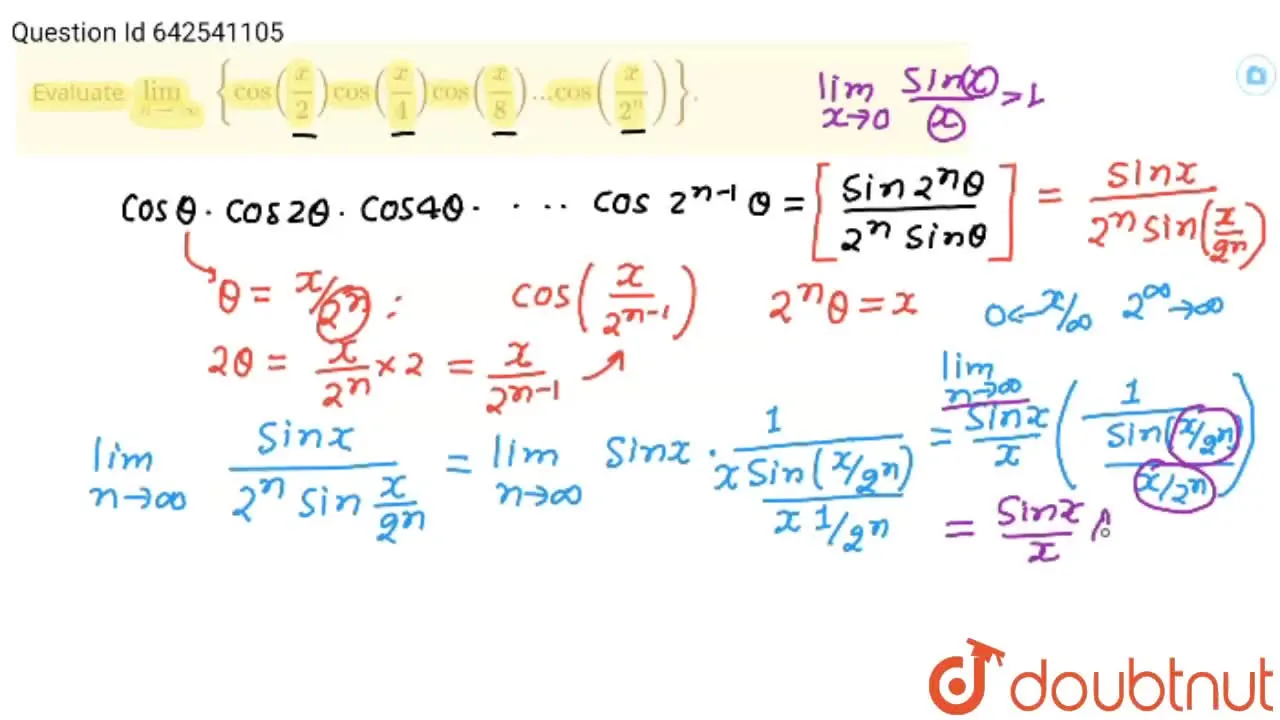
Evaluate limn→∞{cos(x2)cos(x4)cos(x8)...cos(x2n)}.
Video Solution
Text Solution
We know that
cosθcos2θcos4θ...cos2n−1θ=sin2nθ2nsinθ
∴ cos(x2)cos(x4)cos(x8)...cos(x2n)=sinx2nsin(x2n)
∴ Given limits is limn→∞sinx2nsin(x2n)=limn→∞sinxx(x2n)sin(x2n)
=sinxx
|
Updated on:21/07/2023
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - LIMITSEXERCISE - EXERCISE 2.110videos
LIMITS
- BOOK - CENGAGE ENGLISHCHAPTER - LIMITSEXERCISE - EXERCISE 2.27videos
LIMITS
- BOOK - CENGAGE ENGLISHCHAPTER - LIMITSEXERCISE - Comprehension Type4videos
LIMITS
- BOOK - CENGAGE ENGLISHCHAPTER - JEE 2019EXERCISE - Chapter 109videos
JEE 2019
- BOOK - CENGAGE ENGLISHCHAPTER - LINEAR COMBINATION OF VECTORS, DEPENDENT AND INDEPENDENT VECTORSEXERCISE - DPP 1.210videos
LINEAR COMBINATION OF VECTORS, DEPENDENT AND INDEPENDENT VECTORS
Similar Questions
Evaluate limx→01−cos2xx2
View SolutionEvaluate (lim)n→∞{cos(x2)cos(x4)cos(x8)...cos(x2n)}
View SolutionThe value of limn→∞(cos.x2cos.x4cos.x8………cos.x2n+1) is equal to
View SolutionWe have
f(x)limn→∞cos(x)2cos(x)22cos(x)23cos(x)24……
….cos(x)2n=sinx2nsinx2n
using the identity
limn→∞limx→0 f(x) equalsView SolutionEvaluate: limx→01−cos2xx2
View SolutionEvaluate :
limx→0ecosx−1cosxView Solution8⋅sin(x8)⋅cos(x2)⋅cos(x4)⋅cos(x8)=
View SolutionEvaluate: (lim)x→08x8{1−cos(x22)−cos(x24)+cos(x22)cos(x24)}
View SolutionEvaluate limx→π4cosx−sinxcos2x
View Solutionlimx→0cos2x−1cosx−1
View Solution