Question

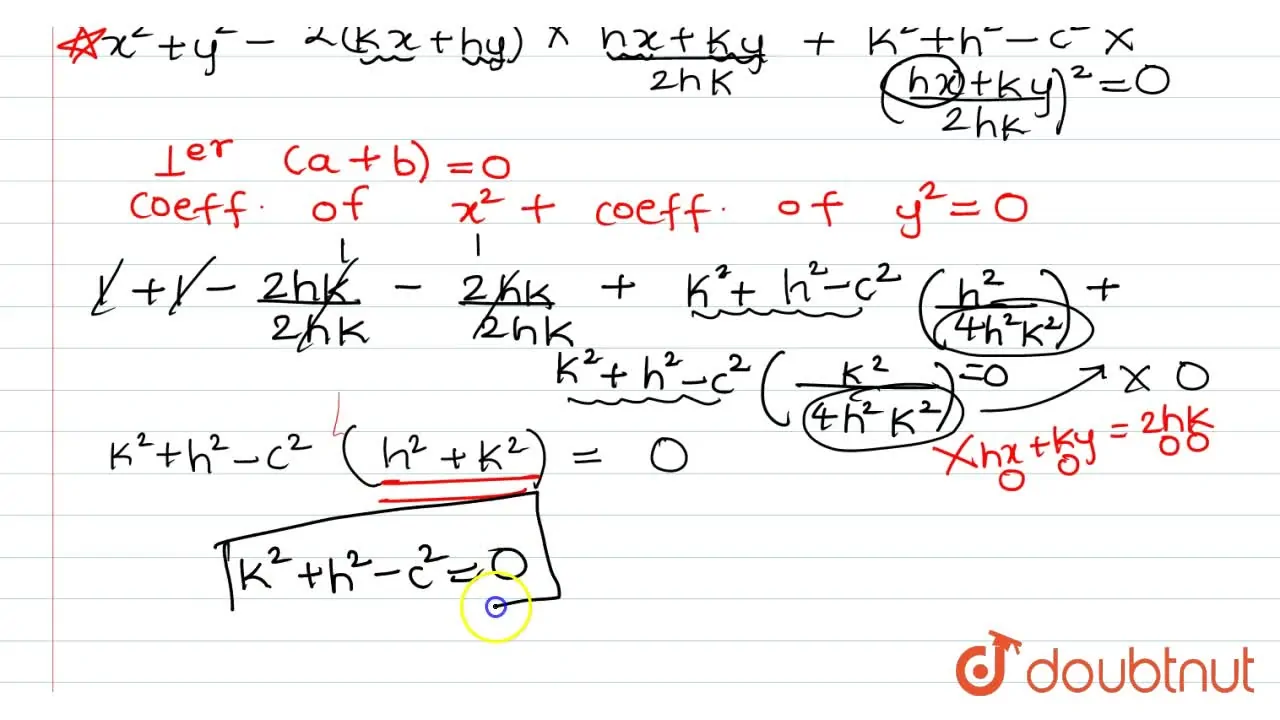
Prove that the straight lines joining the origin to the points of intersection of the straight line $hx+ky=2hk$ and the curve ${(x-k)}^2+{(y-h)}^2=c^2$ are at right angle if $h^2+k^2=c^2$.

Explanation

To prove that the straight lines joining the origin to the points of intersection of the straight line $$hx+ky=2hk$$ and the curve $$(x-k{)}^2+(y-h{)}^2=c^2$$ are at right angles if $$h^2+k^2=c^2$$, we can follow these steps:

Step 1: Write the equations
We have the straight line:
\( hx + ky = 2hk \)
And the circle (curve):
\( (x - k)^2 + (y - h)^2 = c^2 \)

Step 2: Rearrange the line equation
Rearranging the line equation gives:
\( hx + ky - 2hk = 0 \)
This can be expressed as:
\( y = -\frac{h}{k}x + 2h \)

Step 3: Substitute \( y \) in the circle equation
Substituting \( y \) from the line equation into the circle equation:
\( (x - k)^2 + \left(-\frac{h}{k}x + 2h - h\right)^2 = c^2 \)
This simplifies to:
\( (x - k)^2 + \left(-\frac{h}{k}x + h\right)^2 = c^2 \)

Step 4: Expand the equation
Expanding both terms:
\( (x - k)^2 = x^2 - 2kx + k^2 \)
\( \left(-\frac{h}{k}x + h\right)^2 = \frac{h^2}{k^2}x^2 - 2hx + h^2 \)
Combining these gives:
\( x^2 - 2kx + k^2 + \frac{h^2}{k^2}x^2 - 2hx + h^2 = c^2 \)

Step 5: Combine like terms
Combining the \( x^2 \) terms:
\( \left(1 + \frac{h^2}{k^2}\right)x^2 - (2k + 2h)x + (k^2 + h^2 - c^2) = 0 \)

Step 6: Use the condition \( h^2 + k^2 = c^2 \)
Substituting \( h^2 + k^2 = c^2 \):
\( \left(1 + \frac{h^2}{k^2}\right)x^2 - (2k + 2h)x + 0 = 0 \)
This indicates that the quadratic equation has roots, which correspond to the points of intersection.

Step 7: Find the slopes of the lines
The slopes of the lines from the origin to the points of intersection can be calculated. If the points of intersection are \( (x_1, y_1) \) and \( (x_2, y_2) \), the slopes are:
\( m_1 = \frac{y_1}{x_1}, \quad m_2 = \frac{y_2}{x_2} \)

Step 8: Show that the product of slopes is -1
For the lines to be perpendicular, the product of their slopes must equal -1:
\( m_1 \cdot m_2 = -1 \)

Conclusion
Thus, we have shown that the lines joining the origin to the points of intersection of the given straight line and curve are at right angles if \( h^2 + k^2 = c^2 \).

---