Question

The following Venn diagram shows three events, A, B, and C, and also the probabilities of the various intersections.
Determine
(a) P(A)
(b) $P(B\cap \overline{C})$
(c ) $P(A\cup B)$
(d) $P(A\cap \overline{B})$
(e) $P(B\cap C)$
(f) Probability of the event that exactly one of A, B, and C occurs.

Explanation

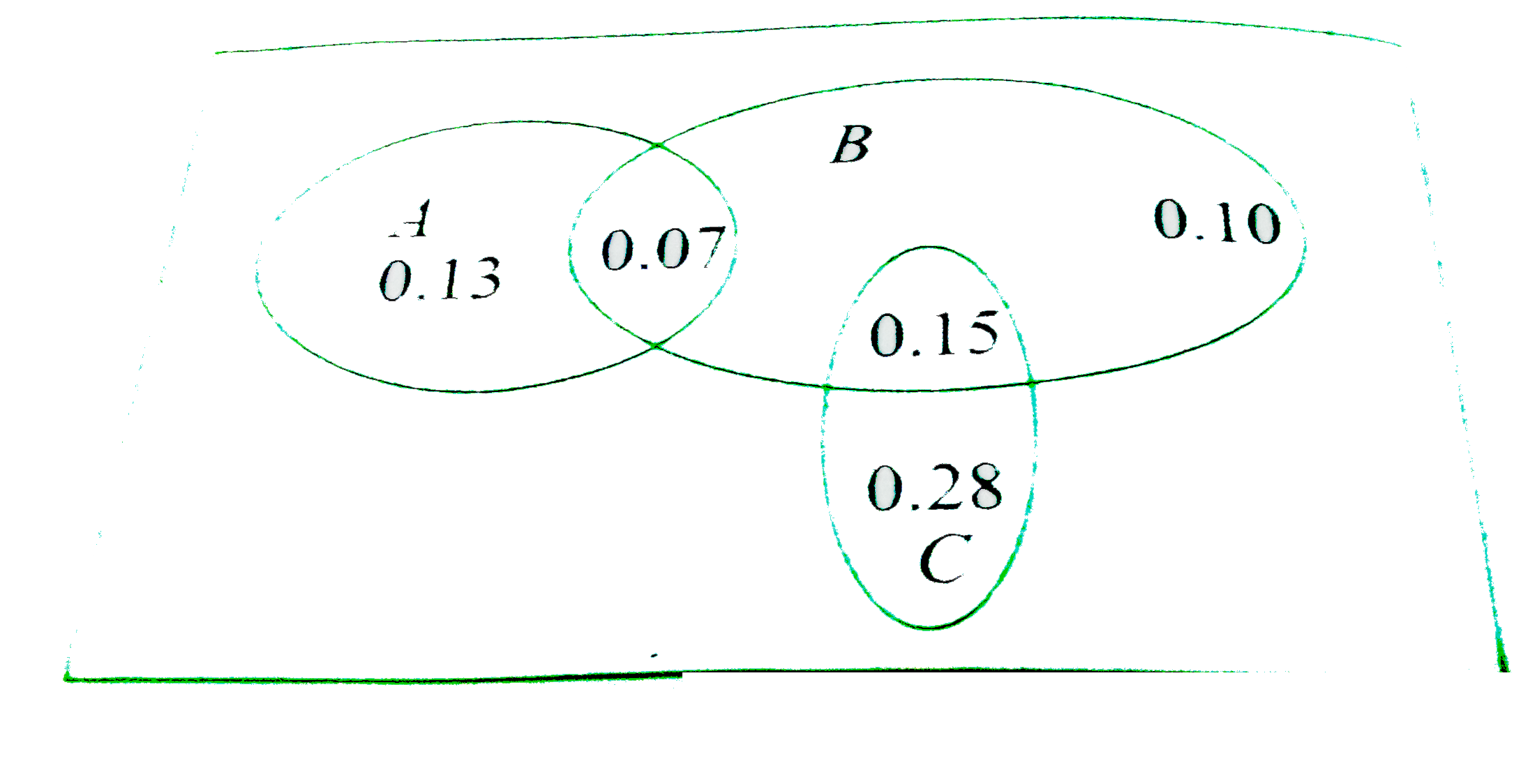
From the above Venn diagram,
(a) `P(A) = 0.13 + 0.07 = 0.20`
(b) `P(B nn barC) = P(B) - P(B nn C)`
= 0.07 + 0.10 + 0.15 - 0.15 = 0.17
(c ) `P(A uu B) = P(A) + P(B) - P(A nn B)`
`= 0.13 + 0.07 + 0.07 + 0.10 + 0.15 - 0.07 = 0.45`
(d) `P(A nn barB) = P(A) - P(A nn B) = 0.13 + 0.07 - 0.07 = 0.13`
(e) `P(B nn C) = 0//15`
(f) P (exactly one of the three occurs) = 0.13 + 0.10 + 0.28 = 0.51