Four candidates A, B, C, D have applied for the assignment ot coach a school cricket team. If A is twice as likely to be selected as B, and B and C are given about the same chance of being selected, while C is twice as likely to be selected as D, what are the probability that (i) C will be selected ? (ii) A will not be selected?
To solve the problem step-by-step, we will define the probabilities of each candidate based on the information provided in the question.
Step 1: Define the probabilities
Let:
- P(A) = probability of A being selected
- P(B) = probability of B being selected
- P(C) = probability of C being selected
- P(D) = probability of D being selected
Step 2: Set up the relationships based on the problem statement
From the problem, we have the following relationships:
1. A is twice as likely to be selected as B:
P(A)=2P(B)
2. B and C are given about the same chance of being selected:
P(B)=P(C)
3. C is twice as likely to be selected as D:
P(C)=2P(D)
Step 3: Express all probabilities in terms of P(D)
From the third relationship, we can express P(C) in terms of P(D):
P(C)=2P(D)
Using the second relationship, we can express P(B):
P(B)=P(C)=2P(D)
Now substituting P(B) into the first relationship gives:
P(A)=2P(B)=2(2P(D))=4P(D)
Step 4: Write the total probability equation
The total probability must equal 1:
P(A)+P(B)+P(C)+P(D)=1
Substituting our expressions for P(A), P(B), and P(C):
4P(D)+2P(D)+2P(D)+P(D)=1
Step 5: Simplify the equation
Combining the terms gives:
4P(D)+2P(D)+2P(D)+P(D)=9P(D)=1
Step 6: Solve for P(D)
Now, solving for P(D):
P(D)=19
Step 7: Calculate the probabilities for A, B, and C
Now we can use P(D) to find the other probabilities:
- P(A)=4P(D)=4×19=49
- P(B)=2P(D)=2×19=29
- P(C)=2P(D)=2×19=29
Step 8: Answer the questions
(i) The probability that C will be selected:
P(C)=29
(ii) The probability that A will not be selected:
P(A not selected)=1−P(A)=1−49=59
Final Answers
- (i) The probability that C will be selected is 29.
- (ii) The probability that A will not be selected is 59.
Let:
- P(A) = probability of A being selected
- P(B) = probability of B being selected
- P(C) = probability of C being selected
- P(D) = probability of D being selected
From the problem, we have the following relationships:
1. A is twice as likely to be selected as B:
P(A)=2P(B)
2. B and C are given about the same chance of being selected:
P(B)=P(C)
3. C is twice as likely to be selected as D:
P(C)=2P(D)
From the third relationship, we can express P(C) in terms of P(D):
P(C)=2P(D)
P(B)=P(C)=2P(D)
P(A)=2P(B)=2(2P(D))=4P(D)
The total probability must equal 1:
P(A)+P(B)+P(C)+P(D)=1
4P(D)+2P(D)+2P(D)+P(D)=1
Combining the terms gives:
4P(D)+2P(D)+2P(D)+P(D)=9P(D)=1
Now, solving for P(D):
P(D)=19
Now we can use P(D) to find the other probabilities:
- P(A)=4P(D)=4×19=49
- P(B)=2P(D)=2×19=29
- P(C)=2P(D)=2×19=29
(i) The probability that C will be selected:
P(C)=29
P(A not selected)=1−P(A)=1−49=59
- (i) The probability that C will be selected is 29.
- (ii) The probability that A will not be selected is 59.
Topper's Solved these Questions
- PROBABILITY I BOOK - CENGAGE ENGLISHCHAPTER - PROBABILITY I EXERCISE - Solved Example
9 Videos
- PROBABILITY I BOOK - CENGAGE ENGLISHCHAPTER - PROBABILITY I EXERCISE - Exercise 9.1
6 Videos
- PROBABILITYBOOK - CENGAGE ENGLISHCHAPTER - PROBABILITYEXERCISE - Comprehension
2 Videos
- PROBABILITY IIBOOK - CENGAGE ENGLISHCHAPTER - PROBABILITY IIEXERCISE - MULTIPLE CORRECT ANSWER TYPE
6 Videos
Similar Questions
Three students A,BandC are in a swimming race. AandB have the same probability of winning and each is twice as likely to win as C. Find the probability that the BorC wins. Assume no two reach the winning point simultaneously.
View SolutionFor a post,three persons A, B and C appearin the interview. The probability of A being selected is twice that of B and the probability of b being selected is thrice that of C. What of the individual probability of A, B and C being selected?
View Solution
Knowledge Check
Identify the parts labelled as A, B, C, D and E in the given diagram of a human sperm and select the correct option.
 AA-Nucleus, B-Tail, C-Mitochondria,
AA-Nucleus, B-Tail, C-Mitochondria,
D-Acrosome, E-CentrioleBA-Acrosome, B-Nucleus,
C-Centriole, D-Mitochondria,
E-Plasma membraneCA-Nucleus, B-Mitochondria,
C-Plasma membrane,
D-Centriole, E-NeckDA-Acrosome, B-Centriole,
C-Mitochondria, B-Centriole,
C-Mitochondria, D-Plasma membrane, E-TailGiven is the diagrammatic sketch of a certain type of connective tissue
Identify the parts labelled as A,B,C and D and select the correct option.
 A A B C D Macrophage Fibroblast Collagen fibres Mast cellB A B C D Mast cell Macrophage Fibroblast Collagen fibreC A B C D Macrophage Collagen fibre Fibroblast Mast cellD A B C D Mast cell Collagen fibre Fibroblast Macrophage
A A B C D Macrophage Fibroblast Collagen fibres Mast cellB A B C D Mast cell Macrophage Fibroblast Collagen fibreC A B C D Macrophage Collagen fibre Fibroblast Mast cellD A B C D Mast cell Collagen fibre Fibroblast Macrophage
A and B are two candidates seeking admission in a college. The probability that A is selected is 0.7 and the probability that exactly one of them is selected is 0.6. Find the probability that B is selected.
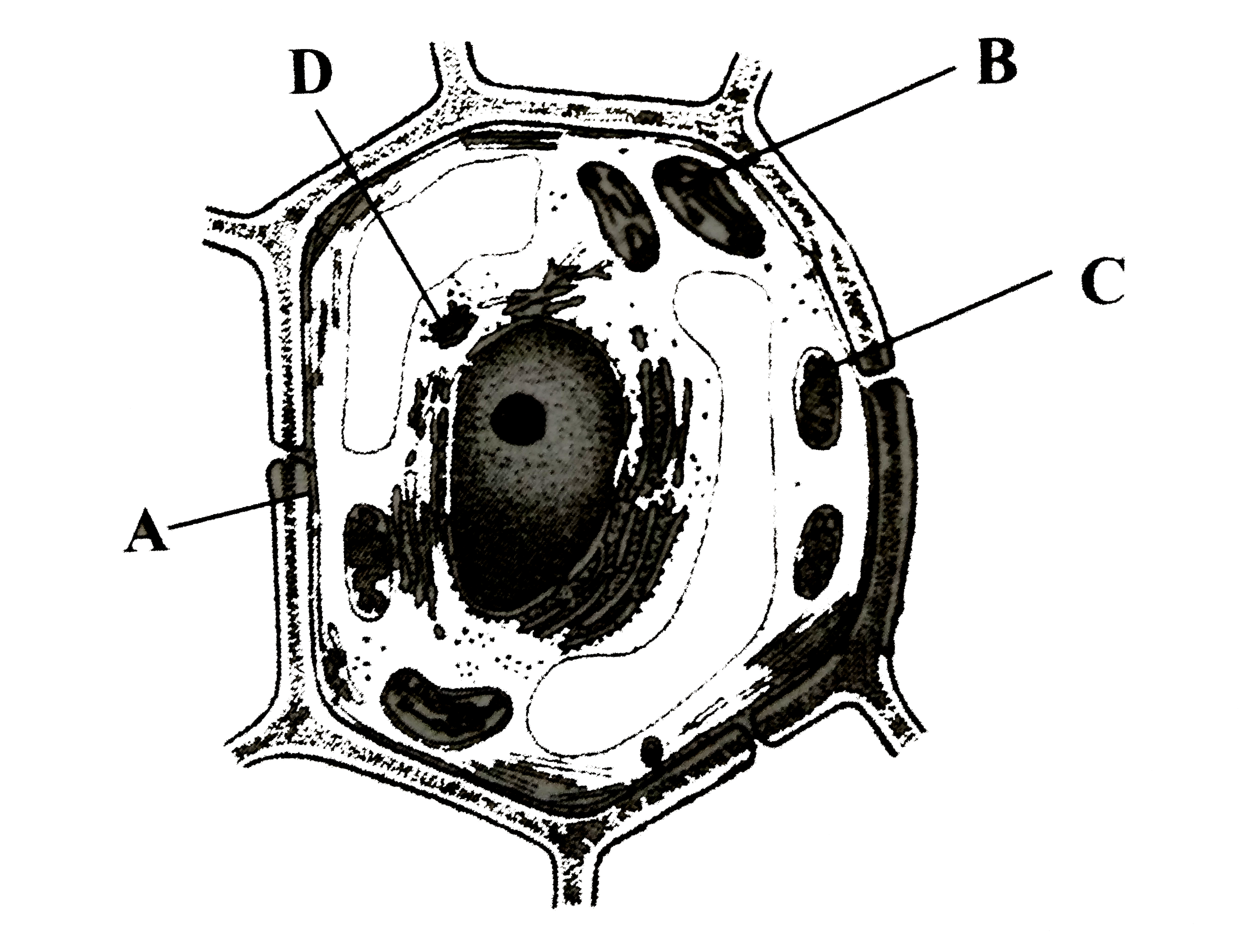
View SolutionIdentify the parts labelled as A, B, C and D in the given ultrastructure of a plant cell and select the correct option.
View SolutionGiven below is the diagram of the transverse section of monocot stem. Identify the parts labelled A, B, C and D and select the right option about the

View SolutionIf A and B are two candidates seeking admission in an engineering college. The probability that A is selected is 0.5 and the probability that both A and B are selected is at most 0.3. Is it possible that the probability of B getting selected is 0.7 ?
View SolutionIdentify the parts labelled as A,B,C and D in the given figure and select the correct option from the given codes.
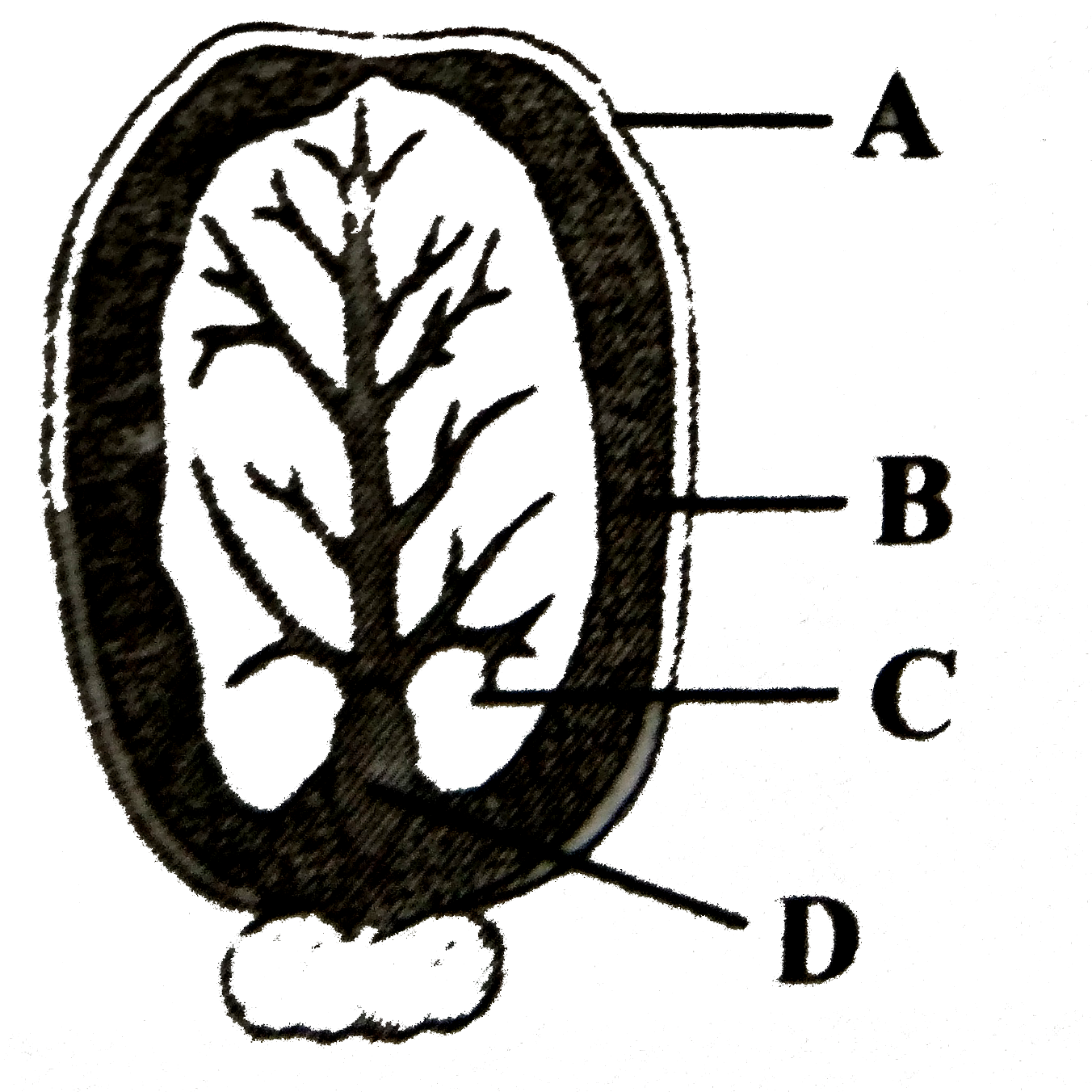
View SolutionGiven is the diagrammatic sketch of a certain type of connective tissue
Identify the parts labelled as A,B,C and D and select the correct option.

View Solution
