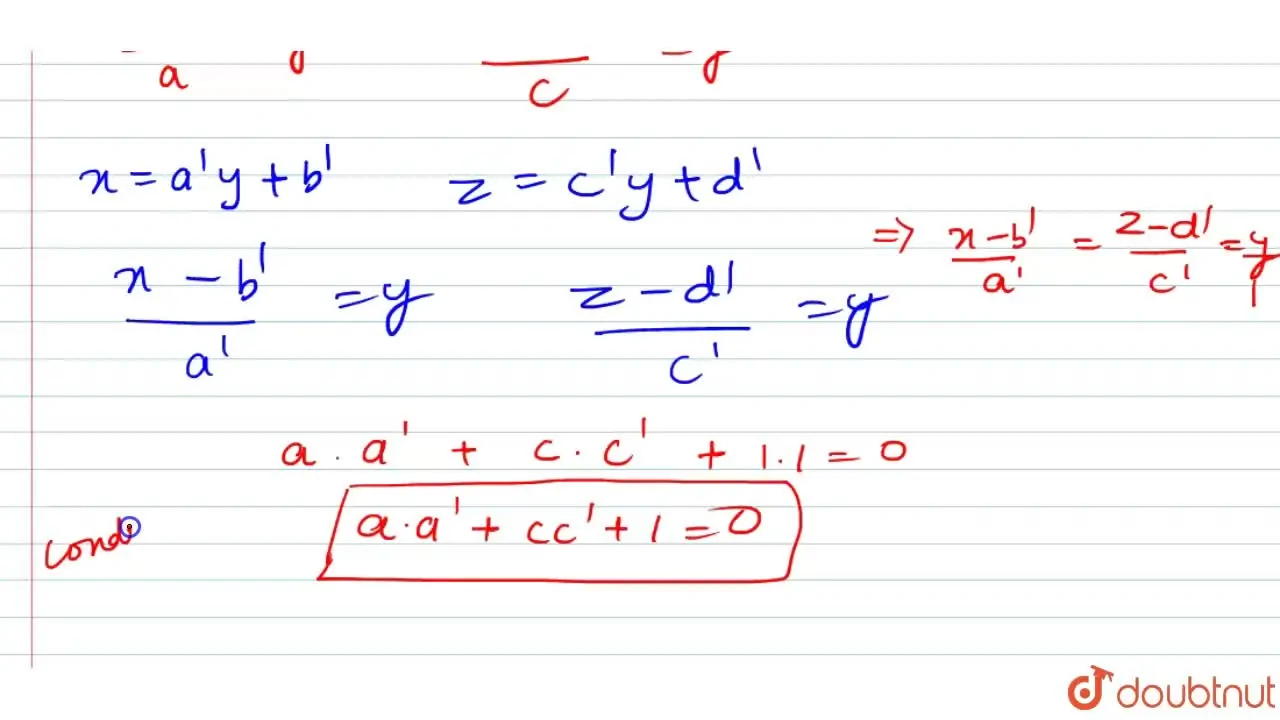
Fid the condition if lines are perpendicular.
The equations of straight lines can be rewritten as
and
The above lines are perpendicular if .
Related Playlists
Similar Questions
Prove that the line x=ay+b, z=cy+d and x=a′y+b′, z=c′y+d′ are perpendicular if aa′ +cc′+ 1=0
View SolutionIf the lines x=a1y+b1,z=c1y+d1 and x=a2y+b2,z=c2y+d2 are perpendicular, then
View SolutionIf the abscissae and the ordinates of two point AandB be the roots of ax2+bx+c=0anda′y2+b′y=c′=0 respectively, show that the equation of the circle described on AB as diameter is aa′(x2+y2)+a′bx+ab′y+(ca′+ca)=0.
View SolutionShow the condition that the curves ax2+by2=1 and a′ x2+b′ y2=1 Should intersect orthogonally
View SolutionThe two lines x=ay+b,z=cy+dandx=a'y+b',z=c'y+d' are pendicular to each other if
View SolutionIf the quadrilateral formed by the lines ax+by+c=0,a′x+b′y+c=0,ax+by+c′=0,a′x+b′y+c′=0 has perpendicular diagonals, then (a) b2+c2=b'2+c'2 (b)c2+a2=c'2+a'2 (c)a2+b2=a'2+b'2 (d) none of these
View SolutionIf the quadrilateral formed by the lines ax+by+c=0,a′x+b′y+c=0,ax+by+c′=0,a′x+b′y+c′=0 has perpendicular diagonals, then b2+c2=b'2+c'2 c2+a2=c'2+a'2 a2+b2=a'2+b'2 (d) none of these
View SolutionLet f be a continuous, differentiable, and bijective function. If the tangent to y=f(x)atx=a is also the normal to y=f(x)atx=b, then there exists at least one c∈(a,b) such that (a)f′(c)=0 (b) f′(c)>0 (c)f′(c)<0 (d) none of these
View SolutionLet f be a continuous, differentiable, and bijective function. If the tangent to y=f(x)atx=a is also the normal to y=f(x)atx=b, then there exists at least one c∈(a,b) such that f′(c)=0 (b) f′(c)>0 f′(c)<0 (d) none of these
View SolutionA parallelepiped S has base points A,B,CandD and upper face points A′,B′,C′,andD' . The parallelepiped is compressed by upper face A'B'C'D' to form a new parallepiped T having upper face points A'',B'',C''and D'' . The volume of parallelepiped T is 90 percent of the volume of parallelepiped S. Prove that the locus of A is a plane.
View Solution