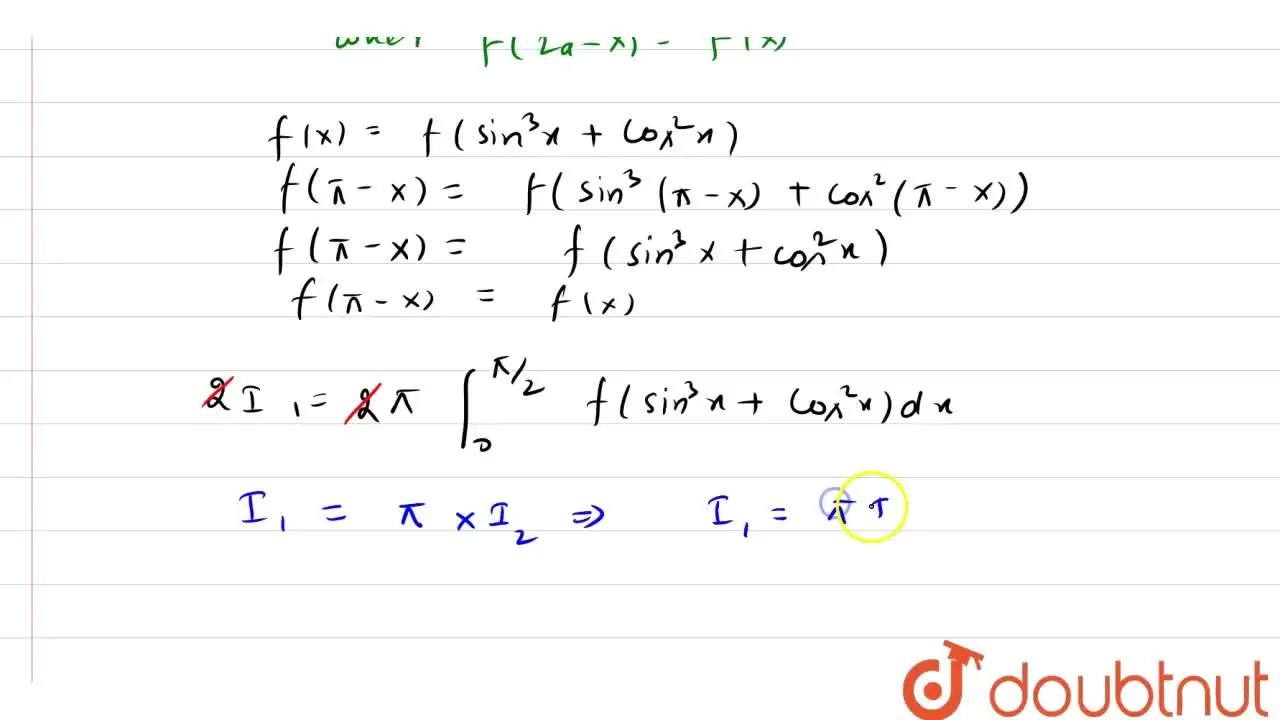
If I1=∫π0xf(sin3x+cos2x)dxand I2=∫π20f(sin3x+cos2x)dx,thenrelateI1andI2
Step by step video & image solution for If I_1=int_0^pixf(sin^3x+cos^2x)dxa n d I_2=int_0^(pi/2)f(sin^3x+cos^2x)dx ,t h e nr e l a t eI_1a n dI_2 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
If I1=∫π0xf(sin3x+cos2x)dxand I2=∫π20f(sin3x+cos2x)dx,thenrelateI1andI2
View Solution∫π20sin3xsin3x+cos3xdx
View SolutionI=∫2π0esin2x+sinx+1dx then
View SolutionEvaluate: ∫π/2014sin2x+5cos2xdx
View SolutionIf In = ∫π20sin2nxsin2xdx, then
View SolutionEvaluate: ∫π/20sinx1+cos2xdx
View SolutionIfI1=∫π20cos2x1+cos2xdx,I2=∫π20sin2x1+sin2xdx I3=∫π201+2cos2xsin2x4+2cos2xsin2xdx,then I1=I2>I3 (b) I3>I1=I2 I1=I2=I3 (d) none of these
View SolutionIf I1=∫03πf(cos2x)dx and I2=∫0πf(cos2x) then
View SolutionIf I1=∫π/20cos2x1+cos2xdx , I2=∫π/20sin2x1+sin2xdx and I3=∫π/201+2cos2xsin2x4+2cos2xsin2xdx, then (a) I1=I2>I3 (b) I3>I1=I2 (c) I1=I2=I3 (d) none of these
View SolutionIfII=∫π/20cos(sinx)dx,I2=∫π20sin(cosx)d,andI3=∫π20cosxdx, then find the order in which the values I1,I2,I3, exist.
View Solution