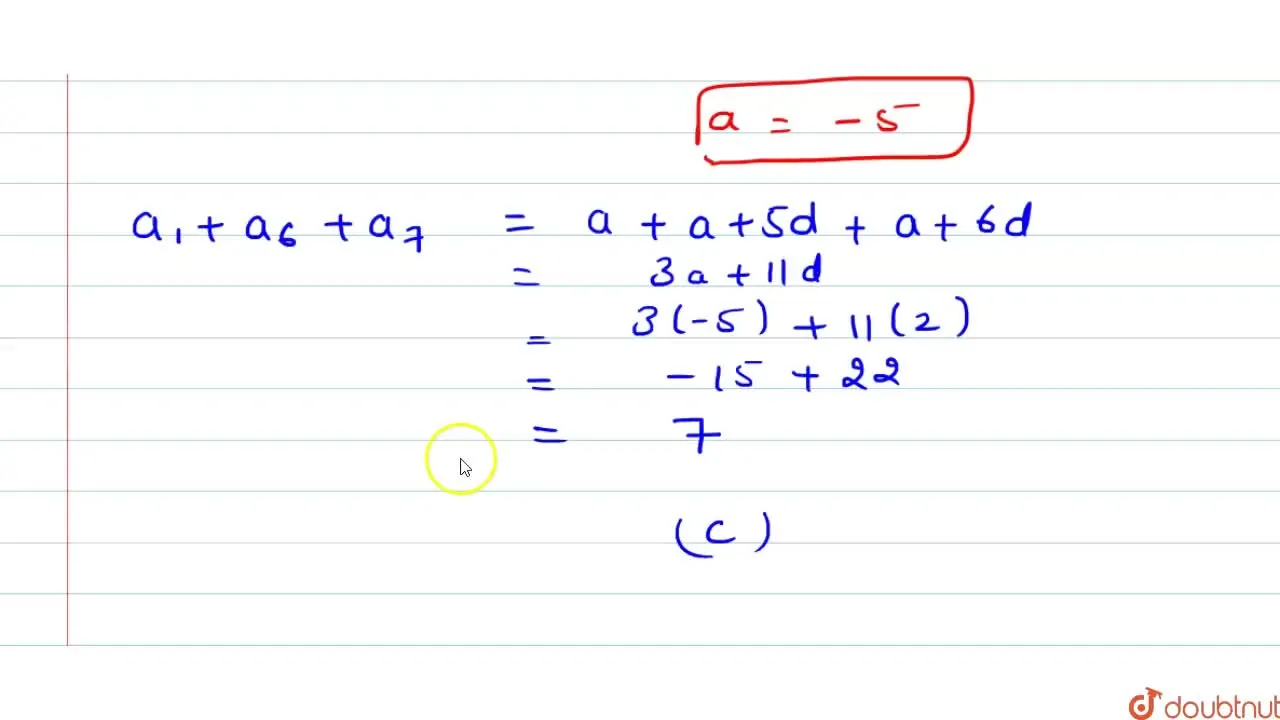
Consider an A.P. a1,a2,a3,......... such that a3+a5+a8=11anda4+a2=−2, then the value of a1+a6+a7 is (a). -8 (b). 5 (c). 7 (d). 9
Step by step video & image solution for Consider an A.P. a_1, a_2, a_3,......... such that a_3+a_5+a_8=11 and a_4+a_2=-2, then the value of a_1+a_6+a_7 is (a). -8 (b). 5 (c). 7 (d). 9 by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
Consider an A.P.a1,a2,a3,..... such that a3+a5+a8=11anda4+a2=−2 then the value of a1+a6+a7 is.....
View SolutionIf for an A.P.a1,a2,a3,........,an,........a1+a3+a5=−12anda1a2a3=8, then the value of a2+a4+a6 equals
View SolutionLet {an} bc a G.P. such that a4a6=14 and a2+a5=216. Then a1=
View SolutionLet a1,a2,a3,....an,.........be in A.P. If a3+a7+a11+a15=72, then the sum of itsfirst 17 terms is equal to :
View SolutionLet a1,a2,a3……,an be in G.P such that 3a1+7a2+3a3−4a5=0 Then common ratio of G.P can be
View SolutionIf a1+a2+a3+......+an=1∀ai>0,i=1,2,3,......,n, then find the maximum value of a1a2a3a4a5......an.
View SolutionThree distinct numbers a1,a2,a3 are in increasing G.P. a21+a22+a23=364and a1+a2+a3=26 then the value of a10 if an is the nth term of the given G.P. is:
A. 2.39
B. 39
C. 2.310
D. 312View SolutionLet a1,a2,a3.......... be in A.P.andh1,h2,h3.....,in H.P. If a1=2=h1,anda30=25=h30 then a7h24+a14+a17=
View SolutionFor an increasing A.P. a1,a2,anifa1=a2+a3+a5=−12 and a1a3a5=80, then which of the following is/are true? a.a1=−10 b. a2=−1 c. a3=−4 d. a5=+2
View SolutionFor an increasing A.P. a1,a2,anifa1=a2+a3+a5=−12 and a1a3a5=80, then which of the following is/are true? a1=−10 b. a2=−1 c. a3=−4 d. a5=+2
View Solution