Solve sin−1x+sin−12x=π3.
Video Solution
Text Solution
sin−1x+sin−1(2x)=π3
⇒sin−1(2x)=π3−sin−1x
⇒sin−1(2x)=sin−1(√32)−sin−1x
⇒sin−1(2x)=sin−1[√32√1−x2−x√1−34]
⇒sin−1(2x)=sin−1[√32√1−x2−x2)]
⇒2x=√32√1−x2−x2
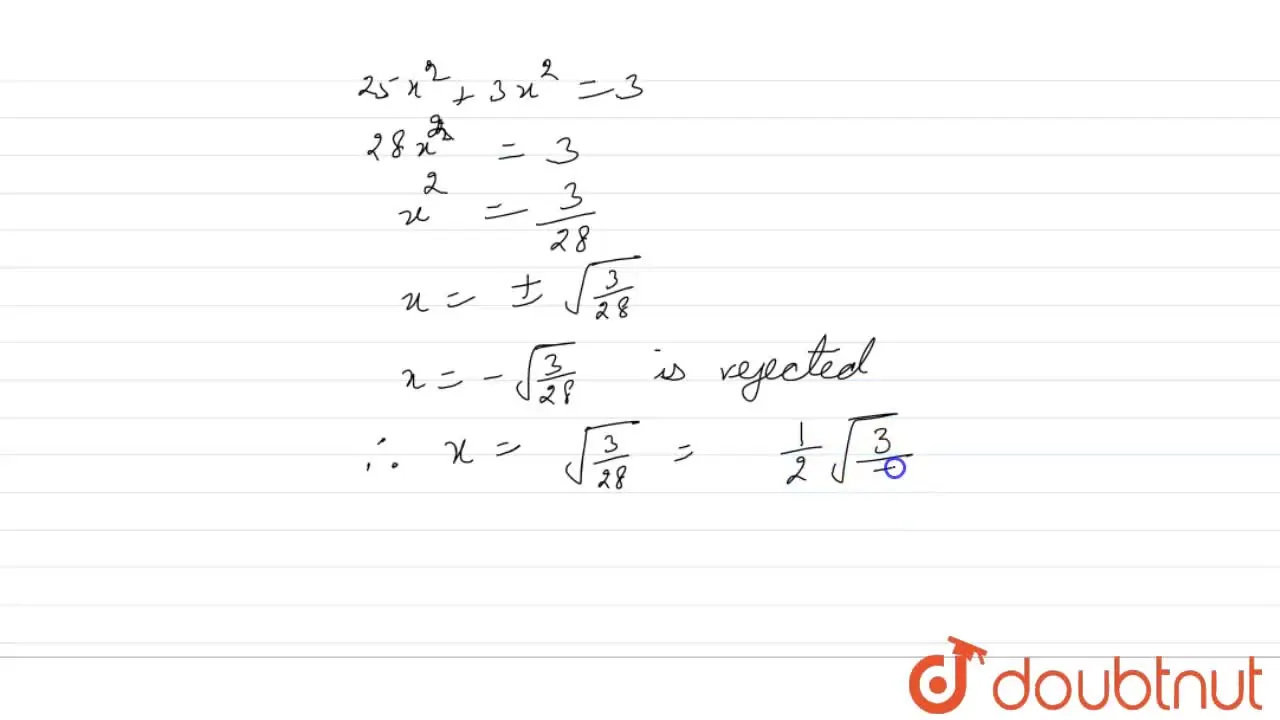
⇒5x2=√32√1−x2
Squaring both sides,
25x24=34−34x2
⇒284x2=34
⇒x=±√328
As, x=−√328 will make the equation negative, so we will reject it.
∴x=√328=12√37.
|
Updated on:21/07/2023
Similar Questions
Solve sin−1(1−x)−2sin−1x=π2
View Solutionsolve:sin−1x+sin−12x=π3
View Solutionsolve:sin−1x+sin−12x=π3
View SolutionSolve sin−1(1−x)−2sin−1x=π2
View SolutionIf sin−1(x)+sin−1(2x)=π3 then x=
View SolutionSolve 2sin−1x+sin−1(1−x)=π2
View SolutionSolve : sin−1(x)+sin−1(2x)=sin−1(√32).
View SolutionThe solution of sin−1x−sin−12x=±π3 is
View SolutionSolve : sin(sin−1x)=12
View SolutionSolve : 4sin−1x=π−cos−1x
View Solution