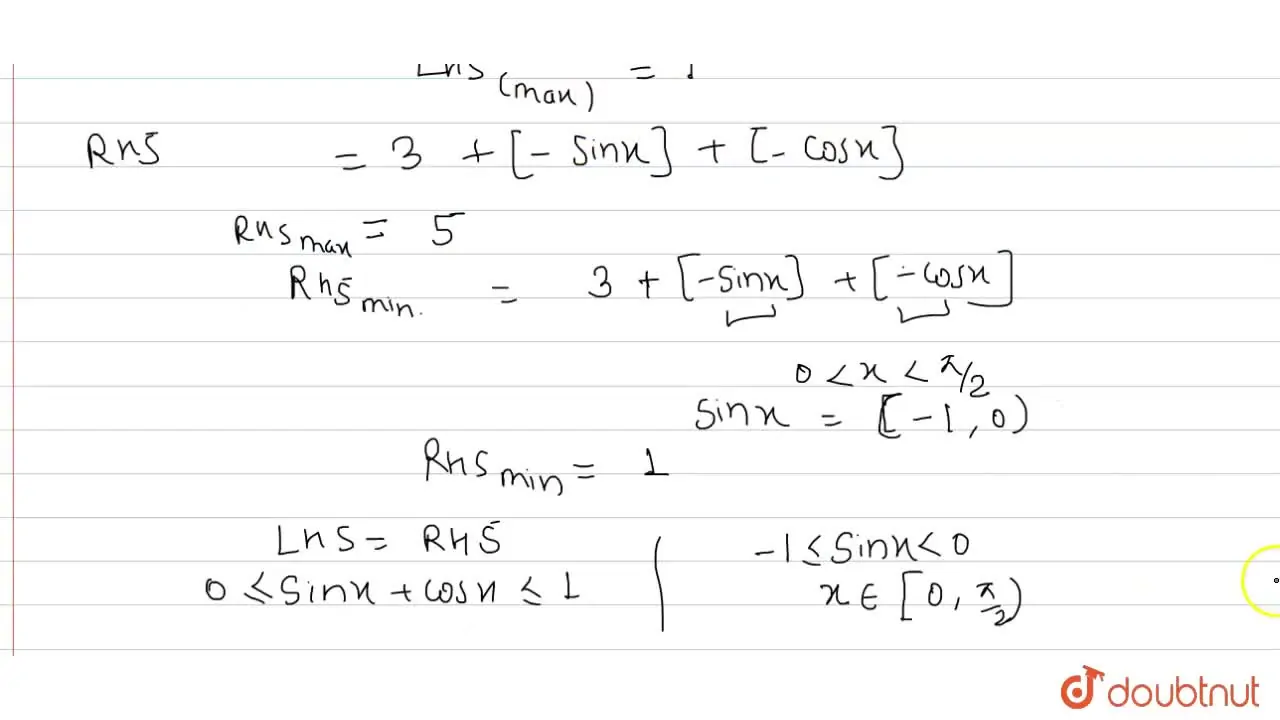The number of solutions of [sinx+cosx]=3+[−sinx]+[−cosx](where[] denotes the greatest integer function), x∈[0,2π] , is 0 (b) 4 (c) infinite (d) 1
Step by step video & image solution for The number of solutions of [sinx+cosx]=3+[-sinx]+[-cosx](w h e r e[] denotes the greatest integer function), x in [0,2pi] , is 0 (b) 4 (c) infinite (d) 1 by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
Find the number of solution of [cosx]+∣sinx=1∈π≤x≤3π where [] denotes the greatest integer function.
View SolutionThe number of points where f(x)=[sinx+cosx] (where [.] denotes the greatest integer function) x∈(0,2π) is not continuous is
View SolutionFind the number of solution of [cosx]+|sinx|=1,x∈π≤x≤3π (where [ ] denotes the greatest integer function).
View SolutionNumber of solutions of the equation cos[x]=e2x−1,x∈[0,2π], where[.] denotes the greatest integer function is
View SolutionThe range of the function f(x)=[sinx+cosx] (where [x] denotes the greatest integer function) is f(x)∈ :
View SolutionEvaluate: ∫2π0[sinx]dx,where[.] denotes the greatest integer function.
View SolutionEvaluate:- ∫π0[cotx]dx,where[.] denotes the greatest integer function.
View SolutionEvaluate: ∫5−5x2[x+12]dx(where[.] denotes the greatest integer function).
View SolutionThe number of roots of x2−2=[sinx],where[.] stands for the greatest integer function is 0 (b) 1 (c) 2 (d) 3.
View SolutionThe number of roots of x2−2=[sinx],where[.] stands for the greatest integer function is 0 (b) 1 (c) 2 (d) 3.
View Solution