If sin2θ=x2+y2+12x , then x must be −3 (b) −2 (c) 1 (d) none of these
sin2θ=x2+y2+12x
x2+y2+12x=<1
x2+y2+1≤2x
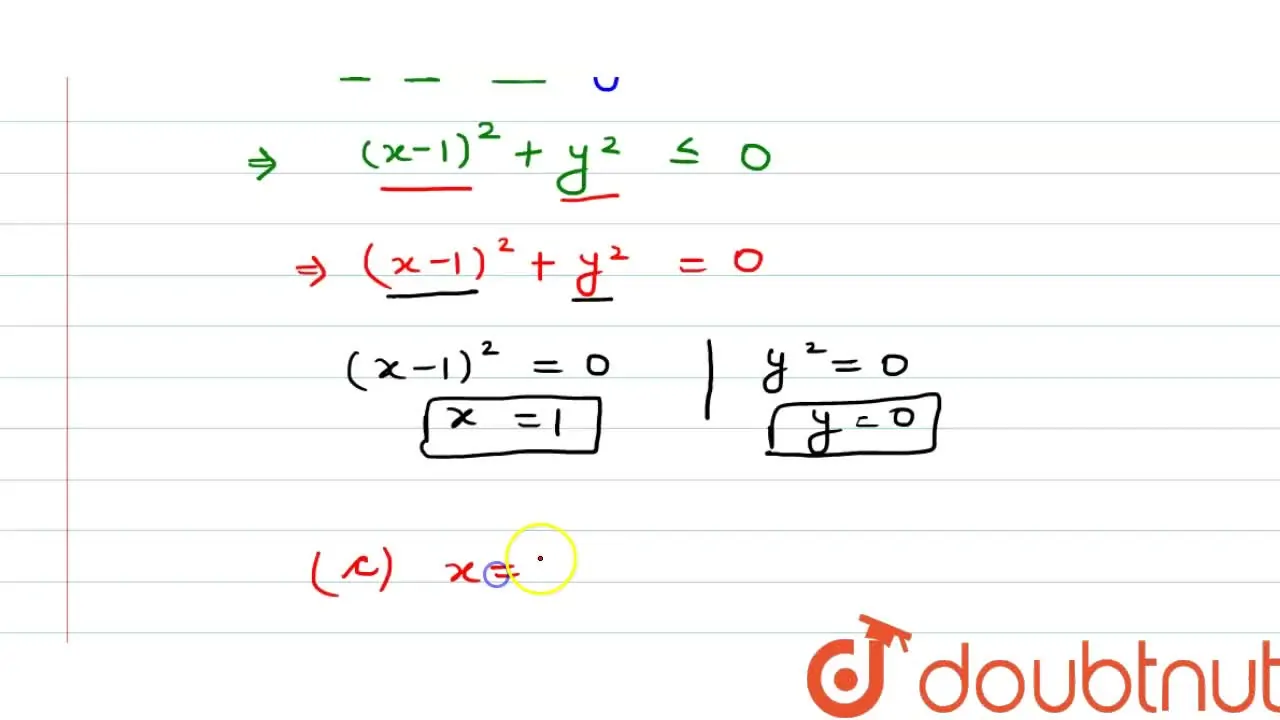
x2+y2−2x+1≤0
(x−1)2+y2≤0
x−1=0,y=0
x=1,y=0.
Related Playlists
Similar Questions
If sin2θ=x2+y2+12x. Find the value of x and y.
View SolutionIf sin−1x+sin−1y=π2,then1+x4+y4x2−x2y2+y2 is equal to 1 (b) 2 (c) 12 (d) none of these
View SolutionIf sin−1x+sin−1y=π2,then1+x4+y4x2−x2y2+y2 is equal to 1 (b) 2 (c) 12 (d) none of these
View SolutionIf x2+y2=t−1tandx4+y4=t2+1t2, then x3ydydx=
(a) 0 (b) 1(c) −1 (d) none of theseView SolutionIf y=(sin−1x)2, then (1−x2)y2 is equal to xy1+2 (b) xy1−2 (c) xy1+2 (d) none of these
View SolutionIf sin−1x−cos−1x=π6 , then x= (a)12 (b) √32 (c) −12 (d) none of these
View SolutionIf xcosθ=ycos(θ−2π3)=zcos(θ+2π3) then x+y+z is (a)1 (b) 0 (c) −1 (d) none of these
View SolutionLet f(x)=(x−a)2+(x−b)2+(x−c)2. Then, f(x) has a minimum at x= a+b+c3 (b) 12 (c) 18 (d) none of these
View SolutionIf f′(x)=√2x2−1andy=f(x2),thendydxatx=1 is (a)2 (b) 1 (c) −2 (d) none of these
View SolutionIf sinx+siny=√3(cosy−cosx), then sin3x+sin3y=___________ (a) 2sin3x (b) 0 (c) 1 (d) none of these
View Solution