Define an equipotential surface. Draw equipotential surfaces :
(i) in the case of single point charge and
(ii) in a constant electric field in Z-direction.
Why the equpotential surfaces about a single charge are not equidistant ?
(iii) Can electric field exist tangential to an equipotential surface ? Given reason.
Video Solution
Text Solution
(i) 
(ii) A surface where potential at every point is same called equipotential surface.
Because electric field goes on decreasing as we move away from charge. So, potential also decreases.
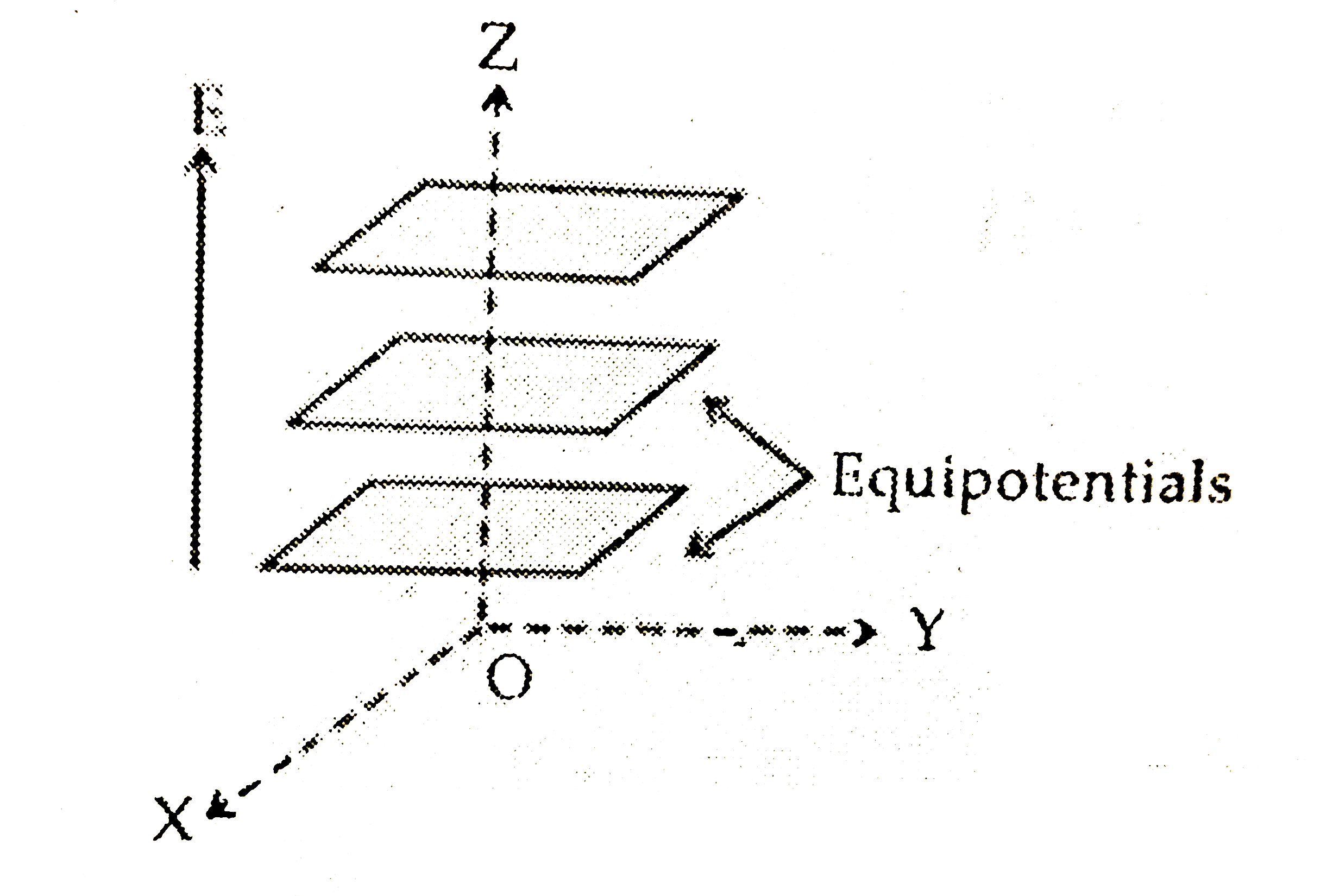
(iii) Let , electric field is tangent to equipotential surface, then work has to be done in moving a charge over surface but work done in moving a charge over an equipotential surface is zero so it contradicts our assumption.
|
Similar Questions
Knowledge Check
The angle between the equipotential surface and the electric field (or line of force) at any point on the equipotential surface is
A90∘ alwaysB0∘ alwaysC0∘ to 90∘D0∘ to 180∘
What is the geometrical shape of equipotential surfaces due to a single isolated charge ?
View SolutionWhat is the geometrical shape of equipotential surface due to a single isolated charge ?
View SolutionWhy are electric field lines perpendicular at a point on an equipotential surface of a conductor ?
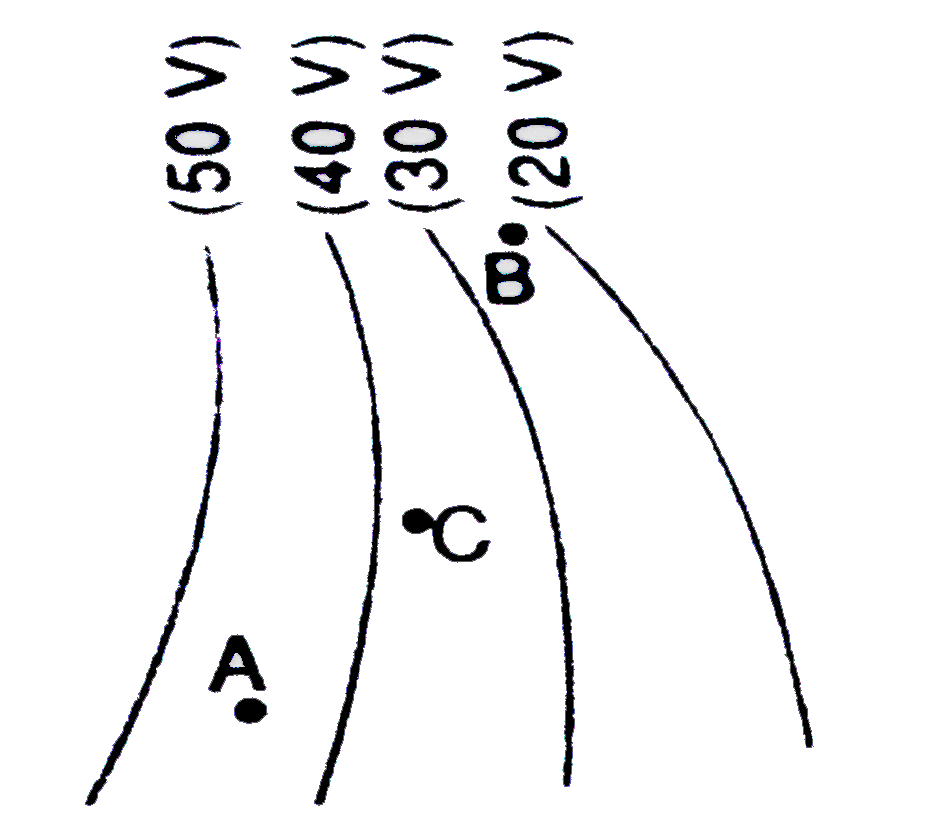
View SolutionFigure shows sone equipotential surfaces produce by some charges. At which point, the value of electric field is greatest ?
View SolutionDescribe schematically the equipotential surfaces corresponding to
A single positive charge at the origin.
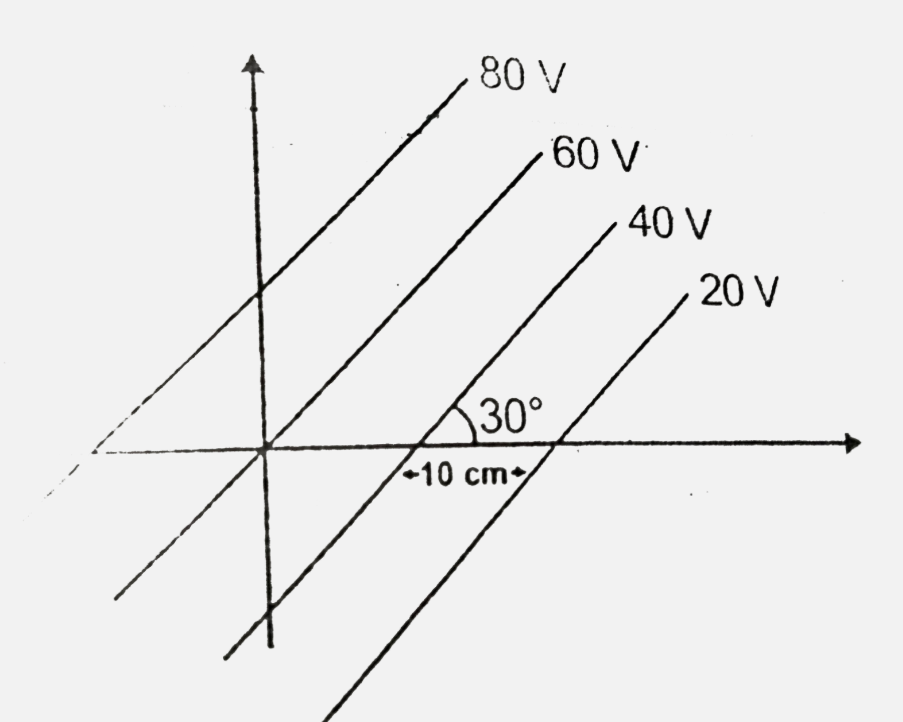
View SolutionIf the given figure shows equipotential surfaces, then the magnitude of electric field is
View SolutionSome equipotential surfaces are shown in figure(29.E3) What can you say about the magnitude and the direction of the electric field?

View Solution
