Find the area if the region bounded by y=√x and y=x.
Video Solution
Text Solution
The correct Answer is:N/a
Given equation of curves are y=√x and y=x.
⇒x=√x⇒x2=x
⇒x2−x=0⇒x(x−1)=0
⇒x=0.1
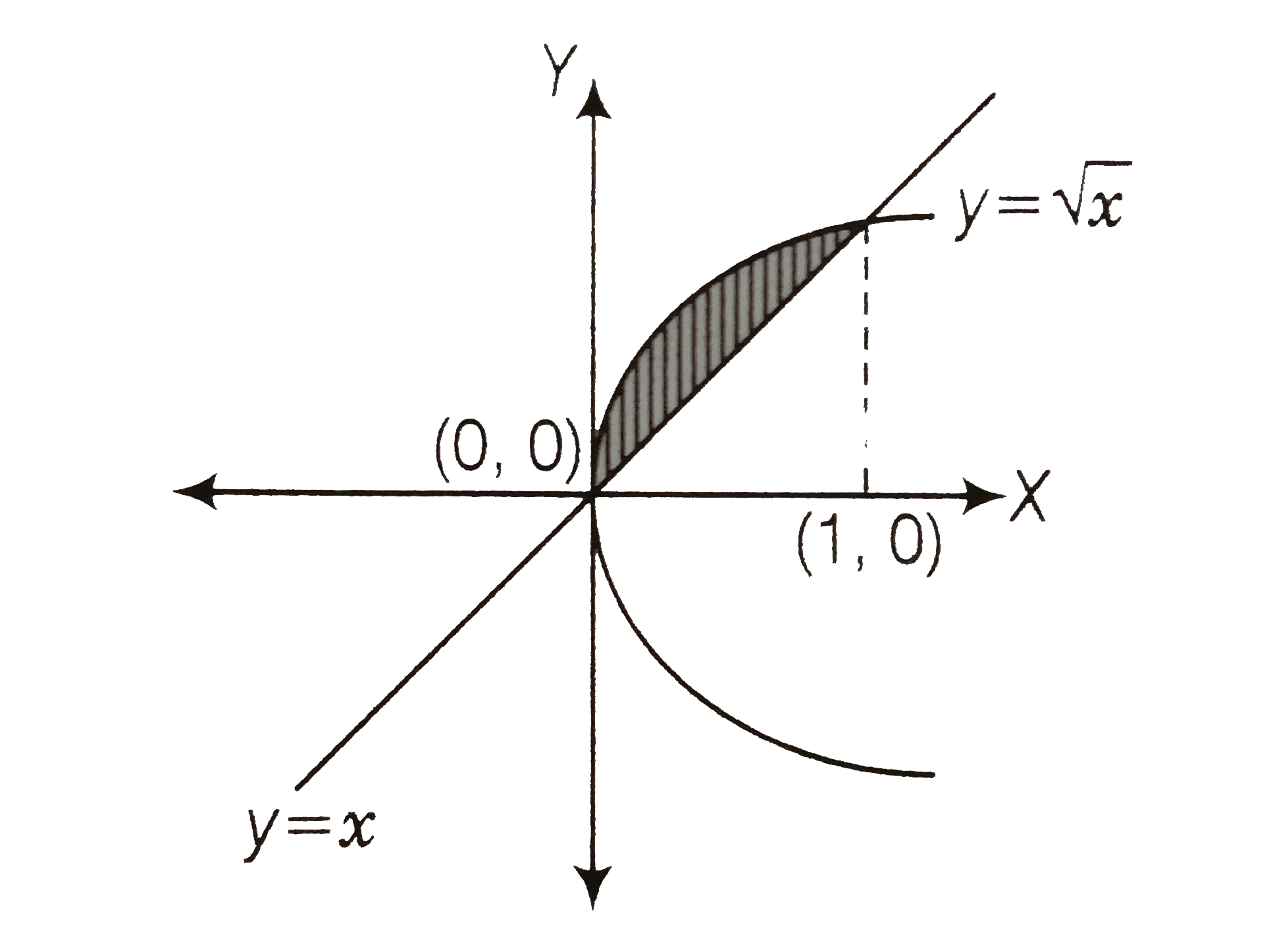
∴ Required area of shaded region, A=∫10(√x)dx−∫10xd
=[2.x3/23]10−[x22]10
=23.1−12=23−12=16 sq units
|
Topper's Solved these Questions
- APPLICATION OF INTEGRALSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Long Answer Type Questions
16 Videos
- APPLICATION OF INTEGRALSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Objective Type Questions
22 Videos
- APPLICATION OF DERIVATIVESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Fillers
5 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - True/False
10 Videos
Similar Questions
Find the area of the region bounded by y=√xandy=x.
View SolutionFind the area of the region bounded by
y=ex and y = x between x = 0 and x = 1.
View SolutionFind the area of the region bounded by
y=cosx and the x axis in the interval [0,2π]
View SolutionFind the area of the region bounded by y=√x, x=2y+3 in the first quadrant and x-axis.
View SolutionUsing integration, find the area of the region bounded by the curves y=x and y=x3.
View SolutionFind the area of the region bounded by y=3x and the lines y = 3 and x = 0.
View SolutionFind the area of the region bounded by 4y=∣∣4−x2∣∣ and y=7−|x|
View SolutionFind the area of the region bounded by y=−1,y=2,x=y3andx=0.
View SolutionThe area of the region bounded by y=|x−1|andy=3−|x|, is
View SolutionThe area of the region bounded by y=|x|andy=1−|x| is
View Solution
