Question

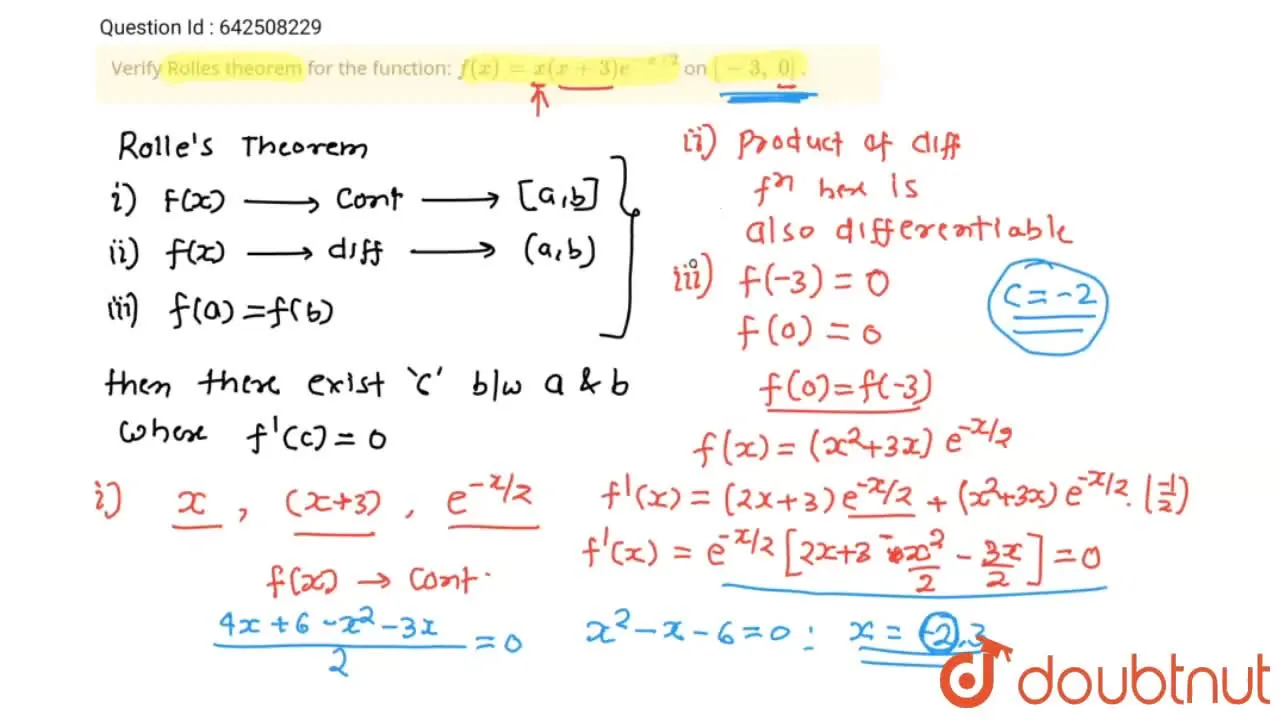
Verify Rolles theorem
for the function: $f\left(x\right)=x(x+3)e^{-x/2}$
on $[-3,0]$
.

Answer

N/a

Explanation

To verify Rolle's Theorem for the function $$f\left(x\right)=x(x+3)e^{-\frac{x}{2}}$$ on the interval $$(-3,0)$$, we will follow the steps outlined in the theorem.

Step 1: Check Continuity on the Closed Interval \((-3, 0)\)

Explanation:
Rolle's Theorem states that if \( f(x) \) is continuous on the closed interval \((a, b)\), then we can proceed with the theorem.

The function \( f(x) = x(x+3)e^{-\frac{x}{2}} \) is a product of three functions:
1. \( x \) (a polynomial, hence continuous everywhere),
2. \( x + 3 \) (also a polynomial, hence continuous everywhere),
3. \( e^{-\frac{x}{2}} \) (an exponential function, hence continuous everywhere).

Since all these functions are continuous, their product \( f(x) \) is also continuous on \((-3, 0)\).

Step 2: Check Differentiability on the Open Interval \((-3, 0)\)

Explanation:
Next, we need to check if \( f(x) \) is differentiable on the open interval \((-3, 0)\).

Since \( f(x) \) is composed of polynomials and an exponential function, which are both differentiable everywhere, \( f(x) \) is differentiable on \((-3, 0)\).

Step 3: Check if \( f(a) = f(b) \)

Explanation:
We need to check if \( f(-3) = f(0) \).

Calculating \( f(-3) \):
\( f(-3) = (-3)(-3 + 3)e^{-\frac{-3}{2}} = (-3)(0)e^{\frac{3}{2}} = 0 \)

Calculating \( f(0) \):
\( f(0) = (0)(0 + 3)e^{-\frac{0}{2}} = (0)(3)e^{0} = 0 \)

Since \( f(-3) = 0 \) and \( f(0) = 0 \), we have \( f(-3) = f(0) \).

Step 4: Find \( c \) such that \( f'(c) = 0 \)

Explanation:
Now we need to find the derivative \( f'(x) \) and solve for \( c \) in the interval \((-3, 0)\) such that \( f'(c) = 0 \).

Using the product rule:
Let \( u = x(x+3) \) and \( v = e^{-\frac{x}{2}} \).

Then,
\( f'(x) = u'v + uv' \)
Calculating \( u' \):
\( u = x^2 + 3x \implies u' = 2x + 3 \)
Calculating \( v' \):
\( v = e^{-\frac{x}{2}} \implies v' = e^{-\frac{x}{2}} \cdot \left(-\frac{1}{2}\right) \)

Now substituting back:
\( f'(x) = (2x + 3)e^{-\frac{x}{2}} + (x^2 + 3x)\left(-\frac{1}{2}e^{-\frac{x}{2}}\right) \)
Factoring out \( e^{-\frac{x}{2}} \):
\( f'(x) = e^{-\frac{x}{2}} \left( (2x + 3) - \frac{1}{2}(x^2 + 3x) \right) \)
Simplifying:
\( f'(x) = e^{-\frac{x}{2}} \left( 2x + 3 - \frac{x^2}{2} - \frac{3x}{2} \right) \)
\( = e^{-\frac{x}{2}} \left( -\frac{x^2}{2} + \frac{x}{2} + 3 \right) \)
Setting \( f'(x) = 0 \):
\( -\frac{x^2}{2} + \frac{x}{2} + 3 = 0 \)
Multiplying through by -2:
\( x^2 - x - 6 = 0 \)
Factoring:
\( (x - 3)(x + 2) = 0 \)
Thus, \( x = 3 \) or \( x = -2 \).

Since we are looking for \( c \) in the interval \((-3, 0)\), we take \( c = -2 \).

Conclusion

Since all conditions of Rolle's Theorem are satisfied, we conclude that there exists a \( c \) in the interval \((-3, 0)\) such that \( f'(c) = 0 \). In this case, \( c = -2 \).