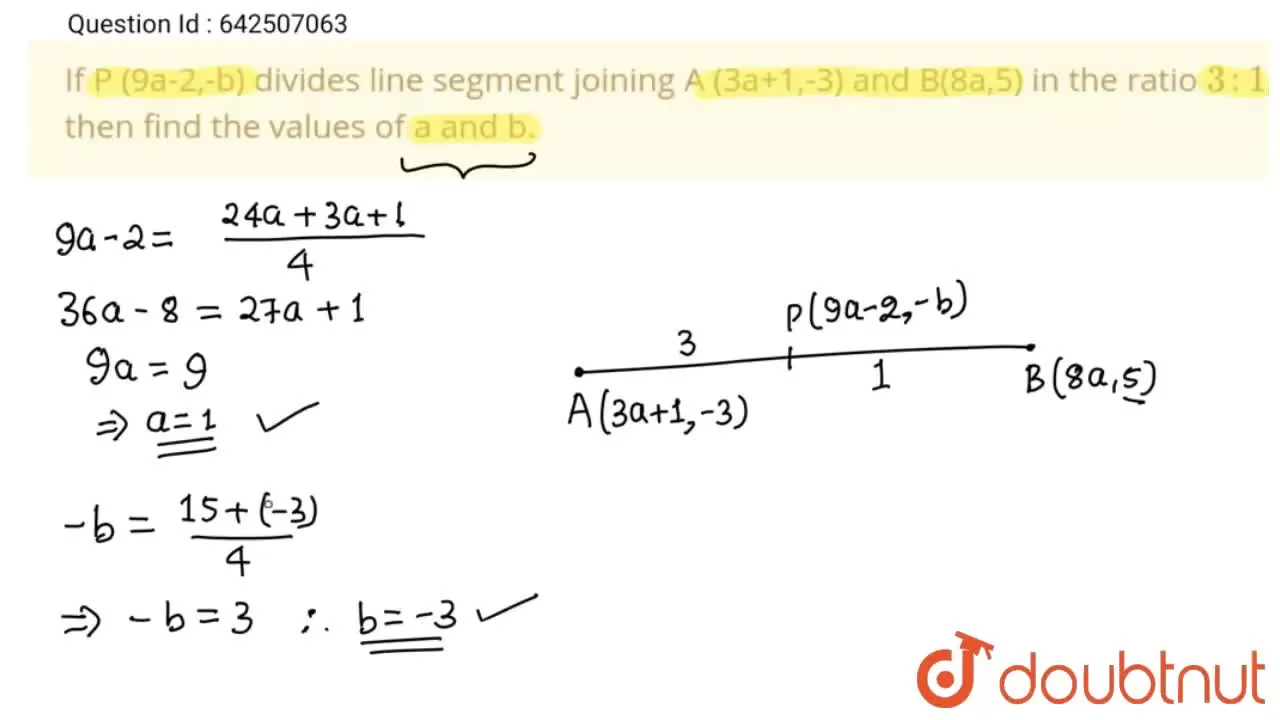
If P (9a-2,-b) divides line segment joining A (3a+1,-3) and B(8a,5) in the ratio 3:1, then find the values of a and b.
Let P(9a-2,-b) divides AB internally in the ration 3:1.
By section formula ,
9a−2=3(8a)+1(3a+1)3+1
[∵ internal section formula , the coordinates of point P divides the line seggment joining the Point (x2,y1)and(x2,y2) in the ratio m1:m2 internally is (m2x1+m1x2m1+m2,m2y1+m1y2m1+m2)]
and −b=3(5)+1(−3)3+1
⇒9a−2=24a+3a+14
and −b=15−34
⇒9a−2=27a+14
and −b=124
⇒36a−27a−8−1=0
⇒9a−9=0
∴a=1 Hence , the required values of a and b are 1 and -3.
Related Playlists
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 7.4 Long Answer Type Questions6videos
COORDINATE GEOMETRY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 7.2 Very Short Answer Type Questions12videos
COORDINATE GEOMETRY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Exercise 10.4 Long Answer type Questions7videos
CONSTRUCTIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONSEXERCISE - LONG ANSWER TYPES QUESTIONS18videos
INTRODUCTION TO TRIGoNOMETRY AND ITS APPLICATIONS
Similar Questions
If P(9a−2,−b) divides the line segment joining the points A(31+1,−3)andB(81,5) in the ratio 3:1: Find the values of a and b.
View SolutionIf the point P (−1,2) divides the line segment joining A (2,5) and B in the ratio 3:4 , find the co-ordinate of B .
View SolutionIf point C(−4,1) divides the line segment joining the point A(2,−2) and B in the ratio 3:5, then the coordinates of B are
View SolutionIf the point P(-1,2) divides the join of points A(2, 5) and B(a, b) in the ratio 3 : 4, find the value of a×b−a.
View SolutionFind the point which divides the line segment joining
(-1,2), (4,-5) in the ratio 3 : 2View SolutionIn what ratio does the point P (a, 2) divide the line segment joining the points A(5,-3) and B(-9, 4) ? Also, find the value of 'a'.
View SolutionIf the point C(−1,2) divides internally the line segment joining A(2,5) and B in ratio 3:4, find the coordinates of B.
View SolutionIn what ratio does the point M(p−1) divide the line segment joining the points A(1,−3)andB(6,2) ? Hence, find the value of p.
View SolutionThe midpoint of the line segment joining A(29,4) and B(-2,3b) is C(2a+1,1).Find the values of a and b.
View SolutionThe mid-point of the line segment joining (4a, 23) and (-4, 3b) is (2, -2a). Find the values of a and b
View Solution