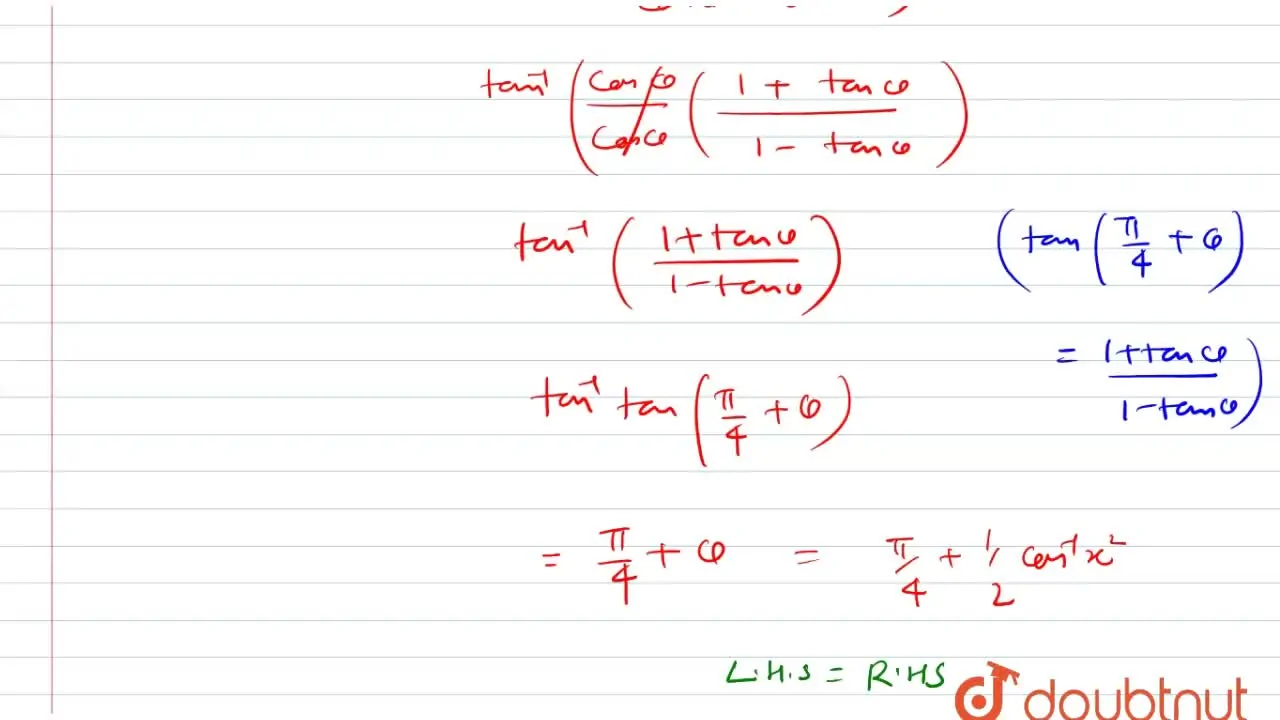
Prove that tan−1[√1+x2+√1−x2√1+x2−√1−x2]=π4+12cos−1x2

Video Solution
Doubtnut Promotions Banner
Text Solution
Generated By DoubtnutGPT

The correct Answer is:N/a

To prove that

tan−1(√1+x2+√1−x2√1+x2−√1−x2)=π4+12cos−1(x2)

we will follow these steps:

Step 1: Substitute x2=cos(2θ)

Let x2=cos(2θ). Then, we can express √1+x2 and √1−x2 in terms of θ:

√1+x2=√1+cos(2θ)=√2cos2(θ)=√2cos(θ)

√1−x2=√1−cos(2θ)=√2sin2(θ)=√2sin(θ)

Step 2: Substitute these into the original expression

Now substitute these values into the left-hand side:

tan−1(√2cos(θ)+√2sin(θ)√2cos(θ)−√2sin(θ))

This simplifies to:

tan−1(cos(θ)+sin(θ)cos(θ)−sin(θ))

Step 3: Use the tangent addition formula

Using the tangent addition formula, we know that:

tan(π4+θ)=1+tan(θ)1−tan(θ)

In our case, we can express tan(θ)=sin(θ)cos(θ). Therefore,

tan(π4+θ)=1+sin(θ)cos(θ)1−sin(θ)cos(θ)=cos(θ)+sin(θ)cos(θ)−sin(θ)

Thus,

tan−1(cos(θ)+sin(θ)cos(θ)−sin(θ))=π4+θ

Step 4: Substitute back for θ

Since θ=12cos−1(x2), we can substitute back:

tan−1(cos(θ)+sin(θ)cos(θ)−sin(θ))=π4+12cos−1(x2)

Conclusion

Thus, we have shown that:

tan−1(√1+x2+√1−x2√1+x2−√1−x2)=π4+12cos−1(x2)

Hence, the proof is complete.

---

Updated on:7/8/2024
|