If the eccentricity of an ellipse is 58 and the distance between its foci is 10, then find the latusrectum of the ellipse.
Given that, eccentricity=58i.e.,e=58
Let equation of the ellipse be x2a2+y2b2=1 ltvbrgt since the foci of the ellipse is )±ae,o).
∴ Distance between foci =√(ae+ae)2
⇒2√a2e2=10 [∵ distance between the foci=10]
⇒√a2e2=5
⇒a2e2=25
⇒a2=25×6425
∴a=8
We know that,
⇒b2=a2(1−e2)
⇒b2=64(1−2564)
⇒b2=64(64−2564)
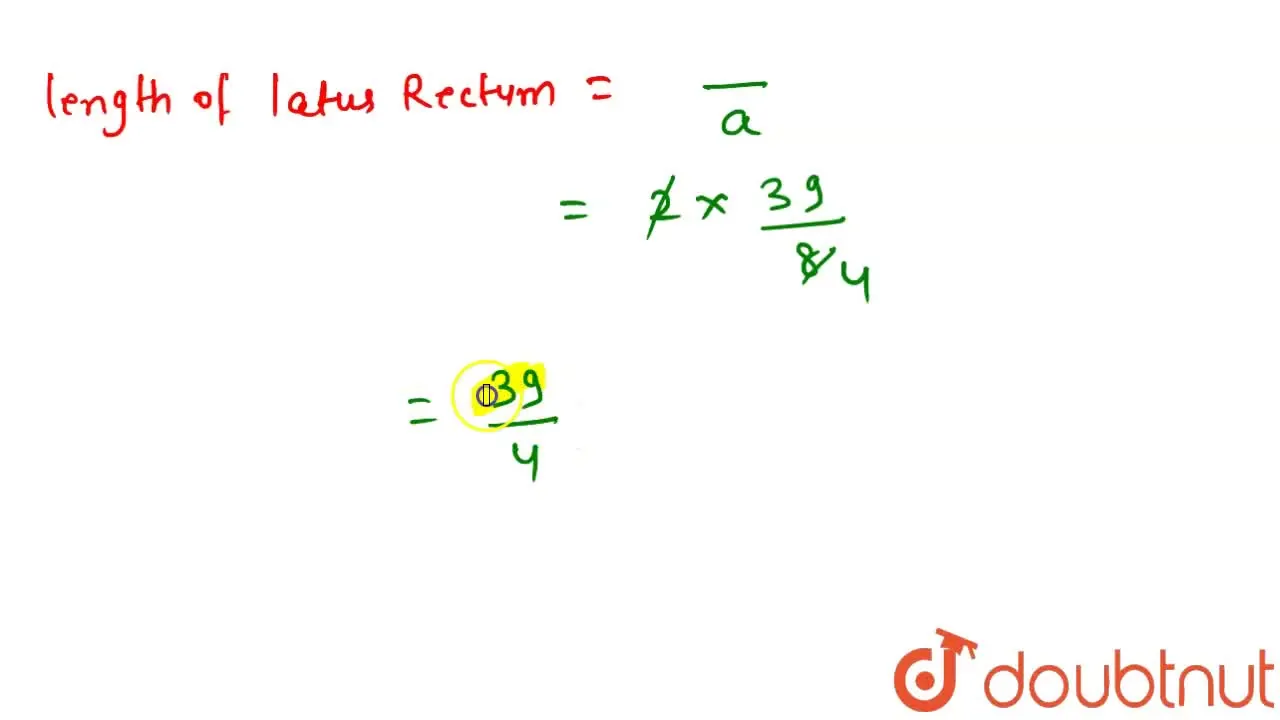
b2=39
∴ Length of latusrectum of ellipse=2b2a=2(398)=394
Related Playlists
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - Long answer10videos
CONIC SECTIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - True/False8videos
CONIC SECTIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - OBJECTIVE TYPE QUESTIONS16videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO THREE DIMENSIONAL GEOMETRYEXERCISE - Fillers16videos
INTRODUCTION TO THREE DIMENSIONAL GEOMETRY
Similar Questions
If the eccentricity of and ellipse is 58 and the distance between its foci is 10, then length of its latus rectum is
View SolutionIf the eccentricity of an ellipse is 49 and the distance between its foci is 8 units, then find the length of the latus rectum of the ellipse.
View SolutionThe eccentricity of the ellipse is 25 and the distance between the foci is 10. Find the length of the latus rectum of the ellipse.
View SolutionIf the length of the eccentricity of an ellipse is 38 and the distance between the foci is 6 units, then the length of the latus rectum of the ellipse is
View SolutionThe eccentricity of the ellipse, if the distance between the foci is equal to the lenght of the latus rectum, is
View SolutionThe eccentricity of an ellipse is 12 and the distance between its foci is 4 units. If the major and minor axes of the ellipse are respectively along the xandy axes, find the equation of the ellipse
View SolutionIf the focal distance of one ed of minor axis of an ellipse is k and distance betwnn foci is 2h then find the equation of the ellipse.
View SolutionThe focal distance of an end of the minor axis of the ellipse is k and the distance between the foci is 2h. Find the lengths of the semi-axes.
View SolutionThe eccentricity of the ellipse if the distance between the foci is equal to the length of the latus rectum is a.√5−12 b. √5+12 c. √5−14 d. none of these
View SolutionIf the eccentricity of an ellipse is 1√2, then its latusrectum is equal to its
View Solution