How many committee of five person with a chairperson can be selected from 12 persons ?
960
3960
2960
1960
The correct Answer is:B
To solve the problem of how many committees of five persons with a chairperson can be selected from 12 persons, we can follow these steps:
1. Select the Chairperson:
We need to select 1 chairperson from the 12 persons. The number of ways to choose 1 chairperson from 12 persons is given by the combination formula (nr), where n is the total number of persons and r is the number of persons to choose.
Ways to choose chairperson=(121)=12
2. Select the Other Members:
After selecting the chairperson, we need to select 4 more members from the remaining 11 persons (since one person has already been chosen as the chairperson). The number of ways to choose 4 members from 11 persons is:
Ways to choose 4 members=(114)
3. Calculate (114):
Using the combination formula (nr)=n!r!(n−r)!:
(114)=11!4!(11−4)!=11!4!⋅7!
This simplifies to:
=11×10×9×84×3×2×1
Calculating this step-by-step:
- The numerator: 11×10=110
- 110×9=990
- 990×8=7920
- The denominator: 4×3=12
- 12×2=24
- 24×1=24
Now divide the numerator by the denominator:
792024=330
4. Total Committees:
Now, we multiply the number of ways to choose the chairperson by the number of ways to choose the other members:
Total ways=Ways to choose chairperson×Ways to choose 4 members=12×330=3960
Final Answer:
The total number of committees of five persons with a chairperson that can be selected from 12 persons is 3960.
---
We need to select 1 chairperson from the 12 persons. The number of ways to choose 1 chairperson from 12 persons is given by the combination formula (nr), where n is the total number of persons and r is the number of persons to choose.
After selecting the chairperson, we need to select 4 more members from the remaining 11 persons (since one person has already been chosen as the chairperson). The number of ways to choose 4 members from 11 persons is:
Using the combination formula (nr)=n!r!(n−r)!:
- 110×9=990
- 990×8=7920
- 12×2=24
- 24×1=24
Now, we multiply the number of ways to choose the chairperson by the number of ways to choose the other members:
The total number of committees of five persons with a chairperson that can be selected from 12 persons is 3960.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Long Answer Type Questions6videos
PERMUTATIONS AND COMBINATIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONSEXERCISE - Objective Type Questions15videos
PERMUTATIONS AND COMBINATIONS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MATHEMATICAL REASONING EXERCISE - OBJECTIVE TYPE QUESTIONS21videos
MATHEMATICAL REASONING
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL INDUCTION EXERCISE - OBJECTIVE TYPE QUESTIONS5videos
PRINCIPLE OF MATHEMATICAL INDUCTION
Similar Questions
Knowledge Check
- Question 1 - Select One
In how many ways can 2 doors be selected from 3 doors ?
A1B3C6D9 - Question 2 - Select One
How many 3 - person committees can be selected from a fraternity with 25 members?
A15625B13800C2300D75 - Question 3 - Select One
In how many ways a committee consisting of 3 men and 2 women can be chosen from 7 men and 5 women?
A(a) 45B(b) 350C(c) 4200D(d) 230
In how many ways a committee of 5 member can be selected from 6 men and 5 women, consisting of 3 men 2 women?
View SolutionA committee of three persons is to be randomly selected from a group of three men and two women and the chair person will be randomly selected from 2 woman and 1 men for the committee. The probability that the committee will have exactly two woman and one man and that the chair person will be a woman, is
View SolutionA committee of 5 persons is to be randomly selected from a group of 5 men and 4 women and a chairperson will be randomly selected from the committee will have exactly 2 women and 3 men and the chairperson will be a man is p, then 1p is equal to
View SolutionFrom the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
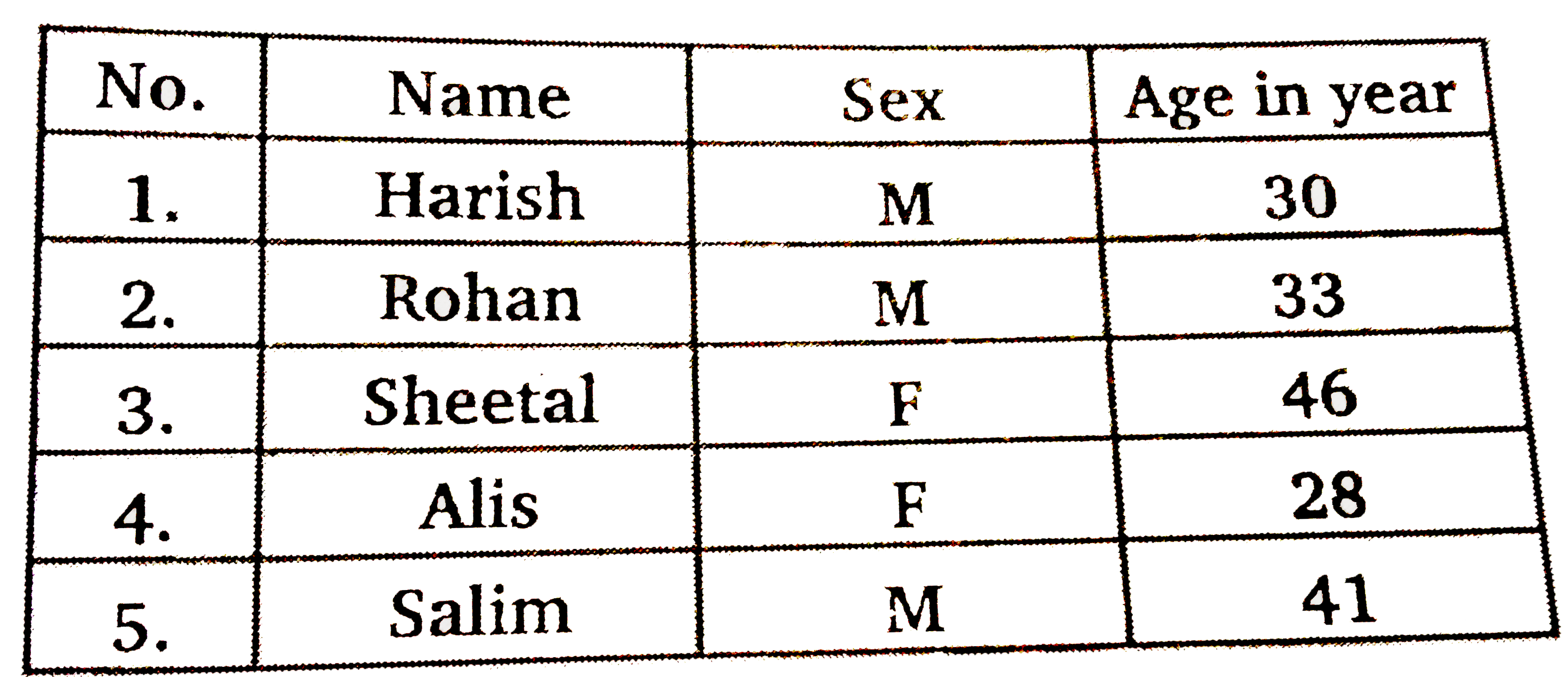
A person is selected at random from this group to act as a spokeperson. What is the probability that the spokesperson wil be either male or over 35 years?View SolutionIn how many ways can a committee of five persons be formed out of 8 members when a particular member is taken every time?
View Solution
