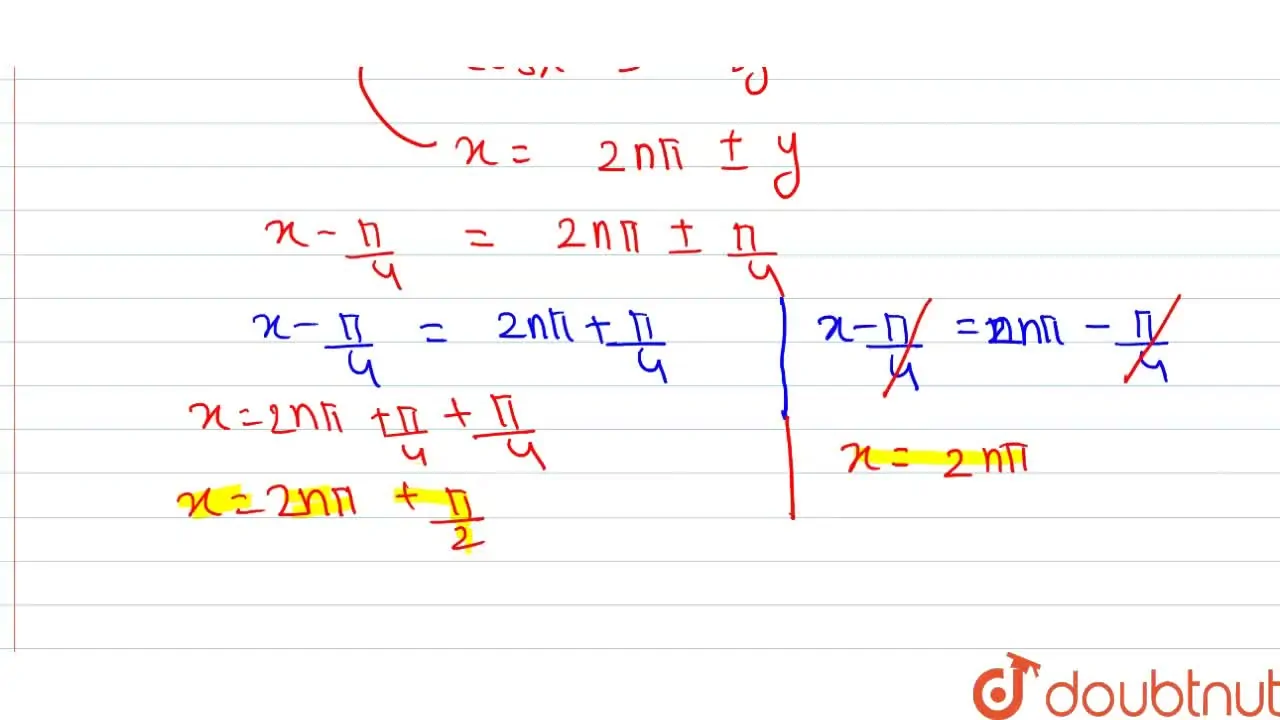
If $cosec x = 1 + cot x$ , then $x = 2 n π, 2 n π + \frac{π}{2}$

Video Solution

Text Solution

Verified by Experts

True
Given that, $cosec x = 1 + cot x$
$\Rightarrow \frac{1}{sin x} = 1 + \frac{cos x}{sin x} \Rightarrow \frac{1}{sin x} = \frac{sin x + cos x}{sin x}$
$\Rightarrow sin x + cos x = 1$
$\Rightarrow \frac{1}{\sqrt{2}} \cdot sin x + \frac{1}{\sqrt{2}} \cdot cos x = \frac{1}{\sqrt{2}}$
$\Rightarrow sin \frac{π}{4} sin x + cos x cos \frac{π}{4} = \frac{1}{\sqrt{2}}$
$\Rightarrow cos (x - \frac{π}{4}) = cos \frac{π}{4}$
$∴ x - \frac{π}{4} = 2 n π \pm \frac{π}{4}$
For positive sign, $x = 2 n π + \frac{π}{4} + \frac{π}{4} = 2 n π + \frac{π}{2}$
For negative sign, $x = 2 n π - \frac{π}{4} + \frac{π}{4} = 2 n π$

|

Updated on:21/07/2023

Class 11 MATHS TRIGONOMETRIC FUNCTIONS

Topper's Solved these Questions

Similar Questions

NCERT EXEMPLAR ENGLISH-TRIGONOMETRIC FUNCTIONS -TRUE/FALSE

Home
Ask Doubt
Profile