In a diagnostic test in mathematics given to students, the following marks (out of 100) are recorded 46,52,48,11,41,62,54,53,96,40,98 and 44.
Which average will be a good representative of the above data and why?
To determine which average (mean, median, or mode) is a good representative of the given data, we will analyze the marks recorded and evaluate the characteristics of each average.
Step 1: List the Marks
The marks recorded are:
46, 52, 48, 11, 41, 62, 54, 53, 96, 40, 98, and 44.
Step 2: Calculate the Mean
The mean is calculated by adding all the marks together and dividing by the number of marks.
1. Sum of the marks:
46+52+48+11+41+62+54+53+96+40+98+44=505
2. Number of marks:
There are 12 marks.
3. Mean:
Mean=Sum of marksNumber of marks=50512≈42.08
Step 3: Calculate the Median
The median is the middle value when the data is arranged in ascending order.
1. Arrange the marks in ascending order:
11, 40, 41, 44, 46, 48, 52, 53, 54, 62, 96, 98
2. Find the median:
Since there are 12 marks (an even number), the median will be the average of the 6th and 7th values.
Median=48+522=1002=50
Step 4: Calculate the Mode
The mode is the value that appears most frequently in the data set.
1. Check for frequency:
Each mark appears only once, so there is no mode in this data set.
Step 5: Analyze the Results
- Mean: Approximately 42.08
- Median: 50
- Mode: None
Step 6: Conclusion
The median (50) is a better representative of the data than the mean (approximately 42.08) because:
1. The mean is affected by extreme values (11 and 98), which skews the average downwards.
2. The median is not affected by extreme values and provides a better central tendency of the data.
Final Answer
The median is a good representative of the above data because it is not influenced by extreme values, and all marks are unique.
---
The marks recorded are:
46, 52, 48, 11, 41, 62, 54, 53, 96, 40, 98, and 44.
The mean is calculated by adding all the marks together and dividing by the number of marks.
46+52+48+11+41+62+54+53+96+40+98+44=505
There are 12 marks.
Mean=Sum of marksNumber of marks=50512≈42.08
The median is the middle value when the data is arranged in ascending order.
11, 40, 41, 44, 46, 48, 52, 53, 54, 62, 96, 98
Since there are 12 marks (an even number), the median will be the average of the 6th and 7th values.
Median=48+522=1002=50
The mode is the value that appears most frequently in the data set.
Each mark appears only once, so there is no mode in this data set.
- Mean: Approximately 42.08
- Median: 50
- Mode: None
The median (50) is a better representative of the data than the mean (approximately 42.08) because:
1. The mean is affected by extreme values (11 and 98), which skews the average downwards.
2. The median is not affected by extreme values and provides a better central tendency of the data.
The median is a good representative of the above data because it is not influenced by extreme values, and all marks are unique.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - SHORT ANSWER TYPE QUESTIONS20videos
STATISTICS AND PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ANSWER TYPE QUESTIONS12videos
STATISTICS AND PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - LONG ANSWER TYPE QUESTIONS12videos
STATISTICS AND PROBABILITY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - QUADRILATERALS EXERCISE - LONG ANSWER TYPE QUESTIONS18videos
QUADRILATERALS
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - LONG ANSWER TYPE QUESTIONS21videos
TRIANGLES
Similar Questions
In a mathematics test given to 15 students, the following marks (out of 100) are recorded:41, 39, 48, 52, 46, 62, 54, 40, 96, 52, 98, 40, 42, 52, 60 Find the mean, median and mode of this data
View SolutionThe marks obtained (out of 100) by a class of 80 students are given below:
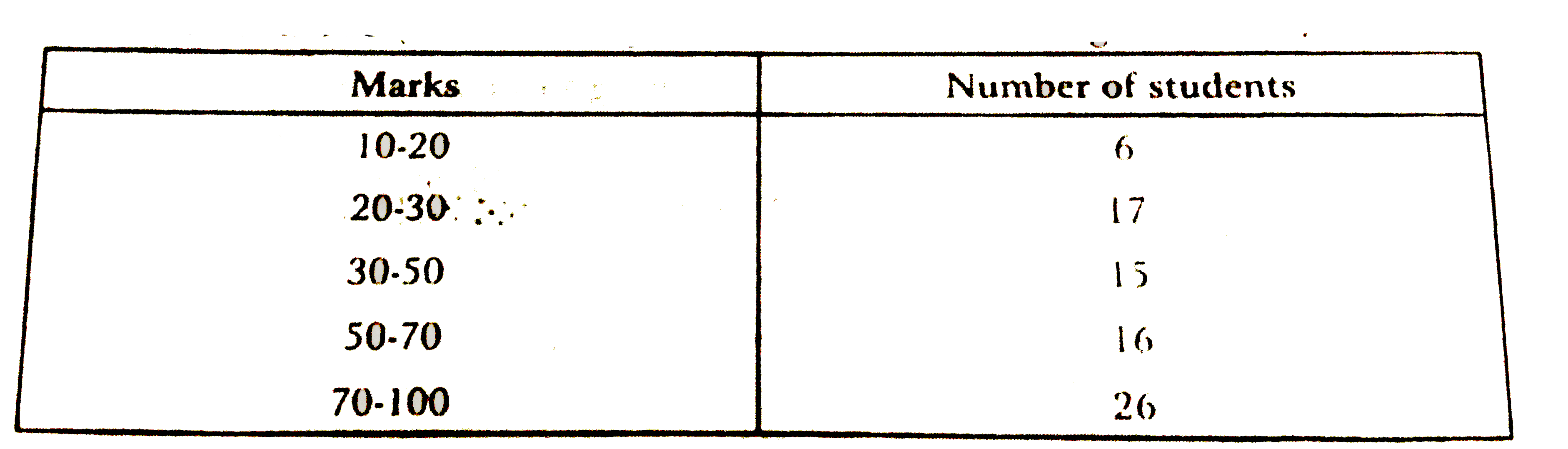
Construct a histogram to represent the data aboveView SolutionThe final marks in mathematics of 30 students are as follows:53, 61, 48, 60, 78, 68, 55, 100,67,90 75,88,77,37,84,58,60,48,62,56 44, 58, 52, 64, 98, 59, 70, 39, 50, 60 (i) Arrange these marks in the ascending order. 30 to 39 one group, 40 to 49 second group, etc. (ii) What is the highest score? (iii) What is the lowest score? (iv) What is the range?
View SolutionThe final marks in mathematics of 30 students are as follows:
53,61,48,60,78,68,55,100,67,90,75,88,77,37,84,58,60,48,62,56,44,58,52,64,98,59,70,39,50,60 Arrange these marks in the ascending order, 30 to 39 one group, 40 to 49 second group etc. Now answer the following: What is the highest score? What is the lowest score? What is the range? If 40 is the pass marks how many have failed? How many have scored 75 or more? Which observations between 50 and 60 have not actually appeared? How many have scored less than 50?View SolutionGiven below (figure) is the bar graph indicating the marks obtained out of 50 in mathematics paper by 100 students. Read the bar graph and answer the following questions: It is decided to distribute work books on mathematics to the students obtaining less then 20 marks, giving one workbook to each of such students. If a work book costs Rs.5, what sum is required to buy the work books? Every student belonging to the highest mark group is entitled to get a prize of Rs. 10. How much amount of money is required for distributing the prize money? Every student belonging to the lowest mark-group has to solve 5 problems per day. How many problems, in all, will be solved by the students of this group per day? State whether true or false. 17% students have obtained marks ranging from 40 to 49. 59 students have obtained marks ranging from 10 to 29. What is the number of students getting less than 20 marks? What is the number of students getting more than 29 marks? What is the number of students getting marks between 9 to 40? What is the number of students belonging to the highest mark group? What is the number of students obtaining more than 19 marks?
View SolutionThe income and expenditure for 5 years of a family is given in the following data: years 1995-96 1996-97 1997-98 1998-99 1999-00 Income (Rs. Thousands) 100 140 150 170 210 Expenditure (Rs. in thousands) 80 130 145 160 190 Represent the above data by a bar graph.
View SolutionThe following are the ages (in years) of 12 teachers in a school :
36, 44, 39, 46, 35, 53, 38, 42, 55, 45, 49, 40
Arrange the above data in an ascending order and answer the questions given below:
What is the mean age of the teachers in the school?View SolutionThe following are the ages (in years) of 12 teachers in a school :
36, 44, 39, 46, 35, 53, 38, 42, 55, 45, 49, 40
Arrange the above data in an ascending order and answer the questions given below:
What is the age of the youngest teacher in the school?View SolutionThe following are the ages (in years) of 12 teachers in a school :
36, 44, 39, 46, 35, 53, 38, 42, 55, 45, 49, 40
Arrange the above data in an ascending order and answer the questions given below:
What is the range of the ages of the teachers in the school?View SolutionThe following are the ages (in years) of 12 teachers in a school :
36, 44, 39, 46, 35, 53, 38, 42, 55, 45, 49, 40
Arrange the above data in an ascending order and answer the questions given below:
What is the age of the eldest teacher in the school?View Solution
