Write True or False and justify your answer in each of the following:
The volume of the largest right circular cone that can be fitted in a cube whose edge is 2r equals to the volume of a hemisphere radius r.
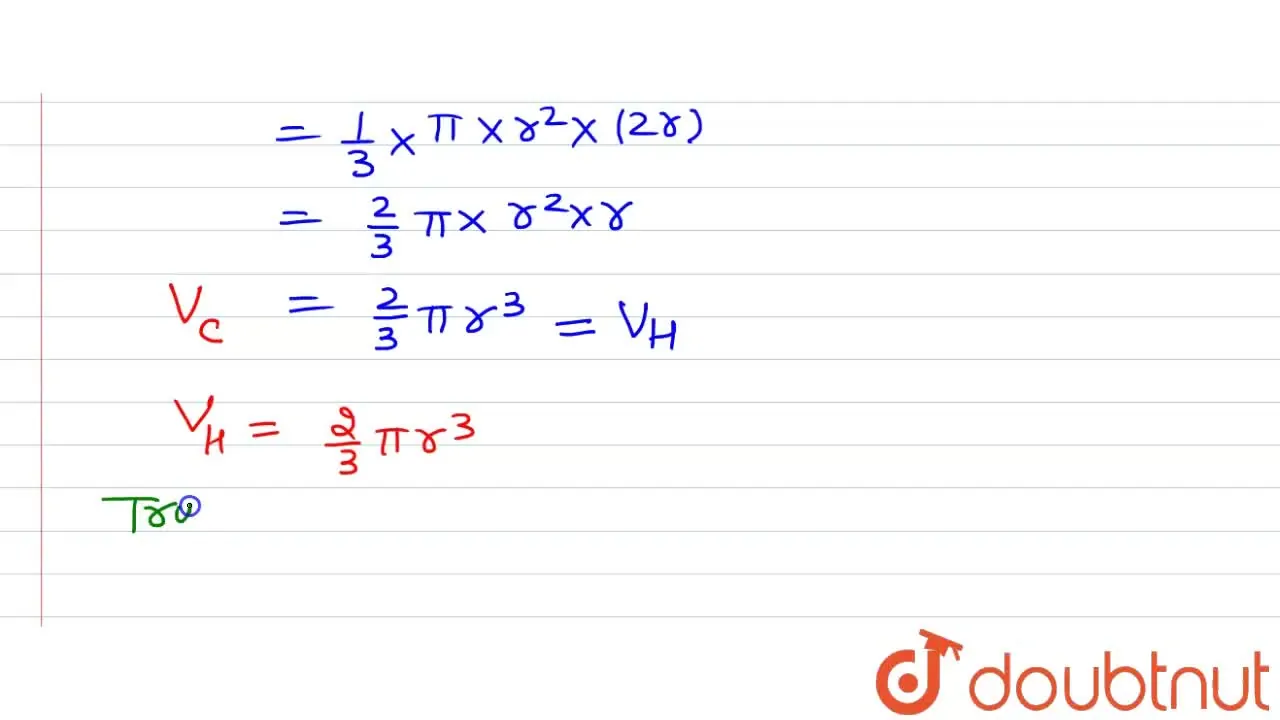
True
Given, edge of cube = 2r, then height of cube becomes h = 2r.
Volume of a cone =13πr2h=13πr2(2r)=23πr3
Volume of a hemisphere =23πr3
Hence, the volume of a cone is equal to the volume of the volume of a hemisphere.
Related Playlists
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - VOLUME AND SURFACE AREAEXERCISE - SHORT ANSWER TYPW QUESTIONS10videos
VOLUME AND SURFACE AREA
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - VOLUME AND SURFACE AREAEXERCISE - LONG ANSWER TYPE QUESTIONS8videos
VOLUME AND SURFACE AREA
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - VOLUME AND SURFACE AREAEXERCISE - LONG ANSWER TYPE QUESTIONS8videos
VOLUME AND SURFACE AREA
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - LONG ANSWER TYPE QUESTIONS21videos
TRIANGLES
Similar Questions
Find the volume of the largest right circular cone that can be fitted in a cube whose edge is 14cm.
View SolutionFind the volume of the largest right circular cone that can be cut out of a cube whose edge is 9cm.
View SolutionFind the volume of the largest right circular cone that can be cut out of a cube whose edge is 9cm.
View SolutionFind the volume of the larges right circular cone that can be cut out of a cube whose edge is 9cm.
View SolutionThe radius (in cm) of the largest right circular cone that can be cut out from a cube of edge 4.2 cm is
View SolutionFind the volume of the largest cylinder that can be inscribed in a sphere of radius r
View SolutionFind the volume of that largest cone that can be cut from a cube of edge 8 cm.
View SolutionWrite True or False and justify your answer in each of the following:
If the radius of a cylinder is doubled and its curved surface area is not changed, the height must be halved.View SolutionFind the volume of that largest cone that can be cut from a cube of edge 14 cm.
View SolutionWrite True or False and justify your answer:
The area of the isosceles triangle is 54√11 cm2 if the perimeter is 11 cm and the base is 5 cm.View Solution