The area of the equilateral triangle is 20√3cm2 whose each side is 8 cm.
To determine whether the statement "The area of the equilateral triangle is 20√3cm2 whose each side is 8cm" is true or false, we will calculate the area of the equilateral triangle using the formula for its area.
1. Identify the formula for the area of an equilateral triangle:
The formula for the area A of an equilateral triangle with side length a is given by:
A=√34a2
2. Substitute the side length into the formula:
Given that the side length a=8cm, we substitute this value into the formula:
A=√34×(8)2
3. Calculate 82:
82=64
So, we can rewrite the area as:
A=√34×64
4. Simplify the expression:
Now, we simplify 644:
644=16
Therefore, the area becomes:
A=16√3cm2
5. Compare the calculated area with the given area:
The calculated area 16√3cm2 is not equal to the given area 20√3cm2.
6. Conclusion:
Since 16√3cm2 is not equal to 20√3cm2, we conclude that the statement is false.
Final Answer:
The statement is false because the area of the equilateral triangle with each side of 8cm is 16√3cm2, not 20√3cm2.
The formula for the area A of an equilateral triangle with side length a is given by:
A=√34a2
Given that the side length a=8cm, we substitute this value into the formula:
A=√34×(8)2
82=64
So, we can rewrite the area as:
A=√34×64
Now, we simplify 644:
644=16
Therefore, the area becomes:
A=16√3cm2
The calculated area 16√3cm2 is not equal to the given area 20√3cm2.
Since 16√3cm2 is not equal to 20√3cm2, we conclude that the statement is false.
The statement is false because the area of the equilateral triangle with each side of 8cm is 16√3cm2, not 20√3cm2.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - HERON'S FORMULAEXERCISE - Short Answer Type Questions10videos
HERON'S FORMULA
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - HERON'S FORMULAEXERCISE - Long Answer Type Questions8videos
HERON'S FORMULA
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - HERON'S FORMULAEXERCISE - Long Answer Type Questions8videos
HERON'S FORMULA
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - COORDINATE GEOMETRYEXERCISE - Exercise 3.4 Long Answer Type Questions5videos
COORDINATE GEOMETRY
- BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - INTRODUCTION TO EUCLID GEOMETRYEXERCISE - Exercise 5.4 Long Answer Type Questions5videos
INTRODUCTION TO EUCLID GEOMETRY
Similar Questions
The area of an equilateral triangle with side 2√3 cm is
View SolutionThe area of an equilateral triangle is 144√3cm2, find its perimeter.
View SolutionThe area of an equilateral triangle is 36√3 sq. cm. Find its perimeter.
View SolutionIf the area of an equilateral triangle is 16√3cm2, then the perimeter of the triangle is
View SolutionIf area of an equilateral triangle is 3√3 cm2, then its height is (a)3 cm (b) √3 cm (c)6 cm (d) 2√3 cm
View SolutionThe area of an equilateral triangle is 4√3 cm2. The length of each of its side is
(a)3 cm
(b) 4 cm
(c)2√3 cm
(d) √32 cmView SolutionThe given figure shows an equilateral triangle ABC whose each side is 10 cm and a right-angled triangle BDC whose side BD = 8 cm and ∠D=90∘. Find the area of the shaded portion.
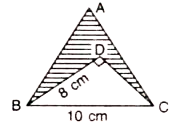 View Solution
View SolutionFind the area and the height of an equilateral triangle whose each side measures :
6.4 m (Take √3=1.73 in each case )View SolutionFind the area and the height of an equilateral triangle whose each side measures :
12 cmView SolutionProve that the area of an equilateral triangle is equal to √34a2, where a is the side of the triangle.
View Solution
